how to solve questions on elasticity in physics

• Science Notes Posts
• Contact Science Notes
• Todd Helmenstine Biography
• Anne Helmenstine Biography
• Free Printable Periodic Tables (PDF and PNG)
• Periodic Table Wallpapers
• Interactive Periodic Table
• Periodic Table Posters
• How to Grow Crystals
• Chemistry Projects
• Fire and Flames Projects
• Holiday Science
• Chemistry Problems With Answers
• Physics Problems
• Unit Conversion Example Problems
• Chemistry Worksheets
• Biology Worksheets
• Periodic Table Worksheets
• Physical Science Worksheets
• Science Lab Worksheets
• My Amazon Books

Elastic Collision Example Problem – Physics Example Problems

Elastic collisions are collisions between objects where both momentum and kinetic energy are conserved. This elastic collision example problem will show how to find the final velocities of two bodies after an elastic collision.

This illustration shows a generic elastic collision between two masses A and B. The variables involved are

m A is the mass of the object A V Ai is the initial velocity of the object A V Af is the final velocity of the object A m B is the mass of the object B V Bi is the initial velocity of the object B and V Bf is the final velocity of the object B.

If the initial conditions are known, the total momentum of the system can be expressed as

total momentum before collision = total momentum after collision

m A V Ai + m B V Bi = m A V Af + m B V Bf

The kinetic energy of the system is

kinetic energy before collision = kinetic energy after collection

½m A V Ai 2 + ½m B V Bi 2 = ½m A V Af 2 + ½m B V Bf 2

These two equations can be solved for the final velocities as

If you’d like to see how to get to these equations, see Elastic Collision of Two Masses – It Can Be Shown Exercise for a step by step solution.

Elastic Collision Example Problem

A 10 kg mass traveling 2 m/s meets and collides elastically with a 2 kg mass traveling 4 m/s in the opposite direction. Find the final velocities of both objects.

First, visualize the problem. This illustration shows what we know of the conditions.

The second step is to set your reference. Velocity is a vector quantity and we need to distinguish the direction of the velocity vectors. I’m going to choose from left to right as the “positive” direction. Any velocity moving from right to left will then contain a negative value.

Next, identify the known variables. We know the following:

m A = 10 kg V Ai 2 m/s m B = 2 kg V Bi = -4 m/s. The negative sign is because the velocity is in the negative direction.

Now we need to find V Af and V Bf . Use the equations from above. Let’s start with V Af .

Plug in our known values.

V Af = 0 m/s

The final velocity of the larger mass is zero. The collision completely stopped this mass.

Now for V Bf

Plug in our known values

V Bf = 6 m/s

The second, smaller mass shoots off to the right (positive sign on the answer) at 6 m/s while the first, larger mass is stopped dead in space by the elastic collision.

Note: If you chose your frame of reference in the opposite direction in the second step, your final answer will be V Af = 0 m/s and V Bf = -6 m/s. The collision does not change, only the signs on your answers. Make sure the velocity values you use in your formulas match your frame of reference.

Related Posts

12.4 Elasticity and Plasticity

Learning objectives.

By the end of this section, you will be able to:

• Explain the limit where a deformation of material is elastic
• Describe the range where materials show plastic behavior
• Analyze elasticity and plasticity on a stress-strain diagram

We referred to the proportionality constant between stress and strain as the elastic modulus. But why do we call it that? What does it mean for an object to be elastic and how do we describe its behavior?

Elasticity is the tendency of solid objects and materials to return to their original shape after the external forces (load) causing a deformation are removed. An object is elastic when it comes back to its original size and shape when the load is no longer present. Physical reasons for elastic behavior vary among materials and depend on the microscopic structure of the material. For example, the elasticity of polymers and rubbers is caused by stretching polymer chains under an applied force. In contrast, the elasticity of metals is caused by resizing and reshaping the crystalline cells of the lattices (which are the material structures of metals) under the action of externally applied forces.

The two parameters that determine the elasticity of a material are its elastic modulus and its elastic limit . A high elastic modulus is typical for materials that are hard to deform; in other words, materials that require a high load to achieve a significant strain. An example is a steel band. A low elastic modulus is typical for materials that are easily deformed under a load; for example, a rubber band. If the stress under a load becomes too high, then when the load is removed, the material no longer comes back to its original shape and size, but relaxes to a different shape and size: The material becomes permanently deformed. The elastic limit is the stress value beyond which the material no longer behaves elastically but becomes permanently deformed.

Our perception of an elastic material depends on both its elastic limit and its elastic modulus. For example, all rubbers are characterized by a low elastic modulus and a high elastic limit; hence, it is easy to stretch them and the stretch is noticeably large. Among materials with identical elastic limits, the most elastic is the one with the lowest elastic modulus.

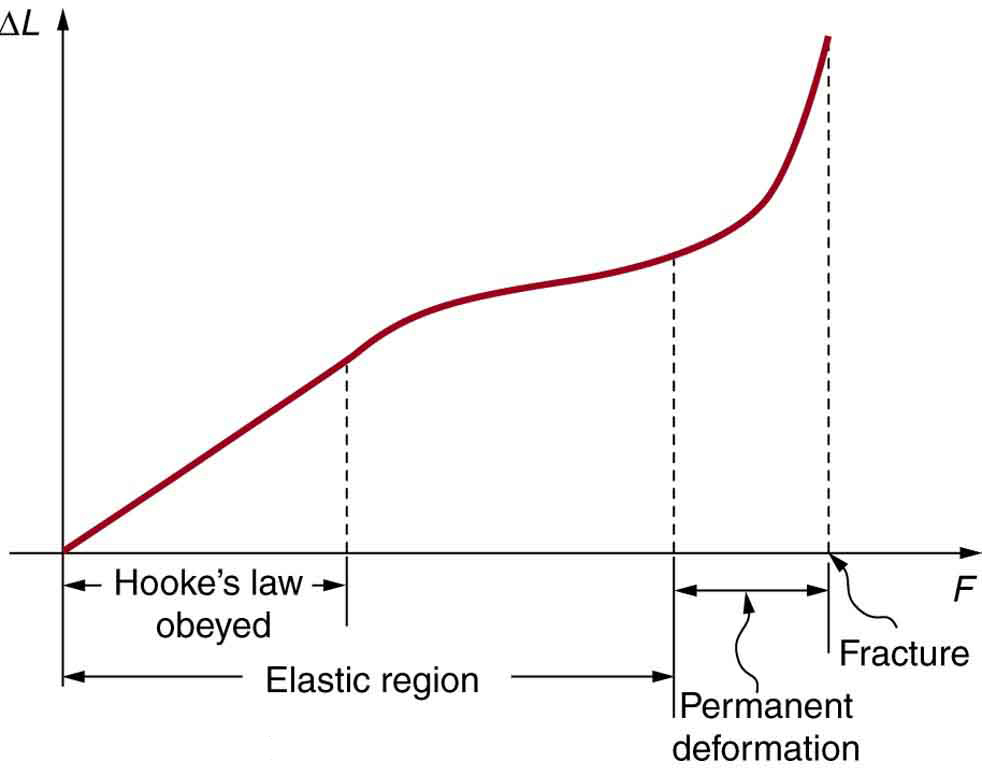
When the load increases from zero, the resulting stress is in direct proportion to strain in the way given by Equation 12.33 , but only when stress does not exceed some limiting value. For stress values within this linear limit, we can describe elastic behavior in analogy with Hooke’s law for a spring. According to Hooke’s law, the stretch value of a spring under an applied force is directly proportional to the magnitude of the force. Conversely, the response force from the spring to an applied stretch is directly proportional to the stretch. In the same way, the deformation of a material under a load is directly proportional to the load, and, conversely, the resulting stress is directly proportional to strain. The linearity limit (or the proportionality limit ) is the largest stress value beyond which stress is no longer proportional to strain. Beyond the linearity limit, the relation between stress and strain is no longer linear. When stress becomes larger than the linearity limit but still within the elasticity limit, behavior is still elastic, but the relation between stress and strain becomes nonlinear.

For stresses beyond the elastic limit, a material exhibits plastic behavior . This means the material deforms irreversibly and does not return to its original shape and size, even when the load is removed. When stress is gradually increased beyond the elastic limit, the material undergoes plastic deformation. Rubber-like materials show an increase in stress with the increasing strain, which means they become more difficult to stretch and, eventually, they reach a fracture point where they break. Ductile materials such as metals show a gradual decrease in stress with the increasing strain, which means they become easier to deform as stress-strain values approach the breaking point. Microscopic mechanisms responsible for plasticity of materials are different for different materials.

We can graph the relationship between stress and strain on a stress-strain diagram . Each material has its own characteristic strain-stress curve. A typical stress-strain diagram for a ductile metal under a load is shown in Figure 12.25 . In this figure, strain is a fractional elongation (not drawn to scale). When the load is gradually increased, the linear behavior (red line) that starts at the no-load point (the origin) ends at the linearity limit at point H . For further load increases beyond point H , the stress-strain relation is nonlinear but still elastic. In the figure, this nonlinear region is seen between points H and E . Ever larger loads take the stress to the elasticity limit E , where elastic behavior ends and plastic deformation begins. Beyond the elasticity limit, when the load is removed, for example at P , the material relaxes to a new shape and size along the green line. This is to say that the material becomes permanently deformed and does not come back to its initial shape and size when stress becomes zero.

The material undergoes plastic deformation for loads large enough to cause stress to go beyond the elasticity limit at E . The material continues to be plastically deformed until the stress reaches the fracture point (breaking point). Beyond the fracture point, we no longer have one sample of material, so the diagram ends at the fracture point. For the completeness of this qualitative description, it should be said that the linear, elastic, and plasticity limits denote a range of values rather than one sharp point.

The value of stress at the fracture point is called breaking stress (or ultimate stress ). Materials with similar elastic properties, such as two metals, may have very different breaking stresses. For example, ultimate stress for aluminum is 2.2 × 10 8 Pa 2.2 × 10 8 Pa and for steel it may be as high as 20.0 × 10 8 Pa , 20.0 × 10 8 Pa , depending on the kind of steel. We can make a quick estimate, based on Equation 12.34 , that for rods with a 1 -in 2 1 -in 2 cross-sectional area, the breaking load for an aluminum rod is 3.2 × 10 4 lb , 3.2 × 10 4 lb , and the breaking load for a steel rod is about nine times larger.

As an Amazon Associate we earn from qualifying purchases.

This book may not be used in the training of large language models or otherwise be ingested into large language models or generative AI offerings without OpenStax's permission.

Want to cite, share, or modify this book? This book uses the Creative Commons Attribution License and you must attribute OpenStax.

Access for free at https://openstax.org/books/university-physics-volume-1/pages/1-introduction

• Authors: William Moebs, Samuel J. Ling, Jeff Sanny
• Publisher/website: OpenStax
• Book title: University Physics Volume 1
• Publication date: Sep 19, 2016
• Location: Houston, Texas
• Book URL: https://openstax.org/books/university-physics-volume-1/pages/1-introduction
• Section URL: https://openstax.org/books/university-physics-volume-1/pages/12-4-elasticity-and-plasticity

© Jan 19, 2024 OpenStax. Textbook content produced by OpenStax is licensed under a Creative Commons Attribution License . The OpenStax name, OpenStax logo, OpenStax book covers, OpenStax CNX name, and OpenStax CNX logo are not subject to the Creative Commons license and may not be reproduced without the prior and express written consent of Rice University.

Want to create or adapt books like this? Learn more about how Pressbooks supports open publishing practices.

5 Further Applications of Newton’s Laws: Friction, Drag, and Elasticity

33 5.3 Elasticity: Stress and Strain

• State Hooke’s law.
• Explain Hooke’s law using graphical representation between deformation and applied force.
• Discuss the three types of deformations such as changes in length, sideways shear and changes in volume.
• Describe with examples the young’s modulus, shear modulus and bulk modulus.
• Determine the change in length given mass, length and radius.

We now move from consideration of forces that affect the motion of an object (such as friction and drag) to those that affect an object’s shape. If a bulldozer pushes a car into a wall, the car will not move but it will noticeably change shape. A change in shape due to the application of a force is a deformation . Even very small forces are known to cause some deformation. For small deformations, two important characteristics are observed. First, the object returns to its original shape when the force is removed—that is, the deformation is elastic for small deformations. Second, the size of the deformation is proportional to the force—that is, for small deformations, Hooke’s law is obeyed. In equation form, Hooke’s law is given by

where[latex]\boldsymbol{\Delta{L}}[/latex]is the amount of deformation (the change in length, for example) produced by the force[latex]\boldsymbol{F},[/latex]and[latex]\boldsymbol{k}[/latex]is a proportionality constant that depends on the shape and composition of the object and the direction of the force. Note that this force is a function of the deformation[latex]\boldsymbol{\Delta{L}}[/latex]—it is not constant as a kinetic friction force is. Rearranging this to

makes it clear that the deformation is proportional to the applied force. Figure 1 shows the Hooke’s law relationship between the extension[latex]\boldsymbol{\Delta{L}}[/latex]of a spring or of a human bone. For metals or springs, the straight line region in which Hooke’s law pertains is much larger. Bones are brittle and the elastic region is small and the fracture abrupt. Eventually a large enough stress to the material will cause it to break or fracture. Tensile strength is the breaking stress that will cause permanent deformation or fracture of a material.

HOOKE’S LAW

where[latex]\boldsymbol{\Delta{L}}[/latex]is the amount of deformation (the change in length, for example) produced by the force[latex]\boldsymbol{F},[/latex]and[latex]\boldsymbol{k}[/latex]is a proportionality constant that depends on the shape and composition of the object and the direction of the force.

The proportionality constant[latex]\boldsymbol{k}[/latex]depends upon a number of factors for the material. For example, a guitar string made of nylon stretches when it is tightened, and the elongation[latex]\boldsymbol{\Delta{L}}[/latex]is proportional to the force applied (at least for small deformations). Thicker nylon strings and ones made of steel stretch less for the same applied force, implying they have a larger[latex]\boldsymbol{k}[/latex](see Figure 2 ). Finally, all three strings return to their normal lengths when the force is removed, provided the deformation is small. Most materials will behave in this manner if the deformation is less than about 0.1% or about 1 part in[latex]\boldsymbol{10^3}.[/latex]

STRETCH YOURSELF A LITTLE

How would you go about measuring the proportionality constant[latex]\boldsymbol{k}[/latex]of a rubber band? If a rubber band stretched 3 cm when a 100-g mass was attached to it, then how much would it stretch if two similar rubber bands were attached to the same mass—even if put together in parallel or alternatively if tied together in series?

We now consider three specific types of deformations: changes in length (tension and compression), sideways shear (stress), and changes in volume. All deformations are assumed to be small unless otherwise stated.

Changes in Length—Tension and Compression: Elastic Modulus

A change in length[latex]\boldsymbol{\Delta{L}}[/latex]is produced when a force is applied to a wire or rod parallel to its length[latex]\boldsymbol{L_0},[/latex]either stretching it (a tension) or compressing it. (See Figure 3 .)

Experiments have shown that the change in length ([latex]\boldsymbol{\Delta{L}}[/latex]) depends on only a few variables. As already noted,[latex]\boldsymbol{\Delta{L}}[/latex]is proportional to the force[latex]\boldsymbol{F}[/latex]and depends on the substance from which the object is made. Additionally, the change in length is proportional to the original length[latex]\boldsymbol{L_0}[/latex]and inversely proportional to the cross-sectional area of the wire or rod. For example, a long guitar string will stretch more than a short one, and a thick string will stretch less than a thin one. We can combine all these factors into one equation for[latex]\boldsymbol{\Delta{L}}:[/latex]

where[latex]\boldsymbol{\Delta{L}}[/latex]is the change in length,[latex]\boldsymbol{F}[/latex]the applied force,[latex]\boldsymbol{Y}[/latex]is a factor, called the elastic modulus or Young’s modulus, that depends on the substance,[latex]\boldsymbol{A}[/latex]is the cross-sectional area, and[latex]\boldsymbol{L_0}[/latex]is the original length. Table 3 lists values of[latex]\boldsymbol{Y}[/latex]for several materials—those with a large[latex]\boldsymbol{Y}[/latex]are said to have a large tensile stiffness because they deform less for a given tension or compression.

Young’s moduli are not listed for liquids and gases in Table 3 because they cannot be stretched or compressed in only one direction. Note that there is an assumption that the object does not accelerate, so that there are actually two applied forces of magnitude[latex]\boldsymbol{F}[/latex]acting in opposite directions. For example, the strings in Figure 3 are being pulled down by a force of magnitude[latex]\boldsymbol{w}[/latex]and held up by the ceiling, which also exerts a force of magnitude[latex]\boldsymbol{w}.[/latex]

Example 1: The Stretch of a Long Cable

Suspension cables are used to carry gondolas at ski resorts. (See Figure 4 ) Consider a suspension cable that includes an unsupported span of 3 km. Calculate the amount of stretch in the steel cable. Assume that the cable has a diameter of 5.6 cm and the maximum tension it can withstand is[latex]\boldsymbol{3.0\times10^6\textbf{ N}}.[/latex]

The force is equal to the maximum tension, or[latex]\boldsymbol{F=3.0\times10^6\textbf{ N}}.[/latex]The cross-sectional area is[latex]\boldsymbol{\pi{r}^2=2.46\times10^{-3}\textbf{ m}^2}.[/latex]The equation[latex]\boldsymbol{\Delta{L}=\frac{1}{Y}\frac{F}{A}L_0}[/latex]can be used to find the change in length.

All quantities are known. Thus,

This is quite a stretch, but only about 0.6% of the unsupported length. Effects of temperature upon length might be important in these environments.

Bones, on the whole, do not fracture due to tension or compression. Rather they generally fracture due to sideways impact or bending, resulting in the bone shearing or snapping. The behavior of bones under tension and compression is important because it determines the load the bones can carry. Bones are classified as weight-bearing structures such as columns in buildings and trees. Weight-bearing structures have special features; columns in building have steel-reinforcing rods while trees and bones are fibrous. The bones in different parts of the body serve different structural functions and are prone to different stresses. Thus the bone in the top of the femur is arranged in thin sheets separated by marrow while in other places the bones can be cylindrical and filled with marrow or just solid. Overweight people have a tendency toward bone damage due to sustained compressions in bone joints and tendons.

Another biological example of Hooke’s law occurs in tendons. Functionally, the tendon (the tissue connecting muscle to bone) must stretch easily at first when a force is applied, but offer a much greater restoring force for a greater strain. Figure 5 shows a stress-strain relationship for a human tendon. Some tendons have a high collagen content so there is relatively little strain, or length change; others, like support tendons (as in the leg) can change length up to 10%. Note that this stress-strain curve is nonlinear, since the slope of the line changes in different regions. In the first part of the stretch called the toe region, the fibers in the tendon begin to align in the direction of the stress—this is called uncrimping . In the linear region, the fibrils will be stretched, and in the failure region individual fibers begin to break. A simple model of this relationship can be illustrated by springs in parallel: different springs are activated at different lengths of stretch. Examples of this are given in the problems at end of this chapter. Ligaments (tissue connecting bone to bone) behave in a similar way.

Unlike bones and tendons, which need to be strong as well as elastic, the arteries and lungs need to be very stretchable. The elastic properties of the arteries are essential for blood flow. The pressure in the arteries increases and arterial walls stretch when the blood is pumped out of the heart. When the aortic valve shuts, the pressure in the arteries drops and the arterial walls relax to maintain the blood flow. When you feel your pulse, you are feeling exactly this—the elastic behavior of the arteries as the blood gushes through with each pump of the heart. If the arteries were rigid, you would not feel a pulse. The heart is also an organ with special elastic properties. The lungs expand with muscular effort when we breathe in but relax freely and elastically when we breathe out. Our skins are particularly elastic, especially for the young. A young person can go from 100 kg to 60 kg with no visible sag in their skins. The elasticity of all organs reduces with age. Gradual physiological aging through reduction in elasticity starts in the early 20s.

Example 2: Calculating Deformation: How Much Does Your Leg Shorten When You Stand on It?

Calculate the change in length of the upper leg bone (the femur) when a 70.0 kg man supports 62.0 kg of his mass on it, assuming the bone to be equivalent to a uniform rod that is 40.0 cm long and 2.00 cm in radius.

The force is equal to the weight supported, or

and the cross-sectional area is[latex]\boldsymbol{\pi{r}^2=1.257\times10^{-3}\textbf{ m}^2}.[/latex]The equation[latex]\boldsymbol{\Delta{L}=\frac{1}{Y}\frac{F}{A}L_0}[/latex]can be used to find the change in length.

All quantities except[latex]\boldsymbol{\Delta{L}}[/latex]are known. Note that the compression value for Young’s modulus for bone must be used here. Thus,

This small change in length seems reasonable, consistent with our experience that bones are rigid. In fact, even the rather large forces encountered during strenuous physical activity do not compress or bend bones by large amounts. Although bone is rigid compared with fat or muscle, several of the substances listed in Table 3 have larger values of Young’s modulus[latex]\boldsymbol{Y}.[/latex]In other words, they are more rigid.

The equation for change in length is traditionally rearranged and written in the following form:

The ratio of force to area,[latex]\boldsymbol{\frac{F}{A}},[/latex]is defined as stress (measured in[latex]\boldsymbol{\textbf{N/m}^2}[/latex]), and the ratio of the change in length to length,[latex]\boldsymbol{\frac{\Delta{L}}{L_0}},[/latex]is defined as strain (a unitless quantity). In other words,

In this form, the equation is analogous to Hooke’s law, with stress analogous to force and strain analogous to deformation. If we again rearrange this equation to the form

we see that it is the same as Hooke’s law with a proportionality constant

This general idea—that force and the deformation it causes are proportional for small deformations—applies to changes in length, sideways bending, and changes in volume.

The ratio of force to area,[latex]\boldsymbol{\frac{F}{A}},[/latex]is defined as stress measured in N/m 2 .

The ratio of the change in length to length,[latex]\boldsymbol{\frac{\Delta{L}}{L_0}},[/latex]is defined as strain (a unitless quantity). In other words,

Sideways Stress: Shear Modulus

Figure 6 illustrates what is meant by a sideways stress or a shearing force . Here the deformation is called[latex]\boldsymbol{\Delta{x}}[/latex]and it is perpendicular to[latex]\boldsymbol{L_0},[/latex]rather than parallel as with tension and compression. Shear deformation behaves similarly to tension and compression and can be described with similar equations. The expression for shear deformation is

where[latex]\boldsymbol{S}[/latex]is the shear modulus (see Table 3 ) and[latex]\boldsymbol{F}[/latex]is the force applied perpendicular to[latex]\boldsymbol{L_0}[/latex]and parallel to the cross-sectional area[latex]\boldsymbol{A}.[/latex]Again, to keep the object from accelerating, there are actually two equal and opposite forces[latex]\boldsymbol{F}[/latex]applied across opposite faces, as illustrated in Figure 6 . The equation is logical—for example, it is easier to bend a long thin pencil (small[latex]\boldsymbol{A}[/latex]) than a short thick one, and both are more easily bent than similar steel rods (large[latex]\boldsymbol{S}[/latex]).

SHEAR DEFORMATION

where[latex]\boldsymbol{S}[/latex]is the shear modulus and[latex]\boldsymbol{F}[/latex]is the force applied perpendicular to[latex]\boldsymbol{L_0}[/latex]and parallel to the cross-sectional area[latex]\boldsymbol{A}.[/latex]

Examination of the shear moduli in Table 3 reveals some telling patterns. For example, shear moduli are less than Young’s moduli for most materials. Bone is a remarkable exception. Its shear modulus is not only greater than its Young’s modulus, but it is as large as that of steel. This is why bones are so rigid.

The spinal column (consisting of 26 vertebral segments separated by discs) provides the main support for the head and upper part of the body. The spinal column has normal curvature for stability, but this curvature can be increased, leading to increased shearing forces on the lower vertebrae. Discs are better at withstanding compressional forces than shear forces. Because the spine is not vertical, the weight of the upper body exerts some of both. Pregnant women and people that are overweight (with large abdomens) need to move their shoulders back to maintain balance, thereby increasing the curvature in their spine and so increasing the shear component of the stress. An increased angle due to more curvature increases the shear forces along the plane. These higher shear forces increase the risk of back injury through ruptured discs. The lumbosacral disc (the wedge shaped disc below the last vertebrae) is particularly at risk because of its location.

The shear moduli for concrete and brick are very small; they are too highly variable to be listed. Concrete used in buildings can withstand compression, as in pillars and arches, but is very poor against shear, as might be encountered in heavily loaded floors or during earthquakes. Modern structures were made possible by the use of steel and steel-reinforced concrete. Almost by definition, liquids and gases have shear moduli near zero, because they flow in response to shearing forces.

Example 3: Calculating Force Required to Deform: That Nail Does Not Bend Much Under a Load

Find the mass of the picture hanging from a steel nail as shown in Figure 7 , given that the nail bends only[latex]\boldsymbol{1.80\:\mu\textbf{m}}.[/latex](Assume the shear modulus is known to two significant figures.)

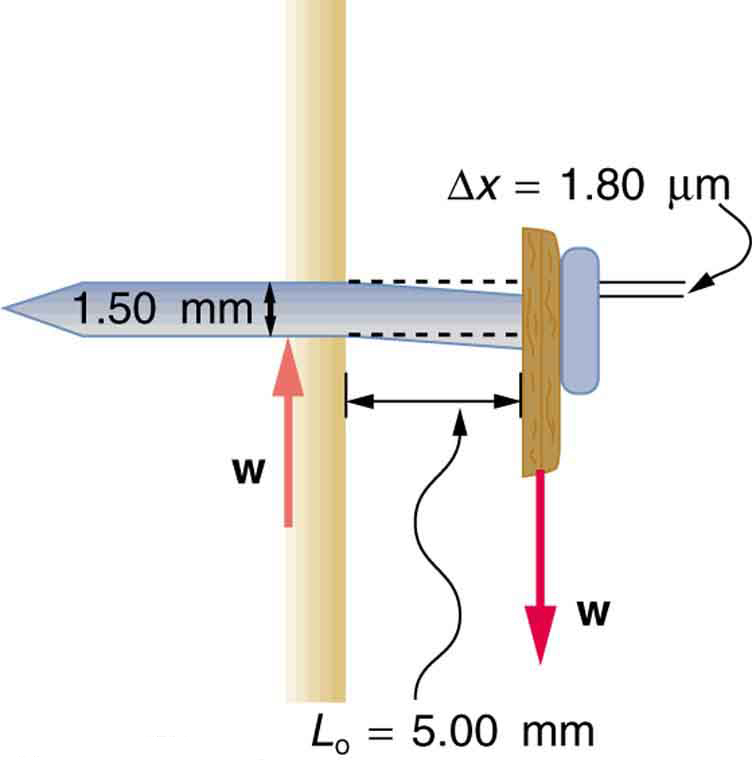
The force[latex]\boldsymbol{F}[/latex]on the nail (neglecting the nail’s own weight) is the weight of the picture[latex]\boldsymbol{w}.[/latex]If we can find[latex]\boldsymbol{w},[/latex]then the mass of the picture is just[latex]\boldsymbol{wg}.[/latex]The equation[latex]\boldsymbol{\Delta{x}=\frac{1}{S}\frac{F}{A}L_0}[/latex]can be solved for[latex]\boldsymbol{F}.[/latex]

Solving the equation[latex]\boldsymbol{\Delta{x}=\frac{1}{S}\frac{F}{A}L_0}[/latex]for[latex]\boldsymbol{F},[/latex]we see that all other quantities can be found:

S is found in Table 3 and is[latex]\boldsymbol{S=80\times10^9\textbf{ N/m}^2}.[/latex]The radius[latex]\boldsymbol{r}[/latex]is 0.750 mm (as seen in the figure), so the cross-sectional area is

The value for[latex]\boldsymbol{L_0}[/latex]is also shown in the figure. Thus,

This 51 N force is the weight[latex]\boldsymbol{w}[/latex]of the picture, so the picture’s mass is

This is a fairly massive picture, and it is impressive that the nail flexes only[latex]\boldsymbol{1.80 \:\mu\textbf{m}}[/latex]—an amount undetectable to the unaided eye.

Changes in Volume: Bulk Modulus

An object will be compressed in all directions if inward forces are applied evenly on all its surfaces as in Figure 8 . It is relatively easy to compress gases and extremely difficult to compress liquids and solids. For example, air in a wine bottle is compressed when it is corked. But if you try corking a brim-full bottle, you cannot compress the wine—some must be removed if the cork is to be inserted. The reason for these different compressibilities is that atoms and molecules are separated by large empty spaces in gases but packed close together in liquids and solids. To compress a gas, you must force its atoms and molecules closer together. To compress liquids and solids, you must actually compress their atoms and molecules, and very strong electromagnetic forces in them oppose this compression .

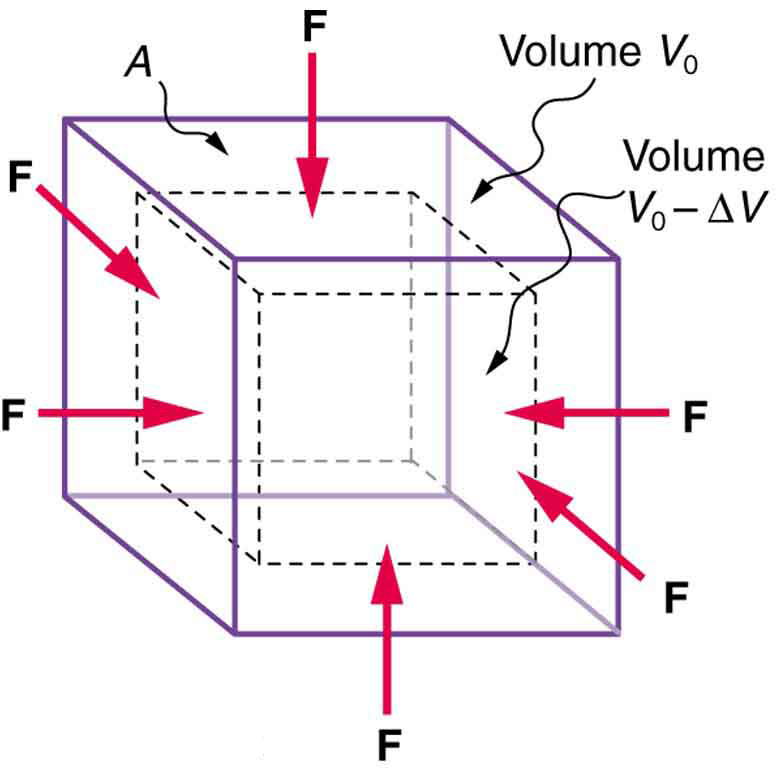
We can describe the compression or volume deformation of an object with an equation. First, we note that a force “applied evenly” is defined to have the same stress, or ratio of force to area[latex]\boldsymbol{\frac{F}{A}}[/latex]on all surfaces. The deformation produced is a change in volume[latex]\boldsymbol{\Delta{V}},[/latex]which is found to behave very similarly to the shear, tension, and compression previously discussed. (This is not surprising, since a compression of the entire object is equivalent to compressing each of its three dimensions.) The relationship of the change in volume to other physical quantities is given by

where[latex]\boldsymbol{B}[/latex]is the bulk modulus (see Table 3 ),[latex]\boldsymbol{V_0}[/latex]is the original volume, and[latex]\boldsymbol{\frac{F}{A}}[/latex]is the force per unit area applied uniformly inward on all surfaces. Note that no bulk moduli are given for gases.

What are some examples of bulk compression of solids and liquids? One practical example is the manufacture of industrial-grade diamonds by compressing carbon with an extremely large force per unit area. The carbon atoms rearrange their crystalline structure into the more tightly packed pattern of diamonds. In nature, a similar process occurs deep underground, where extremely large forces result from the weight of overlying material. Another natural source of large compressive forces is the pressure created by the weight of water, especially in deep parts of the oceans. Water exerts an inward force on all surfaces of a submerged object, and even on the water itself. At great depths, water is measurably compressed, as the following example illustrates.

Example 4: Calculating Change in Volume with Deformation: How Much Is Water Compressed at Great Ocean Depths?

Calculate the fractional decrease in volume ([latex]\boldsymbol{\frac{\Delta{V}}{V_0}}[/latex]) for seawater at 5.00 km depth, where the force per unit area is[latex]\boldsymbol{5.00\times10^7\textbf{ N/m}^2}.[/latex]

Equation[latex]\boldsymbol{\Delta{V}=\frac{1}{B}\frac{F}{A}V_0}[/latex]is the correct physical relationship. All quantities in the equation except[latex]\boldsymbol{\frac{\Delta{V}}{V_0}}[/latex]are known.

Solving for the unknown[latex]\boldsymbol{\frac{\Delta{V}}{V_0}}[/latex]gives

Substituting known values with the value for the bulk modulus[latex]\boldsymbol{B}[/latex]from Table 3 ,

Although measurable, this is not a significant decrease in volume considering that the force per unit area is about 500 atmospheres (1 million pounds per square foot). Liquids and solids are extraordinarily difficult to compress.

Conversely, very large forces are created by liquids and solids when they try to expand but are constrained from doing so—which is equivalent to compressing them to less than their normal volume. This often occurs when a contained material warms up, since most materials expand when their temperature increases. If the materials are tightly constrained, they deform or break their container. Another very common example occurs when water freezes. Water, unlike most materials, expands when it freezes, and it can easily fracture a boulder, rupture a biological cell, or crack an engine block that gets in its way.

Other types of deformations, such as torsion or twisting, behave analogously to the tension, shear, and bulk deformations considered here.

Section Summary

where[latex]\boldsymbol{\Delta{L}}[/latex]is the amount of deformation (the change in length),[latex]\boldsymbol{F}[/latex]is the applied force, and[latex]\boldsymbol{k}[/latex]is a proportionality constant that depends on the shape and composition of the object and the direction of the force. The relationship between the deformation and the applied force can also be written as

where[latex]\boldsymbol{Y}[/latex]is Young’s modulus , which depends on the substance,[latex]\boldsymbol{A}[/latex]is the cross-sectional area, and[latex]\boldsymbol{L_0}[/latex]is the original length.

• The ratio of force to area,[latex]\boldsymbol{\frac{F}{A}},[/latex]is defined as stress , measured in N/m 2 .
• The ratio of the change in length to length,[latex]\boldsymbol{\frac{\Delta{L}}{L_0}},[/latex]is defined as strain (a unitless quantity). In other words, [latex]\boldsymbol{\textbf{stress}=Y\times\textbf{strain}}.[/latex]

where[latex]\boldsymbol{B}[/latex]is the bulk modulus,[latex]\boldsymbol{V_0}[/latex]is the original volume, and[latex]\boldsymbol{\frac{F}{A}}[/latex] is the force per unit area applied uniformly inward on all surfaces.

Conceptual Questions

1: The elastic properties of the arteries are essential for blood flow. Explain the importance of this in terms of the characteristics of the flow of blood (pulsating or continuous).

2: What are you feeling when you feel your pulse? Measure your pulse rate for 10 s and for 1 min. Is there a factor of 6 difference?

3: Examine different types of shoes, including sports shoes and thongs. In terms of physics, why are the bottom surfaces designed as they are? What differences will dry and wet conditions make for these surfaces?

4: Would you expect your height to be different depending upon the time of day? Why or why not?

5: Why can a squirrel jump from a tree branch to the ground and run away undamaged, while a human could break a bone in such a fall?

6: Explain why pregnant women often suffer from back strain late in their pregnancy.

7: An old carpenter’s trick to keep nails from bending when they are pounded into hard materials is to grip the center of the nail firmly with pliers. Why does this help?

8: When a glass bottle full of vinegar warms up, both the vinegar and the glass expand, but vinegar expands significantly more with temperature than glass. The bottle will break if it was filled to its tightly capped lid. Explain why, and also explain how a pocket of air above the vinegar would prevent the break. (This is the function of the air above liquids in glass containers.)

Problems & Exercises

1: During a circus act, one performer swings upside down hanging from a trapeze holding another, also upside-down, performer by the legs. If the upward force on the lower performer is three times her weight, how much do the bones (the femurs) in her upper legs stretch? You may assume each is equivalent to a uniform rod 35.0 cm long and 1.80 cm in radius. Her mass is 60.0 kg.

2: During a wrestling match, a 150 kg wrestler briefly stands on one hand during a maneuver designed to perplex his already moribund adversary. By how much does the upper arm bone shorten in length? The bone can be represented by a uniform rod 38.0 cm in length and 2.10 cm in radius.

3: (a) The “lead” in pencils is a graphite composition with a Young’s modulus of about[latex]\boldsymbol{1\times10^9\textbf{ N/m}^2}.[/latex]Calculate the change in length of the lead in an automatic pencil if you tap it straight into the pencil with a force of 4.0 N. The lead is 0.50 mm in diameter and 60 mm long. (b) Is the answer reasonable? That is, does it seem to be consistent with what you have observed when using pencils?

4: TV broadcast antennas are the tallest artificial structures on Earth. In 1987, a 72.0-kg physicist placed himself and 400 kg of equipment at the top of one 610-m high antenna to perform gravity experiments. By how much was the antenna compressed, if we consider it to be equivalent to a steel cylinder 0.150 m in radius?

5: (a) By how much does a 65.0-kg mountain climber stretch her 0.800-cm diameter nylon rope when she hangs 35.0 m below a rock outcropping? (b) Does the answer seem to be consistent with what you have observed for nylon ropes? Would it make sense if the rope were actually a bungee cord?

6: A 20.0-m tall hollow aluminum flagpole is equivalent in stiffness to a solid cylinder 4.00 cm in diameter. A strong wind bends the pole much as a horizontal force of 900 N exerted at the top would. How far to the side does the top of the pole flex?

7: As an oil well is drilled, each new section of drill pipe supports its own weight and that of the pipe and drill bit beneath it. Calculate the stretch in a new 6.00 m length of steel pipe that supports 3.00 km of pipe having a mass of 20.0 kg/m and a 100-kg drill bit. The pipe is equivalent in stiffness to a solid cylinder 5.00 cm in diameter.

8: Calculate the force a piano tuner applies to stretch a steel piano wire 8.00 mm, if the wire is originally 0.850 mm in diameter and 1.35 m long.

9: A vertebra is subjected to a shearing force of 500 N. Find the shear deformation, taking the vertebra to be a cylinder 3.00 cm high and 4.00 cm in diameter.

10: A disk between vertebrae in the spine is subjected to a shearing force of 600 N. Find its shear deformation, taking it to have the shear modulus of[latex]\boldsymbol{1\times10^9\textbf{ N/m}^2}.[/latex]The disk is equivalent to a solid cylinder 0.700 cm high and 4.00 cm in diameter.

11: When using a pencil eraser, you exert a vertical force of 6.00 N at a distance of 2.00 cm from the hardwood-eraser joint. The pencil is 6.00 mm in diameter and is held at an angle of[latex]\boldsymbol{20.0^0}[/latex]to the horizontal. (a) By how much does the wood flex perpendicular to its length? (b) How much is it compressed lengthwise?

12: To consider the effect of wires hung on poles, we take data from Chapter 4.7 Example 2 , in which tensions in wires supporting a traffic light were calculated. The left wire made an angle[latex]\boldsymbol{30.0^0}[/latex]below the horizontal with the top of its pole and carried a tension of 108 N. The 12.0 m tall hollow aluminum pole is equivalent in stiffness to a 4.50 cm diameter solid cylinder. (a) How far is it bent to the side? (b) By how much is it compressed?

13: A farmer making grape juice fills a glass bottle to the brim and caps it tightly. The juice expands more than the glass when it warms up, in such a way that the volume increases by 0.2% (that is,[latex]\boldsymbol{\Delta{V}/V_0=2\times10^{-3}}[/latex]) relative to the space available. Calculate the magnitude of the normal force exerted by the juice per square centimeter if its bulk modulus is[latex]\boldsymbol{1.8\times10^9\textbf{ N/m}^2},[/latex]assuming the bottle does not break. In view of your answer, do you think the bottle will survive?

14: (a) When water freezes, its volume increases by 9.05% (that is,[latex]\boldsymbol{\Delta{V}/V_0=9.05\times10^{-2}}[/latex]). What force per unit area is water capable of exerting on a container when it freezes? (It is acceptable to use the bulk modulus of water in this problem.) (b) Is it surprising that such forces can fracture engine blocks, boulders, and the like?

15: This problem returns to the tightrope walker studied in Chapter 4.5 Example 2 , who created a tension of[latex]\boldsymbol{3.94\times10^3\textbf{ N}}[/latex]in a wire making an angle[latex]\boldsymbol{5.0^0}[/latex]below the horizontal with each supporting pole. Calculate how much this tension stretches the steel wire if it was originally 15 m long and 0.50 cm in diameter.

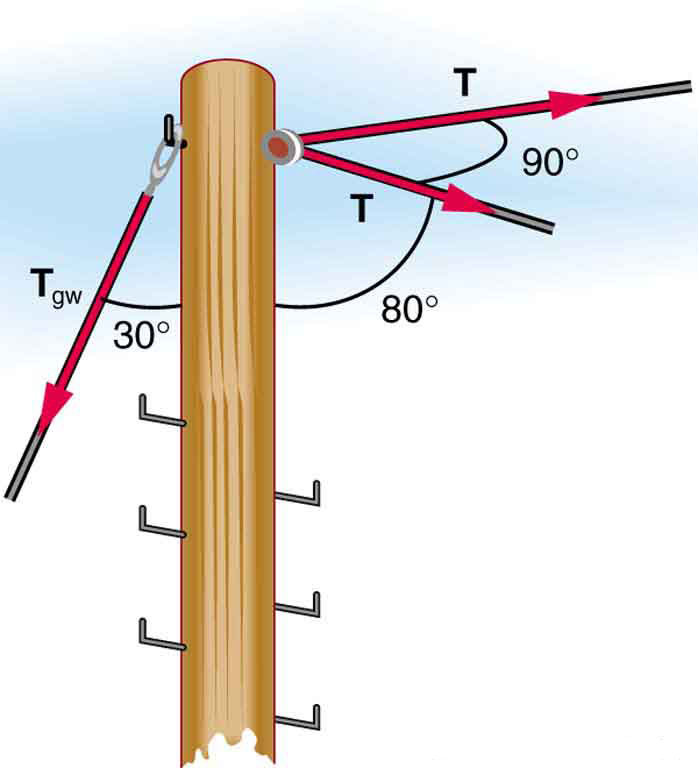
16: The pole in Figure 9 is at a[latex]\boldsymbol{90.0^0}[/latex]bend in a power line and is therefore subjected to more shear force than poles in straight parts of the line. The tension in each line is[latex]\boldsymbol{4.00\times10^4\textbf{ N}},[/latex]at the angles shown. The pole is 15.0 m tall, has an 18.0 cm diameter, and can be considered to have half the stiffness of hardwood. (a) Calculate the compression of the pole. (b) Find how much it bends and in what direction. (c) Find the tension in a guy wire used to keep the pole straight if it is attached to the top of the pole at an angle of[latex]\boldsymbol{30.0^0}[/latex]with the vertical. (Clearly, the guy wire must be in the opposite direction of the bend.)

• 1 Approximate and average values. Young’s moduli[latex]\boldsymbol{Y}[/latex]for tension and compression sometimes differ but are averaged here. Bone has significantly different Young’s moduli for tension and compression.

[latex]\boldsymbol{1.90\times10^{-3}\textbf{ cm}}[/latex]

[latex]\boldsymbol{1.49\times10^{-7}\textbf{ m}}[/latex]

(a)[latex]\boldsymbol{3.99\times10^{-7}\textbf{ m}}[/latex]

(b)[latex]\boldsymbol{9.67\times10^{-8}\textbf{ m}}[/latex]

[latex]\boldsymbol{4\times10^6\textbf{ N/m}^2}.[/latex]This is about 36 atm, greater than a typical jar can withstand.

College Physics chapters 1-17 Copyright © August 22, 2016 by OpenStax is licensed under a Creative Commons Attribution 4.0 International License , except where otherwise noted.

Share This Book

If you're seeing this message, it means we're having trouble loading external resources on our website.

If you're behind a web filter, please make sure that the domains *.kastatic.org and *.kasandbox.org are unblocked.

To log in and use all the features of Khan Academy, please enable JavaScript in your browser.

Physics library

Course: physics library   >   unit 5.

• Intro to springs and Hooke's law

What is Hooke's Law?

• Potential energy stored in a spring
• What is elastic potential energy?
• Spring potential energy example (mistake in math)
• LOL diagrams
• Vertical springs and energy conservation

What is a spring?

What happens when a material is deformed.

• Elastic deformation . When the stress is removed the material returns to the dimension it had before the load was applied. The deformation is reversible, non-permanent.
• Plastic deformation . This occurs when a large stress is applied to a material. The stress is so large that when removed, the material does not spring back to its previous dimension. There is a permanent, irreversible deformation. The minimal value of the stress which produces plastic deformation is known as the elastic limit for the material.

Hooke's law

Young's modulus and combining springs, springs with mass, want to join the conversation.

• Upvote Button navigates to signup page
• Downvote Button navigates to signup page
• Flag Button navigates to signup page

• school Campus Bookshelves
• menu_book Bookshelves
• perm_media Learning Objects
• login Login
• how_to_reg Request Instructor Account
• hub Instructor Commons

Margin Size

• Download Page (PDF)
• Download Full Book (PDF)
• Periodic Table
• Physics Constants
• Scientific Calculator
• Reference & Cite
• Tools expand_more
• Readability

selected template will load here

This action is not available.

Elasticity and Plasticity

• Last updated
• Save as PDF
• Page ID 17978

\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)

\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)

\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)

( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)

\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)

\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)

\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)

\( \newcommand{\Span}{\mathrm{span}}\)

\( \newcommand{\id}{\mathrm{id}}\)

\( \newcommand{\kernel}{\mathrm{null}\,}\)

\( \newcommand{\range}{\mathrm{range}\,}\)

\( \newcommand{\RealPart}{\mathrm{Re}}\)

\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)

\( \newcommand{\Argument}{\mathrm{Arg}}\)

\( \newcommand{\norm}[1]{\| #1 \|}\)

\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)

\( \newcommand{\vectorA}[1]{\vec{#1}}      % arrow\)

\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}}      % arrow\)

\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)

\( \newcommand{\vectorC}[1]{\textbf{#1}} \)

\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)

\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)

\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)

Learning Objectives

• Explain the limit where a deformation of material is elastic
• Describe the range where materials show plastic behavior
• Analyze elasticity and plasticity on a stress-strain diagram

We referred to the proportionality constant between stress and strain as the elastic modulus . But why do we call it that? What does it mean for an object to be elastic and how do we describe its behavior?

Elasticity is the tendency of solid objects and materials to return to their original shape after the external forces (load) causing a deformation are removed. An object is elastic when it comes back to its original size and shape when the load is no longer present. Physical reasons for elastic behavior vary among materials and depend on the microscopic structure of the material. For example, the elasticity of polymers and rubbers is caused by stretching polymer chains under an applied force. In contrast, the elasticity of metals is caused by resizing and reshaping the crystalline cells of the lattices (which are the material structures of metals) under the action of externally applied forces.

The two parameters that determine the elasticity of a material are its elastic modulus and its elastic limit . A high elastic modulus is typical for materials that are hard to deform; in other words, materials that require a high load to achieve a significant strain. An example is a steel band. A low elastic modulus is typical for materials that are easily deformed under a load; for example, a rubber band. If the stress under a load becomes too high, then when the load is removed, the material no longer comes back to its original shape and size, but relaxes to a different shape and size: The material becomes permanently deformed. The elastic limit is the stress value beyond which the material no longer behaves elastically but becomes permanently deformed.

Our perception of an elastic material depends on both its elastic limit and its elastic modulus. For example, all rubbers are characterized by a low elastic modulus and a high elastic limit; hence, it is easy to stretch them and the stretch is noticeably large. Among materials with identical elastic limits, the most elastic is the one with the lowest elastic modulus.

When the load increases from zero, the resulting stress is in direct proportion to strain in the way given by Equation 12.4.4 , but only when stress does not exceed some limiting value. For stress values within this linear limit, we can describe elastic behavior in analogy with Hooke’s law for a spring. According to Hooke’s law, the stretch value of a spring under an applied force is directly proportional to the magnitude of the force. Conversely, the response force from the spring to an applied stretch is directly proportional to the stretch. In the same way, the deformation of a material under a load is directly proportional to the load, and, conversely, the resulting stress is directly proportional to strain. The linearity limit (or the proportionality limit ) is the largest stress value beyond which stress is no longer proportional to strain. Beyond the linearity limit, the relation between stress and strain is no longer linear. When stress becomes larger than the linearity limit but still within the elasticity limit, behavior is still elastic, but the relation between stress and strain becomes nonlinear.

For stresses beyond the elastic limit, a material exhibits plastic behavior . This means the material deforms irreversibly and does not return to its original shape and size, even when the load is removed. When stress is gradually increased beyond the elastic limit, the material undergoes plastic deformation. Rubber-like materials show an increase in stress with the increasing strain, which means they become more difficult to stretch and, eventually, they reach a fracture point where they break. Ductile materials such as metals show a gradual decrease in stress with the increasing strain, which means they become easier to deform as stress-strain values approach the breaking point. Microscopic mechanisms responsible for plasticity of materials are different for different materials.

We can graph the relationship between stress and strain on a stress-strain diagram . Each material has its own characteristic strain-stress curve. A typical stress-strain diagram for a ductile metal under a load is shown in Figure \(\PageIndex{1}\). In this figure, strain is a fractional elongation (not drawn to scale). When the load is gradually increased, the linear behavior (red line) that starts at the no-load point (the origin) ends at the linearity limit at point H. For further load increases beyond point H, the stress-strain relation is nonlinear but still elastic. In the figure, this nonlinear region is seen between points H and E. Ever larger loads take the stress to the elasticity limit E, where elastic behavior ends and plastic deformation begins. Beyond the elasticity limit, when the load is removed, for example at P, the material relaxes to a new shape and size along the green line. This is to say that the material becomes permanently deformed and does not come back to its initial shape and size when stress becomes zero.

The material undergoes plastic deformation for loads large enough to cause stress to go beyond the elasticity limit at E. The material continues to be plastically deformed until the stress reaches the fracture point (breaking point). Beyond the fracture point, we no longer have one sample of material, so the diagram ends at the fracture point. For the completeness of this qualitative description, it should be said that the linear, elastic, and plasticity limits denote a range of values rather than one sharp point.

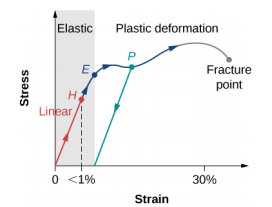
The value of stress at the fracture point is called breaking stress (or ultimate stress ). Materials with similar elastic properties, such as two metals, may have very different breaking stresses. For example, ultimate stress for aluminum is 2.2 x 10 8 Pa and for steel it may be as high as 20.0 x 10 8 Pa, depending on the kind of steel. We can make a quick estimate, based on Equation 12.4.5 , that for rods with a 1-in 2 cross-sectional area, the breaking load for an aluminum rod is 3.2 x 10 4 lb, and the breaking load for a steel rod is about nine times larger.

practice problem 1

Practice problem 2, practice problem 3, practice problem 4.

• Physics Concept Questions And Answers
• Elastic Collision Rectifier Questions

Elastic Collision Questions

A collision takes place when two or more bodies apply forces on each other in a short span of time. Collision is of two types: elastic collision and inelastic collision.

An elastic collision involves no loss in the net kinetic energy due to the collision.

An example of elastic collision is when a ball at a billiard table hits another ball.

Read more: Elastic Collision

Important Elastic Collision Questions with Answers

1. Choose the correct answer: Which type of collision takes place when velocities of both the particles are along the line of the collision?

• Oblique Collision
• Head-on Collision

Answer: b) Head-on Collision

Explanation: In a head-on collision, the velocities of both the particles are along the line of the collision.

2. What is the formula to find the elastic collision of momentum

• m 1 u 1 – m 2 u 2 = m 1 v 1 – m 2 v 2
• m 1 u 1 + m 2 u 2 = m 1 v 1 + m 2 v 2
• m 1 u 1 * m 2 u 2 = m 1 v 1 ^ m 2 v 2
• m 1 u 1 / m 2 u 2 = m 1 v 1 / m 2 v 2

Answer: b) m 1 u 1 + m 2 u 2 = m 1 v 1 + m 2 v 2

Explanation: Elastic collision formula of momentum is given by the formula: m 1 u 1 + m 2 u 2 = m 1 v 1 + m 2 v 2 .

3. What is the elastic collision formula of kinetic energy?

The formula for elastic collision of kinetic energy is given by 1/2 m 1 u 1 2 + 1/2 m 2 u 2 2 = 1/2 m 1 v 1 2 + 1/2 m 2 v 2 2 .

4. In which type of collision the total kinetic energy is conserved?

• Inelastic collision
• Elastic collision

Answer: b) Elastic collision

Explanation: The total kinetic energy is conserved in an elastic collision.

5. State true or false: In an elastic collision, momentum is not conserved.

Answer: b) FALSE

Explanation: In an elastic collision, momentum is not conserved.

6. Collision of two cars is an example of ______

Answer: a) Inelastic collision

Explanation: The collision of two cars is an ideal example of an inelastic collision since it involves a change in kinetic energy.

7. Define the coefficient of restitution.

It is defined as the ratio of the velocity of separation by the velocity of approach along the line of collision.

Coefficient of restitution (e) = Relative Velocity after collision / Relative Velocity after collision

8. Which among the following is a collision in two dimensions?

Answer: a) Oblique Collision

Explanation: Oblique Collision is a type of collision in two dimensions.

9. Is head-on collision a one dimension collision?

Answer: a) YES

Explanation Yes, a head-on collision is an example of one dimension collision.

10. Does conversion of energy takes place in an elastic collision?

Explanation: In an elastic collision, no conversion of energy takes place.

Practice Questions

• What is meant by collision?
• Explain inelastic collision with an example.
• Explain elastic collision with an example.
• In which type of collision the total kinetic energy is conserved.
• Which type of collision is considered a one-dimensional collision?

Watch the video and learn more about collision

• Share Share

Register with BYJU'S & Download Free PDFs

Register with byju's & watch live videos.