how to solve projectile motion problems easily

• PRO Courses Guides New Tech Help Pro Expert Videos About wikiHow Pro Upgrade Sign In
• EDIT Edit this Article
• EXPLORE Tech Help Pro About Us Random Article Quizzes Request a New Article Community Dashboard This Or That Game Popular Categories Arts and Entertainment Artwork Books Movies Computers and Electronics Computers Phone Skills Technology Hacks Health Men's Health Mental Health Women's Health Relationships Dating Love Relationship Issues Hobbies and Crafts Crafts Drawing Games Education & Communication Communication Skills Personal Development Studying Personal Care and Style Fashion Hair Care Personal Hygiene Youth Personal Care School Stuff Dating All Categories Arts and Entertainment Finance and Business Home and Garden Relationship Quizzes Cars & Other Vehicles Food and Entertaining Personal Care and Style Sports and Fitness Computers and Electronics Health Pets and Animals Travel Education & Communication Hobbies and Crafts Philosophy and Religion Work World Family Life Holidays and Traditions Relationships Youth
• Browse Articles
• Learn Something New
• Quizzes Hot
• This Or That Game
• Train Your Brain
• Explore More
• Support wikiHow
• About wikiHow
• Log in / Sign up
• Education and Communications
• Classical Mechanics

How to Solve a Projectile Motion Problem

Last Updated: May 30, 2024

This article was co-authored by Grace Imson, MA . Grace Imson is a math teacher with over 40 years of teaching experience. Grace is currently a math instructor at the City College of San Francisco and was previously in the Math Department at Saint Louis University. She has taught math at the elementary, middle, high school, and college levels. She has an MA in Education, specializing in Administration and Supervision from Saint Louis University. This article has been viewed 77,292 times.

Projectile motion can feel like an intimidating subject in physics, but we're here to help you learn everything you need to know. Most of the time, you need to solve for a few other variables to get the answer you are looking for. This means in order to find the distance an object traveled, you might first have to find the time it took or the initial velocity first. Just follow these steps and you should be able to fly through projectile motion problems!

• (1) an object is thrown off a higher ground than what it will land on.
• (2) the object starts on the ground, soars through the air, and then lands on the ground some distance away from where it started.

Community Q&A

You Might Also Like

About This Article

• Send fan mail to authors

Reader Success Stories

Feb 25, 2019

Did this article help you?

Cyril Shenouda

Feb 5, 2018

Nicholas Bunge

Aug 13, 2022

Featured Articles

Trending Articles

Watch Articles

• Terms of Use
• Privacy Policy
• Do Not Sell or Share My Info
• Not Selling Info

Don’t miss out! Sign up for

wikiHow’s newsletter

4.3 Projectile Motion

Learning objectives.

By the end of this section, you will be able to:

• Use one-dimensional motion in perpendicular directions to analyze projectile motion.
• Calculate the range, time of flight, and maximum height of a projectile that is launched and impacts a flat, horizontal surface.
• Find the time of flight and impact velocity of a projectile that lands at a different height from that of launch.
• Calculate the trajectory of a projectile.

Projectile motion is the motion of an object thrown or projected into the air, subject only to acceleration as a result of gravity. The applications of projectile motion in physics and engineering are numerous. Some examples include meteors as they enter Earth’s atmosphere, fireworks, and the motion of any ball in sports. Such objects are called projectiles and their path is called a trajectory . The motion of falling objects as discussed in Motion Along a Straight Line is a simple one-dimensional type of projectile motion in which there is no horizontal movement. In this section, we consider two-dimensional projectile motion, and our treatment neglects the effects of air resistance.

The most important fact to remember here is that motions along perpendicular axes are independent and thus can be analyzed separately. We discussed this fact in Displacement and Velocity Vectors , where we saw that vertical and horizontal motions are independent. The key to analyzing two-dimensional projectile motion is to break it into two motions: one along the horizontal axis and the other along the vertical. (This choice of axes is the most sensible because acceleration resulting from gravity is vertical; thus, there is no acceleration along the horizontal axis when air resistance is negligible.) As is customary, we call the horizontal axis the x -axis and the vertical axis the y -axis. It is not required that we use this choice of axes; it is simply convenient in the case of gravitational acceleration. In other cases we may choose a different set of axes. Figure 4.11 illustrates the notation for displacement, where we define s → s → to be the total displacement, and x → x → and y → y → are its component vectors along the horizontal and vertical axes, respectively. The magnitudes of these vectors are s , x , and y .

To describe projectile motion completely, we must include velocity and acceleration, as well as displacement. We must find their components along the x- and y -axes. Let’s assume all forces except gravity (such as air resistance and friction, for example) are negligible. Defining the positive direction to be upward, the components of acceleration are then very simple:

Because gravity is vertical, a x = 0 . a x = 0 . If a x = 0 , a x = 0 , this means the initial velocity in the x direction is equal to the final velocity in the x direction, or v x = v 0 x . v x = v 0 x . With these conditions on acceleration and velocity, we can write the kinematic Equation 4.11 through Equation 4.18 for motion in a uniform gravitational field, including the rest of the kinematic equations for a constant acceleration from Motion with Constant Acceleration . The kinematic equations for motion in a uniform gravitational field become kinematic equations with a y = − g , a x = 0 : a y = − g , a x = 0 :

Horizontal Motion

Vertical Motion

Using this set of equations, we can analyze projectile motion, keeping in mind some important points.

Problem-Solving Strategy

Projectile motion.

• Resolve the motion into horizontal and vertical components along the x - and y -axes. The magnitudes of the components of displacement s → s → along these axes are x and y. The magnitudes of the components of velocity v → v → are v x = v cos θ and v y = v sin θ , v x = v cos θ and v y = v sin θ , where v is the magnitude of the velocity and θ is its direction relative to the horizontal, as shown in Figure 4.12 .
• Treat the motion as two independent one-dimensional motions: one horizontal and the other vertical. Use the kinematic equations for horizontal and vertical motion presented earlier.
• Solve for the unknowns in the two separate motions: one horizontal and one vertical. Note that the only common variable between the motions is time t . The problem-solving procedures here are the same as those for one-dimensional kinematics and are illustrated in the following solved examples.
• Recombine quantities in the horizontal and vertical directions to find the total displacement s → s → and velocity v → . v → . Solve for the magnitude and direction of the displacement and velocity using s = x 2 + y 2 , Φ = tan −1 ( y / x ) , v = v x 2 + v y 2 , s = x 2 + y 2 , Φ = tan −1 ( y / x ) , v = v x 2 + v y 2 , where Φ is the direction of the displacement s → . s → .

Example 4.7

A fireworks projectile explodes high and away.

Because y 0 y 0 and v y v y are both zero, the equation simplifies to

Solving for y gives

Now we must find v 0 y , v 0 y , the component of the initial velocity in the y direction. It is given by v 0 y = v 0 sin θ 0 , v 0 y = v 0 sin θ 0 , where v 0 v 0 is the initial velocity of 70.0 m/s and θ 0 = 75 ° θ 0 = 75 ° is the initial angle. Thus,

Thus, we have

Note that because up is positive, the initial vertical velocity is positive, as is the maximum height, but the acceleration resulting from gravity is negative. Note also that the maximum height depends only on the vertical component of the initial velocity, so that any projectile with a 67.6-m/s initial vertical component of velocity reaches a maximum height of 233 m (neglecting air resistance). The numbers in this example are reasonable for large fireworks displays, the shells of which do reach such heights before exploding. In practice, air resistance is not completely negligible, so the initial velocity would have to be somewhat larger than that given to reach the same height.

(b) As in many physics problems, there is more than one way to solve for the time the projectile reaches its highest point. In this case, the easiest method is to use v y = v 0 y − g t . v y = v 0 y − g t . Because v y = 0 v y = 0 at the apex, this equation reduces to simply

This time is also reasonable for large fireworks. If you are able to see the launch of fireworks, notice that several seconds pass before the shell explodes. Another way of finding the time is by using y = y 0 + 1 2 ( v 0 y + v y ) t . y = y 0 + 1 2 ( v 0 y + v y ) t . This is left for you as an exercise to complete.

(c) Because air resistance is negligible, a x = 0 a x = 0 and the horizontal velocity is constant, as discussed earlier. The horizontal displacement is the horizontal velocity multiplied by time as given by x = x 0 + v x t , x = x 0 + v x t , where x 0 x 0 is equal to zero. Thus,

where v x v x is the x -component of the velocity, which is given by

Time t for both motions is the same, so x is

Horizontal motion is a constant velocity in the absence of air resistance. The horizontal displacement found here could be useful in keeping the fireworks fragments from falling on spectators. When the shell explodes, air resistance has a major effect, and many fragments land directly below.

(d) The horizontal and vertical components of the displacement were just calculated, so all that is needed here is to find the magnitude and direction of the displacement at the highest point:

Note that the angle for the displacement vector is less than the initial angle of launch. To see why this is, review Figure 4.11 , which shows the curvature of the trajectory toward the ground level.

When solving Example 4.7 (a), the expression we found for y is valid for any projectile motion when air resistance is negligible. Call the maximum height y = h . Then,

This equation defines the maximum height of a projectile above its launch position and it depends only on the vertical component of the initial velocity.

Check Your Understanding 4.3

A rock is thrown horizontally off a cliff 100.0 m 100.0 m high with a velocity of 15.0 m/s. (a) Define the origin of the coordinate system. (b) Which equation describes the horizontal motion? (c) Which equations describe the vertical motion? (d) What is the rock’s velocity at the point of impact?

Example 4.8

Calculating projectile motion: tennis player.

If we take the initial position y 0 y 0 to be zero, then the final position is y = 10 m. The initial vertical velocity is the vertical component of the initial velocity:

Substituting into Equation 4.22 for y gives us

Rearranging terms gives a quadratic equation in t :

Use of the quadratic formula yields t = 3.79 s and t = 0.54 s. Since the ball is at a height of 10 m at two times during its trajectory—once on the way up and once on the way down—we take the longer solution for the time it takes the ball to reach the spectator:

The time for projectile motion is determined completely by the vertical motion. Thus, any projectile that has an initial vertical velocity of 21.2 m/s and lands 10.0 m above its starting altitude spends 3.79 s in the air.

(b) We can find the final horizontal and vertical velocities v x v x and v y v y with the use of the result from (a). Then, we can combine them to find the magnitude of the total velocity vector v → v → and the angle θ θ it makes with the horizontal. Since v x v x is constant, we can solve for it at any horizontal location. We choose the starting point because we know both the initial velocity and the initial angle. Therefore,

The final vertical velocity is given by Equation 4.21 :

Since v 0 y v 0 y was found in part (a) to be 21.2 m/s, we have

The magnitude of the final velocity v → v → is

The direction θ v θ v is found using the inverse tangent:

Significance

Time of flight, trajectory, and range.

Of interest are the time of flight, trajectory, and range for a projectile launched on a flat horizontal surface and impacting on the same surface. In this case, kinematic equations give useful expressions for these quantities, which are derived in the following sections.

Time of flight

We can solve for the time of flight of a projectile that is both launched and impacts on a flat horizontal surface by performing some manipulations of the kinematic equations. We note the position and displacement in y must be zero at launch and at impact on an even surface. Thus, we set the displacement in y equal to zero and find

Factoring, we have

Solving for t gives us

This is the time of flight for a projectile both launched and impacting on a flat horizontal surface. Equation 4.24 does not apply when the projectile lands at a different elevation than it was launched, as we saw in Example 4.8 of the tennis player hitting the ball into the stands. The other solution, t = 0, corresponds to the time at launch. The time of flight is linearly proportional to the initial velocity in the y direction and inversely proportional to g . Thus, on the Moon, where gravity is one-sixth that of Earth, a projectile launched with the same velocity as on Earth would be airborne six times as long.

The trajectory of a projectile can be found by eliminating the time variable t from the kinematic equations for arbitrary t and solving for y ( x ). We take x 0 = y 0 = 0 x 0 = y 0 = 0 so the projectile is launched from the origin. The kinematic equation for x gives

Substituting the expression for t into the equation for the position y = ( v 0 sin θ 0 ) t − 1 2 g t 2 y = ( v 0 sin θ 0 ) t − 1 2 g t 2 gives

Rearranging terms, we have

This trajectory equation is of the form y = a x + b x 2 , y = a x + b x 2 , which is an equation of a parabola with coefficients

From the trajectory equation we can also find the range , or the horizontal distance traveled by the projectile. Factoring Equation 4.25 , we have

The position y is zero for both the launch point and the impact point, since we are again considering only a flat horizontal surface. Setting y = 0 in this equation gives solutions x = 0, corresponding to the launch point, and

corresponding to the impact point. Using the trigonometric identity 2 sin θ cos θ = sin 2 θ 2 sin θ cos θ = sin 2 θ and setting x = R for range, we find

Note particularly that Equation 4.26 is valid only for launch and impact on a horizontal surface. We see the range is directly proportional to the square of the initial speed v 0 v 0 and sin 2 θ 0 sin 2 θ 0 , and it is inversely proportional to the acceleration of gravity. Thus, on the Moon, the range would be six times greater than on Earth for the same initial velocity. Furthermore, we see from the factor sin 2 θ 0 sin 2 θ 0 that the range is maximum at 45 ° . 45 ° . These results are shown in Figure 4.15 . In (a) we see that the greater the initial velocity, the greater the range. In (b), we see that the range is maximum at 45 ° . 45 ° . This is true only for conditions neglecting air resistance. If air resistance is considered, the maximum angle is somewhat smaller. It is interesting that the same range is found for two initial launch angles that sum to 90 ° . 90 ° . The projectile launched with the smaller angle has a lower apex than the higher angle, but they both have the same range.

Example 4.9

Comparing golf shots.

(a) What is the initial speed of the ball at the second hole?

(b) What is the initial speed of the ball at the fourth hole?

(c) Write the trajectory equation for both cases.

(d) Graph the trajectories.

(b) R = v 0 2 sin 2 θ 0 g ⇒ v 0 = R g sin 2 θ 0 = 90.0 m ( 9.8 m / s 2 ) sin ( 2 ( 70 ° ) ) = 37.0 m / s R = v 0 2 sin 2 θ 0 g ⇒ v 0 = R g sin 2 θ 0 = 90.0 m ( 9.8 m / s 2 ) sin ( 2 ( 70 ° ) ) = 37.0 m / s

(c) y = x [ tan θ 0 − g 2 ( v 0 cos θ 0 ) 2 x ] Second hole: y = x [ tan 30 ° − 9.8 m / s 2 2 [ ( 31.9 m / s)( cos 30 ° ) ] 2 x ] = 0.58 x − 0.0064 x 2 Fourth hole: y = x [ tan 70 ° − 9.8 m / s 2 2 [ ( 37.0 m / s)( cos 70 ° ) ] 2 x ] = 2.75 x − 0.0306 x 2 y = x [ tan θ 0 − g 2 ( v 0 cos θ 0 ) 2 x ] Second hole: y = x [ tan 30 ° − 9.8 m / s 2 2 [ ( 31.9 m / s)( cos 30 ° ) ] 2 x ] = 0.58 x − 0.0064 x 2 Fourth hole: y = x [ tan 70 ° − 9.8 m / s 2 2 [ ( 37.0 m / s)( cos 70 ° ) ] 2 x ] = 2.75 x − 0.0306 x 2

(d) Using a graphing utility, we can compare the two trajectories, which are shown in Figure 4.16 .

Check Your Understanding 4.4

If the two golf shots in Example 4.9 were launched at the same speed, which shot would have the greatest range?

When we speak of the range of a projectile on level ground, we assume R is very small compared with the circumference of Earth. If, however, the range is large, Earth curves away below the projectile and the acceleration resulting from gravity changes direction along the path. The range is larger than predicted by the range equation given earlier because the projectile has farther to fall than it would on level ground, as shown in Figure 4.17 , which is based on a drawing in Newton’s Principia. If the initial speed is great enough, the projectile goes into orbit. Earth’s surface drops 5 m every 8000 m. In 1 s an object falls 5 m without air resistance. Thus, if an object is given a horizontal velocity of 8000 m/s (or 18,000 mi/hr) near Earth’s surface, it will go into orbit around the planet because the surface continuously falls away from the object. This is roughly the speed of the Space Shuttle in a low Earth orbit when it was operational, or any satellite in a low Earth orbit. These and other aspects of orbital motion, such as Earth’s rotation, are covered in greater depth in Gravitation .

Interactive

At PhET Explorations: Projectile Motion , learn about projectile motion in terms of the launch angle and initial velocity.

As an Amazon Associate we earn from qualifying purchases.

This book may not be used in the training of large language models or otherwise be ingested into large language models or generative AI offerings without OpenStax's permission.

Want to cite, share, or modify this book? This book uses the Creative Commons Attribution License and you must attribute OpenStax.

Access for free at https://openstax.org/books/university-physics-volume-1/pages/1-introduction

• Authors: William Moebs, Samuel J. Ling, Jeff Sanny
• Publisher/website: OpenStax
• Book title: University Physics Volume 1
• Publication date: Sep 19, 2016
• Location: Houston, Texas
• Book URL: https://openstax.org/books/university-physics-volume-1/pages/1-introduction
• Section URL: https://openstax.org/books/university-physics-volume-1/pages/4-3-projectile-motion

© Jan 19, 2024 OpenStax. Textbook content produced by OpenStax is licensed under a Creative Commons Attribution License . The OpenStax name, OpenStax logo, OpenStax book covers, OpenStax CNX name, and OpenStax CNX logo are not subject to the Creative Commons license and may not be reproduced without the prior and express written consent of Rice University.

Physics Problems with Solutions

• Electric Circuits
• Electrostatic
• Calculators
• Practice Tests
• Simulations

• Projectile Problems with Solutions and Explanations

Projectile problems are presented along with detailed solutions . These problems may be better understood when projectile equations are first reviewed. An interactive html 5 applet may be used to better understand the projectile equations.

Problems with Detailed Solutions

An object is launched at a velocity of 20 m/s in a direction making an angle of 25° upward with the horizontal. a) What is the maximum height reached by the object? b) What is the total flight time (between launch and touching the ground) of the object? c) What is the horizontal range (maximum x above ground) of the object? d) What is the magnitude of the velocity of the object just before it hits the ground? Solution to Problem 1

A ball kicked from ground level at an initial velocity of 60 m/s and an angle θ with ground reaches a horizontal distance of 200 meters. a) What is the size of angle θ? b) What is time of flight of the ball? Solution to Problem 5

A ball of 600 grams is kicked at an angle of 35° with the ground with an initial velocity V 0 . a) What is the initial velocity V 0 of the ball if its kinetic energy is 22 Joules when its height is maximum? b) What is the maximum height reached by the ball Solution to Problem 6

A projectile starting from ground hits a target on the ground located at a distance of 1000 meters after 40 seconds. a) What is the size of the angle θ? b) At what initial velocity was the projectile launched? Solution to Problem 7

The trajectory of a projectile launched from ground is given by the equation y = -0.025 x 2 + 0.5 x, where x and y are the coordinate of the projectile on a rectangular system of axes. a) Find the initial velocity and the angle at which the projectile is launched. Solution to Problem 8

Two balls A and B of masses 100 grams and 300 grams respectively are pushed horizontally from a table of height 3 meters. Ball has is pushed so that its initial velocity is 10 m/s and ball B is pushed so that its initial velocity is 15 m/s. a) Find the time it takes each ball to hit the ground. b) What is the difference in the distance between the points of impact of the two balls on the ground? Solution to Problem 9

More References and Links

• Projectile Motion Calculator and Solver
• Solutions and Explanations to Projectile Problems
• Projectile Equations with Explanations
• Interactive Simulation of Projectile .

Popular Pages

• Privacy Policy

Two-Dimensional Kinematics

Projectile motion, learning objectives.

By the end of this section, you will be able to:

• Identify and explain the properties of a projectile, such as acceleration due to gravity, range, maximum height, and trajectory.
• Determine the location and velocity of a projectile at different points in its trajectory.
• Apply the principle of independence of motion to solve projectile motion problems.

Projectile motion is the motion of an object thrown or projected into the air, subject to only the acceleration of gravity. The object is called a projectile , and its path is called its trajectory . The motion of falling objects, as covered in Problem-Solving Basics for One-Dimensional Kinematics, is a simple one-dimensional type of projectile motion in which there is no horizontal movement. In this section, we consider two-dimensional projectile motion, such as that of a football or other object for which air resistance  is negligible .

The most important fact to remember here is that motions along perpendicular axes are independent and thus can be analyzed separately. This fact was discussed in Kinematics in Two Dimensions: An Introduction , where vertical and horizontal motions were seen to be independent. The key to analyzing two-dimensional projectile motion is to break it into two motions, one along the horizontal axis and the other along the vertical. (This choice of axes is the most sensible, because acceleration due to gravity is vertical—thus, there will be no acceleration along the horizontal axis when air resistance is negligible.) As is customary, we call the horizontal axis the x -axis and the vertical axis the y -axis. Figure 1 illustrates the notation for displacement, where s is defined to be the total displacement and x and y are its components along the horizontal and vertical axes, respectively. The magnitudes of these vectors are s , x , and y . (Note that in the last section we used the notation A to represent a vector with components A x and A y . If we continued this format, we would call displacement s with components s x and s y . However, to simplify the notation, we will simply represent the component vectors as x and y .)

Of course, to describe motion we must deal with velocity and acceleration, as well as with displacement. We must find their components along the x – and y -axes, too. We will assume all forces except gravity (such as air resistance and friction, for example) are negligible. The components of acceleration are then very simple: a y  = – g  = –9.80 m/s 2 . (Note that this definition assumes that the upwards direction is defined as the positive direction. If you arrange the coordinate system instead such that the downwards direction is positive, then acceleration due to gravity takes a positive value.) Because gravity is vertical, a x =0. Both accelerations are constant, so the kinematic equations can be used.

Review of Kinematic Equations (constant a )

Figure 1. The total displacement s of a soccer ball at a point along its path. The vector s has components x and y along the horizontal and vertical axes. Its magnitude is s, and it makes an angle θ with the horizontal.

Given these assumptions, the following steps are then used to analyze projectile motion:

Step 1.  Resolve or break the motion into horizontal and vertical components along the x- and y-axes. These axes are perpendicular, so A x = A cos θ  and  A y  = A sin θ  are used. The magnitude of the components of displacement s along these axes are x and y. The magnitudes of the components of the velocity v are V x = V cos θ  and V y = v sin θ  where v is the magnitude of the velocity and θ is its direction, as shown in 2. Initial values are denoted with a subscript 0, as usual.

Step 2. Treat the motion as two independent one-dimensional motions, one horizontal and the other vertical. The kinematic equations for horizontal and vertical motion take the following forms:

Step 3. Solve for the unknowns in the two separate motions—one horizontal and one vertical. Note that the only common variable between the motions is time t . The problem solving procedures here are the same as for one-dimensional kinematics and are illustrated in the solved examples below.

Step 4.  Recombine the two motions to find the total displacement s and velocity v. Because the x – and y -motions are perpendicular, we determine these vectors by using the techniques outlined in the Vector Addition and Subtraction: Analytical Methods and employing [latex]A=\sqrt{{{A}_{x}}^{2}+{{A}_{y}}^{2}}\\[/latex] and θ  = tan −1  ( A y / A x ) in the following form, where θ is the direction of the displacement s and θ v is the direction of the velocity v :

Total displacement and velocity

Figure 2. (a) We analyze two-dimensional projectile motion by breaking it into two independent one-dimensional motions along the vertical and horizontal axes. (b) The horizontal motion is simple, because ax=0 and vx is thus constant. (c) The velocity in the vertical direction begins to decrease as the object rises; at its highest point, the vertical velocity is zero. As the object falls towards the Earth again, the vertical velocity increases again in magnitude but points in the opposite direction to the initial vertical velocity. (d) The x – and y -motions are recombined to give the total velocity at any given point on the trajectory.

Example 1. A Fireworks Projectile Explodes High and Away

During a fireworks display, a shell is shot into the air with an initial speed of 70.0 m/s at an angle of 75.0º above the horizontal, as illustrated in Figure 3. The fuse is timed to ignite the shell just as it reaches its highest point above the ground. (a) Calculate the height at which the shell explodes. (b) How much time passed between the launch of the shell and the explosion? (c) What is the horizontal displacement of the shell when it explodes?

Because air resistance is negligible for the unexploded shell, the analysis method outlined above can be used. The motion can be broken into horizontal and vertical motions in which a x  = 0 and a y  = – g . We can then define x 0 and y 0 to be zero and solve for the desired quantities.

Solution for (a)

By “height” we mean the altitude or vertical position y above the starting point. The highest point in any trajectory, called the apex, is reached when v y =0. Since we know the initial and final velocities as well as the initial position, we use the following equation to find y :

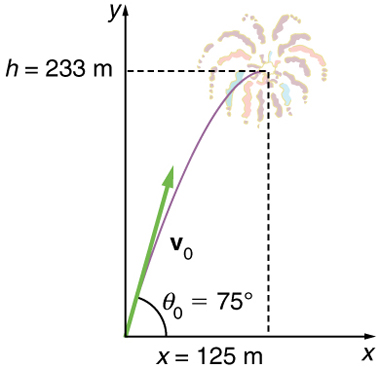
Figure 3. The trajectory of a fireworks shell. The fuse is set to explode the shell at the highest point in its trajectory, which is found to be at a height of 233 m and 125 m away horizontally.

Because y 0 and v y are both zero, the equation simplifies to

Solving for y gives

Now we must find v 0 y , the component of the initial velocity in the y -direction. It is given by v 0y = v 0 sin  θ , where v 0 y is the initial velocity of 70.0 m/s, and θ 0  = 75.0º is the initial angle. Thus,

v Oy = v 0 sin  θ 0 = (70.0 m/s)(sin 75º) = 67.6 m/s

[latex]y=\frac{\left(67.6\text{ m/s}\right)^{2}}{2\left(9.80\text{ m/s}^{2}\right)}\\[/latex] ,

Discussion for (a)

Note that because up is positive, the initial velocity is positive, as is the maximum height, but the acceleration due to gravity is negative. Note also that the maximum height depends only on the vertical component of the initial velocity, so that any projectile with a 67.6 m/s initial vertical component of velocity will reach a maximum height of 233 m (neglecting air resistance). The numbers in this example are reasonable for large fireworks displays, the shells of which do reach such heights before exploding. In practice, air resistance is not completely negligible, and so the initial velocity would have to be somewhat larger than that given to reach the same height.

Solution for (b)

As in many physics problems, there is more than one way to solve for the time to the highest point. In this case, the easiest method is to use [latex]y={y}_{0}+\frac{1}{2}\left({v}_{0y}+{v}_{y}\right)t\\[/latex]. Because y 0 is zero, this equation reduces to simply

[latex]y=\frac{1}{2}\left({v}_{0y}+{v}_{y}\right)t\\[/latex].

Note that the final vertical velocity, v y , at the highest point is zero. Thus,

[latex]\begin{array}{lll}t& =& \frac{2y}{\left({v}_{0y}+{v}_{y}\right)}=\frac{2\left(\text{233 m}\right)}{\left(\text{67.6 m/s}\right)}\\ & =& 6.90\text{ s}\end{array}\\[/latex].

Discussion for (b)

This time is also reasonable for large fireworks. When you are able to see the launch of fireworks, you will notice several seconds pass before the shell explodes. (Another way of finding the time is by using [latex]y={y}_{0}+{v}_{0y}t-\frac{1}{2}{\text{gt}}^{2}\\[/latex], and solving the quadratic equation for t .)

Solution for (c)

Because air resistance is negligible, a x =0 and the horizontal velocity is constant, as discussed above. The horizontal displacement is horizontal velocity multiplied by time as given by x  =  x 0  +  v x t , where x 0 is equal to zero:

x  =  v x t ,

where v x is the x -component of the velocity, which is given by v x = v 0 cos θ 0  Now,

v x  = v 0  cos  θ 0 = (70.0 m/s)(cos 75º) = 18.1 m/s

The time t for both motions is the same, and so x is

x = (18.1 m/s)(6.90 s) = 125 m.

Discussion for (c)

The horizontal motion is a constant velocity in the absence of air resistance. The horizontal displacement found here could be useful in keeping the fireworks fragments from falling on spectators. Once the shell explodes, air resistance has a major effect, and many fragments will land directly below.

In solving part (a) of the preceding example, the expression we found for y is valid for any projectile motion where air resistance is negligible. Call the maximum height y = h ; then,

[latex]h=\frac{{{v}_{0y}}^{2}}{2g}\\[/latex].

This equation defines the maximum height of a projectile and depends only on the vertical component of the initial velocity.

Defining a Coordinate System

Example 2. calculating projectile motion: hot rock projectile.

Kilauea in Hawaii is the world’s most continuously active volcano. Very active volcanoes characteristically eject red-hot rocks and lava rather than smoke and ash. Suppose a large rock is ejected from the volcano with a speed of 25.0 m/s and at an angle 35.0º above the horizontal, as shown in Figure 4. The rock strikes the side of the volcano at an altitude 20.0 m lower than its starting point. (a) Calculate the time it takes the rock to follow this path. (b) What are the magnitude and direction of the rock’s velocity at impact?

Figure 4. The trajectory of a rock ejected from the Kilauea volcano.

Again, resolving this two-dimensional motion into two independent one-dimensional motions will allow us to solve for the desired quantities. The time a projectile is in the air is governed by its vertical motion alone. We will solve for t first. While the rock is rising and falling vertically, the horizontal motion continues at a constant velocity. This example asks for the final velocity. Thus, the vertical and horizontal results will be recombined to obtain v and θ v at the final time t determined in the first part of the example.

While the rock is in the air, it rises and then falls to a final position 20.0 m lower than its starting altitude. We can find the time for this by using

[latex]y={y}_{0}+{v}_{0y}t-\frac{1}{2}{\text{gt}}^{2}\\[/latex].

If we take the initial position y 0 to be zero, then the final position is y  = −20.0 m. Now the initial vertical velocity is the vertical component of the initial velocity, found from  v Oy = v 0 sin  θ 0  = (25.0 m/s)(sin 35.0º) = 14.3 m/s. Substituting known values yields

Rearranging terms gives a quadratic equation in t :

This expression is a quadratic equation of the form at 2 + bt + c = 0 , where the constants are a  = 4.90 , b  = –14.3 , and c  = –20.0. Its solutions are given by the quadratic formula:

[latex]t=\frac{-bpm \sqrt{{b}^{2}-4\text{ac}}}{\text{2}\text{a}}\\[/latex]

This equation yields two solutions: t  = 3.96 and t  = –1.03. (It is left as an exercise for the reader to verify these solutions.) The time is t = 3.96 s or -1.03 s. The negative value of time implies an event before the start of motion, and so we discard it. Thus,

The time for projectile motion is completely determined by the vertical motion. So any projectile that has an initial vertical velocity of 14.3 m/s and lands 20.0 m below its starting altitude will spend 3.96 s in the air.

From the information now in hand, we can find the final horizontal and vertical velocities v x and v y and combine them to find the total velocity v and the angle θ 0 it makes with the horizontal. Of course, v x is constant so we can solve for it at any horizontal location. In this case, we chose the starting point since we know both the initial velocity and initial angle. Therefore:

  v x  = v 0  cos  θ 0  = (25.0 m/s)(cos 35º) = 20.5 m/s

The final vertical velocity is given by the following equation:

[latex]{v}_{y}={v}_{0y}\text{gt}\\[/latex],

where v 0y was found in part (a) to be 14.3 m/s. Thus,

To find the magnitude of the final velocity v we combine its perpendicular components, using the following equation:

[latex]v=\sqrt{{{v}_{x}}^{2}+{{v}_{y}}^{2}}=\sqrt{({20.5}\text{ m/s})^{2}+{({-24.5}\text{ m/s})^{2}}}\\[/latex]

which gives

The direction θ v is found from the equation:

The negative angle means that the velocity is 50.1º below the horizontal. This result is consistent with the fact that the final vertical velocity is negative and hence downward—as you would expect because the final altitude is 20.0 m lower than the initial altitude. (See Figure 4.)

Figure 5. Trajectories of projectiles on level ground. (a) The greater the initial speed v0, the greater the range for a given initial angle. (b) The effect of initial angle θ 0  on the range of a projectile with a given initial speed. Note that the range is the same for 15º and 75º, although the maximum heights of those paths are different.

How does the initial velocity of a projectile affect its range? Obviously, the greater the initial speed v 0 , the greater the range, as shown in Figure 5(a). The initial angle θ 0 also has a dramatic effect on the range, as illustrated in Figure 5(b). For a fixed initial speed, such as might be produced by a cannon, the maximum range is obtained with θ 0  = 45º. This is true only for conditions neglecting air resistance. If air resistance is considered, the maximum angle is approximately 38º. Interestingly, for every initial angle except 45º, there are two angles that give the same range—the sum of those angles is 90º. The range also depends on the value of the acceleration of gravity g . The lunar astronaut Alan Shepherd was able to drive a golf ball a great distance on the Moon because gravity is weaker there. The range R of a projectile on level ground for which air resistance is negligible is given by

[latex]R=\frac{{{v}_{0}}^{2}\sin{2\theta }_{0}}{g}\\[/latex],

where v 0 is the initial speed and θ 0 is the initial angle relative to the horizontal. The proof of this equation is left as an end-of-chapter problem (hints are given), but it does fit the major features of projectile range as described. When we speak of the range of a projectile on level ground, we assume that R is very small compared with the circumference of the Earth. If, however, the range is large, the Earth curves away below the projectile and acceleration of gravity changes direction along the path. The range is larger than predicted by the range equation given above because the projectile has farther to fall than it would on level ground. (See Figure 6.) If the initial speed is great enough, the projectile goes into orbit. This is called escape velocity. This possibility was recognized centuries before it could be accomplished. When an object is in orbit, the Earth curves away from underneath the object at the same rate as it falls. The object thus falls continuously but never hits the surface. These and other aspects of orbital motion, such as the rotation of the Earth, will be covered analytically and in greater depth later in this text. Once again we see that thinking about one topic, such as the range of a projectile, can lead us to others, such as the Earth orbits. In Addition of Velocities , we will examine the addition of velocities, which is another important aspect of two-dimensional kinematics and will also yield insights beyond the immediate topic.

Figure 6. Projectile to satellite. In each case shown here, a projectile is launched from a very high tower to avoid air resistance. With increasing initial speed, the range increases and becomes longer than it would be on level ground because the Earth curves away underneath its path. With a large enough initial speed, orbit is achieved.

PhET Explorations: Projectile Motion

Click to run the simulation.

Section Summary

• Projectile motion is the motion of an object through the air that is subject only to the acceleration of gravity.
• To solve projectile motion problems, perform the following steps:

1. Determine a coordinate system. Then, resolve the position and/or velocity of the object in the horizontal and vertical components. The components of position s are given by the quantities x  and y , and the components of the velocity v are given by v x =   v cos θ  and v y = v sin θ , where v  is the magnitude of the velocity and  θ  is its direction.

2. Analyze the motion of the projectile in the horizontal direction using the following equations:

Horizontal Motion ( a x = 0)

3. Analyze the motion of the projectile in the vertical direction using the following equations:

Vertical Motion (assuming positive is up a y = -g = -9.8 m/s 2 )

4. Recombine the horizontal and vertical components of location and/or velocity using the following equations:

• The maximum height  h of a projectile launched with initial vertical velocity v 0y is given by [latex]h=\frac{{{v}_{0y}}^{2}}{2g}\\[/latex].
• The maximum horizontal distance traveled by a projectile is called the range . The range R of a projectile on level ground launched at an angle  θ 0   above the horizontal with initial speed v 0 is given by [latex]R=\frac{{{{v}_{0}}^{2}}\text{\sin}{2\theta }_{0}}{g}\\[/latex].

Conceptual Questions

1. Answer the following questions for projectile motion on level ground assuming negligible air resistance (the initial angle being neither 0º nor 90º): (a) Is the velocity ever zero? (b) When is the velocity a minimum? A maximum? (c) Can the velocity ever be the same as the initial velocity at a time other than at t = 0? (d) Can the speed ever be the same as the initial speed at a time other than at  t = 0?

2. Answer the following questions for projectile motion on level ground assuming negligible air resistance (the initial angle being neither 0º nor 90º): (a) Is the acceleration ever zero? (b) Is the acceleration ever in the same direction as a component of velocity? (c) Is the acceleration ever opposite in direction to a component of velocity?

3. For a fixed initial speed, the range of a projectile is determined by the angle at which it is fired. For all but the maximum, there are two angles that give the same range. Considering factors that might affect the ability of an archer to hit a target, such as wind, explain why the smaller angle (closer to the horizontal) is preferable. When would it be necessary for the archer to use the larger angle? Why does the punter in a football game use the higher trajectory?

4. During a lecture demonstration, a professor places two coins on the edge of a table. She then flicks one of the coins horizontally off the table, simultaneously nudging the other over the edge. Describe the subsequent motion of the two coins, in particular discussing whether they hit the floor at the same time.

Problems & Exercises

1. A projectile is launched at ground level with an initial speed of 50.0 m/s at an angle of 30.0º above the horizontal. It strikes a target above the ground 3.00 seconds later. What are the x and y distances from where the projectile was launched to where it lands?

2. A ball is kicked with an initial velocity of 16 m/s in the horizontal direction and 12 m/s in the vertical direction. (a) At what speed does the ball hit the ground? (b) For how long does the ball remain in the air? (c)What maximum height is attained by the ball?

3. A ball is thrown horizontally from the top of a 60.0-m building and lands 100.0 m from the base of the building. Ignore air resistance. (a) How long is the ball in the air? (b) What must have been the initial horizontal component of the velocity? (c) What is the vertical component of the velocity just before the ball hits the ground? (d) What is the velocity (including both the horizontal and vertical components) of the ball just before it hits the ground?

4. (a) A daredevil is attempting to jump his motorcycle over a line of buses parked end to end by driving up a 32º ramp at a speed of 40.0 m/s (144 km/h). How many buses can he clear if the top of the takeoff ramp is at the same height as the bus tops and the buses are 20.0 m long? (b) Discuss what your answer implies about the margin of error in this act—that is, consider how much greater the range is than the horizontal distance he must travel to miss the end of the last bus. (Neglect air resistance.)

5. An archer shoots an arrow at a 75.0 m distant target; the bull’s-eye of the target is at same height as the release height of the arrow. (a) At what angle must the arrow be released to hit the bull’s-eye if its initial speed is 35.0 m/s? In this part of the problem, explicitly show how you follow the steps involved in solving projectile motion problems. (b) There is a large tree halfway between the archer and the target with an overhanging horizontal branch 3.50 m above the release height of the arrow. Will the arrow go over or under the branch?

6. A rugby player passes the ball 7.00 m across the field, where it is caught at the same height as it left his hand. (a) At what angle was the ball thrown if its initial speed was 12.0 m/s, assuming that the smaller of the two possible angles was used? (b) What other angle gives the same range, and why would it not be used? (c) How long did this pass take?

7. Verify the ranges for the projectiles in Figure 5 (a) for θ = 45º and the given initial velocities.

8. Verify the ranges shown for the projectiles in Figure 5(b) for an initial velocity of 50 m/s at the given initial angles.

9. The cannon on a battleship can fire a shell a maximum distance of 32.0 km. (a) Calculate the initial velocity of the shell. (b) What maximum height does it reach? (At its highest, the shell is above 60% of the atmosphere—but air resistance is not really negligible as assumed to make this problem easier.) (c) The ocean is not flat, because the Earth is curved. Assume that the radius of the Earth is 6.37 × 10 3 . How many meters lower will its surface be 32.0 km from the ship along a horizontal line parallel to the surface at the ship? Does your answer imply that error introduced by the assumption of a flat Earth in projectile motion is significant here?

10. An arrow is shot from a height of 1.5 m toward a cliff of height  H . It is shot with a velocity of 30 m/s at an angle of 60º above the horizontal. It lands on the top edge of the cliff 4.0 s later. (a) What is the height of the cliff? (b) What is the maximum height reached by the arrow along its trajectory? (c) What is the arrow’s impact speed just before hitting the cliff?

11. In the standing broad jump, one squats and then pushes off with the legs to see how far one can jump. Suppose the extension of the legs from the crouch position is 0.600 m and the acceleration achieved from this position is 1.25 times the acceleration due to gravity, g . How far can they jump? State your assumptions. (Increased range can be achieved by swinging the arms in the direction of the jump.)

12. The world long jump record is 8.95 m (Mike Powell, USA, 1991). Treated as a projectile, what is the maximum range obtainable by a person if he has a take-off speed of 9.5 m/s? State your assumptions.

13. Serving at a speed of 170 km/h, a tennis player hits the ball at a height of 2.5 m and an angle  θ  below the horizontal. The service line is 11.9 m from the net, which is 0.91 m high. What is the angle  θ such that the ball just crosses the net? Will the ball land in the service box, whose out line is 6.40 m from the net?

14. A football quarterback is moving straight backward at a speed of 2.00 m/s when he throws a pass to a player 18.0 m straight downfield. (a) If the ball is thrown at an angle of 25º relative to the ground and is caught at the same height as it is released, what is its initial speed relative to the ground? (b) How long does it take to get to the receiver? (c) What is its maximum height above its point of release?

15. Gun sights are adjusted to aim high to compensate for the effect of gravity, effectively making the gun accurate only for a specific range. (a) If a gun is sighted to hit targets that are at the same height as the gun and 100.0 m away, how low will the bullet hit if aimed directly at a target 150.0 m away? The muzzle velocity of the bullet is 275 m/s. (b) Discuss qualitatively how a larger muzzle velocity would affect this problem and what would be the effect of air resistance.

16. An eagle is flying horizontally at a speed of 3.00 m/s when the fish in her talons wiggles loose and falls into the lake 5.00 m below. Calculate the velocity of the fish relative to the water when it hits the water.

17. An owl is carrying a mouse to the chicks in its nest. Its position at that time is 4.00 m west and 12.0 m above the center of the 30.0 cm diameter nest. The owl is flying east at 3.50 m/s at an angle 30.0º below the horizontal when it accidentally drops the mouse. Is the owl lucky enough to have the mouse hit the nest? To answer this question, calculate the horizontal position of the mouse when it has fallen 12.0 m.

18. Suppose a soccer player kicks the ball from a distance 30 m toward the goal. Find the initial speed of the ball if it just passes over the goal, 2.4 m above the ground, given the initial direction to be 40º above the horizontal.

19. Can a goalkeeper at her/ his goal kick a soccer ball into the opponent’s goal without the ball touching the ground? The distance will be about 95 m. A goalkeeper can give the ball a speed of 30 m/s.

20. The free throw line in basketball is 4.57 m (15 ft) from the basket, which is 3.05 m (10 ft) above the floor. A player standing on the free throw line throws the ball with an initial speed of 7.15 m/s, releasing it at a height of 2.44 m (8 ft) above the floor. At what angle above the horizontal must the ball be thrown to exactly hit the basket? Note that most players will use a large initial angle rather than a flat shot because it allows for a larger margin of error. Explicitly show how you follow the steps involved in solving projectile motion problems.

21. In 2007, Michael Carter (U.S.) set a world record in the shot put with a throw of 24.77 m. What was the initial speed of the shot if he released it at a height of 2.10 m and threw it at an angle of 38.0º above the horizontal? (Although the maximum distance for a projectile on level ground is achieved at 45º  when air resistance is neglected, the actual angle to achieve maximum range is smaller; thus, 38º  will give a longer range than 45º  in the shot put.)

22. A basketball player is running at 5.00 m/s directly toward the basket when he jumps into the air to dunk the ball. He maintains his horizontal velocity. (a) What vertical velocity does he need to rise 0.750 m above the floor? (b) How far from the basket (measured in the horizontal direction) must he start his jump to reach his maximum height at the same time as he reaches the basket?

23. A football player punts the ball at a 45º angle. Without an effect from the wind, the ball would travel 60.0 m horizontally. (a) What is the initial speed of the ball? (b) When the ball is near its maximum height it experiences a brief gust of wind that reduces its horizontal velocity by 1.50 m/s. What distance does the ball travel horizontally?

24. Prove that the trajectory of a projectile is parabolic, having the form [latex]y=\text{ax}+{\text{bx}}^{2}\\[/latex]. To obtain this expression, solve the equation [latex]x={v}_{0x}t\\[/latex] for t  and substitute it into the expression for [latex]y={v}_{0y}t-\left(1/2\right){\text{gt}}^{2}\\[/latex]. (These equations describe the x  and y positions of a projectile that starts at the origin.) You should obtain an equation of the form [latex]y=\text{ax}+{\text{bx}}^{2}\\[/latex] where a and b are constants.

25. Derive [latex]R=\frac{{{v}_{0}}^{2}\text{\sin}{2\theta }_{0}}{g}\\[/latex] for the range of a projectile on level ground by finding the time t at which y becomes zero and substituting this value of t  into the expression for x – x 0 , noting that R = x – x 0 .

26.  Unreasonable Results (a) Find the maximum range of a super cannon that has a muzzle velocity of 4.0 km/s. (b) What is unreasonable about the range you found? (c) Is the premise unreasonable or is the available equation inapplicable? Explain your answer. (d) If such a muzzle velocity could be obtained, discuss the effects of air resistance, thinning air with altitude, and the curvature of the Earth on the range of the super cannon.

27. Construct Your Own Problem Consider a ball tossed over a fence. Construct a problem in which you calculate the ball’s needed initial velocity to just clear the fence. Among the things to determine are; the height of the fence, the distance to the fence from the point of release of the ball, and the height at which the ball is released. You should also consider whether it is possible to choose the initial speed for the ball and just calculate the angle at which it is thrown. Also examine the possibility of multiple solutions given the distances and heights you have chosen.

Selected Solutions to Problems & Exercises

1.  x = 1.30 m × 10 2 , y = 30.9 m

3. (a) 3.50 s (b) 28.6 m/s (c) 34.3 m/s (d) 44.7 m/s, 50.2º below horizontal

5. (a) 18.4º (b) The arrow will go over the branch.

7. [latex]R=\frac{{{{v}_{0}}}^{}}{\sin{2\theta }_{0}g}\\[/latex]

For θ = 45º, [latex]R=\frac{{{{v}_{0}}}^{2}}{g}\\[/latex]

R = 91.9 m for v 0  = 30 m/s; R = 163 m for v 0 ; R = 255 m for v 0 = 50 m/s

9. (a) 560 m/s (b) 800 × 10 3 m  (c) 80.0 m. This error is not significant because it is only 1% of the answer in part (b).

11. 1.50 m, assuming launch angle of 45º

13.  θ  =6.1º. Yes, the ball lands at 5.3 m from the net

15. (a) −0.486 m (b) The larger the muzzle velocity, the smaller the deviation in the vertical direction, because the time of flight would be smaller. Air resistance would have the effect of decreasing the time of flight, therefore increasing the vertical deviation.

17. 4.23 m. No, the owl is not lucky; he misses the nest.

19. No, the maximum range (neglecting air resistance) is about 92 m.

21. 15.0 m/s

23. (a) 24.2 m/s (b) The ball travels a total of 57.4 m with the brief gust of wind.

25. [latex]y-{y}_{0}=0={v}_{0y}t-\frac{1}{2}{gt}^{2}=\left({v}_{0}\sin\theta\right)t-\frac{1}{2}{gt}^{2}\\[/latex] ,

so that [latex]t=\frac{2\left({v}_{0}\sin\theta \right)}{g}\\[/latex]

[latex]x-{x}_{0}={v}_{0x}t=\left({v}_{0}\cos\theta \right)t=R\\[/latex], and substituting for t  gives:

[latex]R={v}_{0}\cos\theta \left(\frac{{2v}_{0}\sin\theta}{g}\right)=\frac{{{2v}_{0}}^{2}\sin\theta \cos\theta }{g}\\[/latex]

since [latex]2\sin\theta \cos\theta =\sin 2\theta\\[/latex], the range is:

[latex]R=\frac{{{v}_{0}}^{2}\sin 2\theta }{g}\\[/latex].

• College Physics. Authored by : OpenStax College. Located at : http://cnx.org/contents/031da8d3-b525-429c-80cf-6c8ed997733a/College_Physics . License : CC BY: Attribution . License Terms : Located at License
• PhET Interactive Simulations . Authored by : University of Colorado Boulder . Located at : http://phet.colorado.edu . License : CC BY: Attribution

• TPC and eLearning
• What's NEW at TPC?
• Read Watch Interact
• Practice Review Test
• Teacher-Tools
• Subscription Selection
• Seat Calculator
• Ad Free Account
• Edit Profile Settings
• Classes (Version 2)
• Student Progress Edit
• Task Properties
• Export Student Progress
• Task, Activities, and Scores
• Metric Conversions Questions
• Metric System Questions
• Metric Estimation Questions
• Significant Digits Questions
• Proportional Reasoning
• Acceleration
• Distance-Displacement
• Dots and Graphs
• Graph That Motion
• Match That Graph
• Name That Motion
• Motion Diagrams
• Pos'n Time Graphs Numerical
• Pos'n Time Graphs Conceptual
• Up And Down - Questions
• Balanced vs. Unbalanced Forces
• Change of State
• Force and Motion
• Mass and Weight
• Match That Free-Body Diagram
• Net Force (and Acceleration) Ranking Tasks
• Newton's Second Law
• Normal Force Card Sort
• Recognizing Forces
• Air Resistance and Skydiving
• Solve It! with Newton's Second Law
• Which One Doesn't Belong?
• Component Addition Questions
• Head-to-Tail Vector Addition
• Projectile Mathematics
• Trajectory - Angle Launched Projectiles
• Trajectory - Horizontally Launched Projectiles
• Vector Addition
• Vector Direction
• Which One Doesn't Belong? Projectile Motion
• Forces in 2-Dimensions
• Being Impulsive About Momentum
• Explosions - Law Breakers
• Hit and Stick Collisions - Law Breakers
• Case Studies: Impulse and Force
• Impulse-Momentum Change Table
• Keeping Track of Momentum - Hit and Stick
• Keeping Track of Momentum - Hit and Bounce
• What's Up (and Down) with KE and PE?
• Energy Conservation Questions
• Energy Dissipation Questions
• Energy Ranking Tasks
• LOL Charts (a.k.a., Energy Bar Charts)
• Match That Bar Chart
• Words and Charts Questions
• Name That Energy
• Stepping Up with PE and KE Questions
• Case Studies - Circular Motion
• Circular Logic
• Forces and Free-Body Diagrams in Circular Motion
• Gravitational Field Strength
• Universal Gravitation
• Angular Position and Displacement
• Linear and Angular Velocity
• Angular Acceleration
• Rotational Inertia
• Balanced vs. Unbalanced Torques
• Getting a Handle on Torque
• Torque-ing About Rotation
• Properties of Matter
• Fluid Pressure
• Buoyant Force
• Sinking, Floating, and Hanging
• Pascal's Principle
• Flow Velocity
• Bernoulli's Principle
• Balloon Interactions
• Charge and Charging
• Charge Interactions
• Charging by Induction
• Conductors and Insulators
• Coulombs Law
• Electric Field
• Electric Field Intensity
• Polarization
• Case Studies: Electric Power
• Know Your Potential
• Light Bulb Anatomy
• I = ∆V/R Equations as a Guide to Thinking
• Parallel Circuits - ∆V = I•R Calculations
• Resistance Ranking Tasks
• Series Circuits - ∆V = I•R Calculations
• Series vs. Parallel Circuits
• Equivalent Resistance
• Period and Frequency of a Pendulum
• Pendulum Motion: Velocity and Force
• Energy of a Pendulum
• Period and Frequency of a Mass on a Spring
• Horizontal Springs: Velocity and Force
• Vertical Springs: Velocity and Force
• Energy of a Mass on a Spring
• Decibel Scale
• Frequency and Period
• Closed-End Air Columns
• Name That Harmonic: Strings
• Rocking the Boat
• Wave Basics
• Matching Pairs: Wave Characteristics
• Wave Interference
• Waves - Case Studies
• Color Addition and Subtraction
• Color Filters
• If This, Then That: Color Subtraction
• Light Intensity
• Color Pigments
• Converging Lenses
• Curved Mirror Images
• Law of Reflection
• Refraction and Lenses
• Total Internal Reflection
• Who Can See Who?
• Formulas and Atom Counting
• Atomic Models
• Bond Polarity
• Entropy Questions
• Cell Voltage Questions
• Heat of Formation Questions
• Reduction Potential Questions
• Oxidation States Questions
• Measuring the Quantity of Heat
• Hess's Law
• Oxidation-Reduction Questions
• Galvanic Cells Questions
• Thermal Stoichiometry
• Molecular Polarity
• Quantum Mechanics
• Balancing Chemical Equations
• Bronsted-Lowry Model of Acids and Bases
• Classification of Matter
• Collision Model of Reaction Rates
• Density Ranking Tasks
• Dissociation Reactions
• Complete Electron Configurations
• Elemental Measures
• Enthalpy Change Questions
• Equilibrium Concept
• Equilibrium Constant Expression
• Equilibrium Calculations - Questions
• Equilibrium ICE Table
• Intermolecular Forces Questions
• Ionic Bonding
• Lewis Electron Dot Structures
• Limiting Reactants
• Line Spectra Questions
• Mass Stoichiometry
• Measurement and Numbers
• Metals, Nonmetals, and Metalloids
• Metric Estimations
• Metric System
• Molarity Ranking Tasks
• Mole Conversions
• Name That Element
• Names to Formulas
• Names to Formulas 2
• Nuclear Decay
• Particles, Words, and Formulas
• Periodic Trends
• Precipitation Reactions and Net Ionic Equations
• Pressure Concepts
• Pressure-Temperature Gas Law
• Pressure-Volume Gas Law
• Chemical Reaction Types
• Significant Digits and Measurement
• States Of Matter Exercise
• Stoichiometry Law Breakers
• Stoichiometry - Math Relationships
• Subatomic Particles
• Spontaneity and Driving Forces
• Gibbs Free Energy
• Volume-Temperature Gas Law
• Acid-Base Properties
• Energy and Chemical Reactions
• Chemical and Physical Properties
• Valence Shell Electron Pair Repulsion Theory
• Writing Balanced Chemical Equations
• Mission CG1
• Mission CG10
• Mission CG2
• Mission CG3
• Mission CG4
• Mission CG5
• Mission CG6
• Mission CG7
• Mission CG8
• Mission CG9
• Mission EC1
• Mission EC10
• Mission EC11
• Mission EC12
• Mission EC2
• Mission EC3
• Mission EC4
• Mission EC5
• Mission EC6
• Mission EC7
• Mission EC8
• Mission EC9
• Mission RL1
• Mission RL2
• Mission RL3
• Mission RL4
• Mission RL5
• Mission RL6
• Mission KG7
• Mission RL8
• Mission KG9
• Mission RL10
• Mission RL11
• Mission RM1
• Mission RM2
• Mission RM3
• Mission RM4
• Mission RM5
• Mission RM6
• Mission RM8
• Mission RM10
• Mission LC1
• Mission RM11
• Mission LC2
• Mission LC3
• Mission LC4
• Mission LC5
• Mission LC6
• Mission LC8
• Mission SM1
• Mission SM2
• Mission SM3
• Mission SM4
• Mission SM5
• Mission SM6
• Mission SM8
• Mission SM10
• Mission KG10
• Mission SM11
• Mission KG2
• Mission KG3
• Mission KG4
• Mission KG5
• Mission KG6
• Mission KG8
• Mission KG11
• Mission F2D1
• Mission F2D2
• Mission F2D3
• Mission F2D4
• Mission F2D5
• Mission F2D6
• Mission KC1
• Mission KC2
• Mission KC3
• Mission KC4
• Mission KC5
• Mission KC6
• Mission KC7
• Mission KC8
• Mission AAA
• Mission SM9
• Mission LC7
• Mission LC9
• Mission NL1
• Mission NL2
• Mission NL3
• Mission NL4
• Mission NL5
• Mission NL6
• Mission NL7
• Mission NL8
• Mission NL9
• Mission NL10
• Mission NL11
• Mission NL12
• Mission MC1
• Mission MC10
• Mission MC2
• Mission MC3
• Mission MC4
• Mission MC5
• Mission MC6
• Mission MC7
• Mission MC8
• Mission MC9
• Mission RM7
• Mission RM9
• Mission RL7
• Mission RL9
• Mission SM7
• Mission SE1
• Mission SE10
• Mission SE11
• Mission SE12
• Mission SE2
• Mission SE3
• Mission SE4
• Mission SE5
• Mission SE6
• Mission SE7
• Mission SE8
• Mission SE9
• Mission VP1
• Mission VP10
• Mission VP2
• Mission VP3
• Mission VP4
• Mission VP5
• Mission VP6
• Mission VP7
• Mission VP8
• Mission VP9
• Mission WM1
• Mission WM2
• Mission WM3
• Mission WM4
• Mission WM5
• Mission WM6
• Mission WM7
• Mission WM8
• Mission WE1
• Mission WE10
• Mission WE2
• Mission WE3
• Mission WE4
• Mission WE5
• Mission WE6
• Mission WE7
• Mission WE8
• Mission WE9
• Vector Walk Interactive
• Name That Motion Interactive
• Kinematic Graphing 1 Concept Checker
• Kinematic Graphing 2 Concept Checker
• Graph That Motion Interactive
• Two Stage Rocket Interactive
• Rocket Sled Concept Checker
• Force Concept Checker
• Free-Body Diagrams Concept Checker
• Free-Body Diagrams The Sequel Concept Checker
• Skydiving Concept Checker
• Elevator Ride Concept Checker
• Vector Addition Concept Checker
• Vector Walk in Two Dimensions Interactive
• Name That Vector Interactive
• River Boat Simulator Concept Checker
• Projectile Simulator 2 Concept Checker
• Projectile Simulator 3 Concept Checker
• Hit the Target Interactive
• Turd the Target 1 Interactive
• Turd the Target 2 Interactive
• Balance It Interactive
• Go For The Gold Interactive
• Egg Drop Concept Checker
• Fish Catch Concept Checker
• Exploding Carts Concept Checker
• Collision Carts - Inelastic Collisions Concept Checker
• Its All Uphill Concept Checker
• Stopping Distance Concept Checker
• Chart That Motion Interactive
• Roller Coaster Model Concept Checker
• Uniform Circular Motion Concept Checker
• Horizontal Circle Simulation Concept Checker
• Vertical Circle Simulation Concept Checker
• Race Track Concept Checker
• Gravitational Fields Concept Checker
• Orbital Motion Concept Checker
• Angular Acceleration Concept Checker
• Balance Beam Concept Checker
• Torque Balancer Concept Checker
• Aluminum Can Polarization Concept Checker
• Charging Concept Checker
• Name That Charge Simulation
• Coulomb's Law Concept Checker
• Electric Field Lines Concept Checker
• Put the Charge in the Goal Concept Checker
• Circuit Builder Concept Checker (Series Circuits)
• Circuit Builder Concept Checker (Parallel Circuits)
• Circuit Builder Concept Checker (∆V-I-R)
• Circuit Builder Concept Checker (Voltage Drop)
• Equivalent Resistance Interactive
• Pendulum Motion Simulation Concept Checker
• Mass on a Spring Simulation Concept Checker
• Particle Wave Simulation Concept Checker
• Boundary Behavior Simulation Concept Checker
• Slinky Wave Simulator Concept Checker
• Simple Wave Simulator Concept Checker
• Wave Addition Simulation Concept Checker
• Standing Wave Maker Simulation Concept Checker
• Color Addition Concept Checker
• Painting With CMY Concept Checker
• Stage Lighting Concept Checker
• Filtering Away Concept Checker
• InterferencePatterns Concept Checker
• Young's Experiment Interactive
• Plane Mirror Images Interactive
• Who Can See Who Concept Checker
• Optics Bench (Mirrors) Concept Checker
• Name That Image (Mirrors) Interactive
• Refraction Concept Checker
• Total Internal Reflection Concept Checker
• Optics Bench (Lenses) Concept Checker
• Kinematics Preview
• Velocity Time Graphs Preview
• Moving Cart on an Inclined Plane Preview
• Stopping Distance Preview
• Cart, Bricks, and Bands Preview
• Fan Cart Study Preview
• Friction Preview
• Coffee Filter Lab Preview
• Friction, Speed, and Stopping Distance Preview
• Up and Down Preview
• Projectile Range Preview
• Ballistics Preview
• Juggling Preview
• Marshmallow Launcher Preview
• Air Bag Safety Preview
• Colliding Carts Preview
• Collisions Preview
• Engineering Safer Helmets Preview
• Push the Plow Preview
• Its All Uphill Preview
• Energy on an Incline Preview
• Modeling Roller Coasters Preview
• Hot Wheels Stopping Distance Preview
• Ball Bat Collision Preview
• Energy in Fields Preview
• Weightlessness Training Preview
• Roller Coaster Loops Preview
• Universal Gravitation Preview
• Keplers Laws Preview
• Kepler's Third Law Preview
• Charge Interactions Preview
• Sticky Tape Experiments Preview
• Wire Gauge Preview
• Voltage, Current, and Resistance Preview
• Light Bulb Resistance Preview
• Series and Parallel Circuits Preview
• Thermal Equilibrium Preview
• Linear Expansion Preview
• Heating Curves Preview
• Electricity and Magnetism - Part 1 Preview
• Electricity and Magnetism - Part 2 Preview
• Vibrating Mass on a Spring Preview
• Period of a Pendulum Preview
• Wave Speed Preview
• Slinky-Experiments Preview
• Standing Waves in a Rope Preview
• Sound as a Pressure Wave Preview
• DeciBel Scale Preview
• DeciBels, Phons, and Sones Preview
• Sound of Music Preview
• Shedding Light on Light Bulbs Preview
• Models of Light Preview
• Electromagnetic Radiation Preview
• Electromagnetic Spectrum Preview
• EM Wave Communication Preview
• Digitized Data Preview
• Light Intensity Preview
• Concave Mirrors Preview
• Object Image Relations Preview
• Snells Law Preview
• Reflection vs. Transmission Preview
• Magnification Lab Preview
• Reactivity Preview
• Ions and the Periodic Table Preview
• Periodic Trends Preview
• Intermolecular Forces Preview
• Melting Points and Boiling Points Preview
• Bond Energy and Reactions Preview
• Reaction Rates Preview
• Ammonia Factory Preview
• Stoichiometry Preview
• Nuclear Chemistry Preview
• Gaining Teacher Access
• Tasks and Classes
• Tasks - Classic
• Subscription
• Subscription Locator
• 1-D Kinematics
• Newton's Laws
• Vectors - Motion and Forces in Two Dimensions
• Momentum and Its Conservation
• Work and Energy
• Circular Motion and Satellite Motion
• Thermal Physics
• Static Electricity
• Electric Circuits
• Vibrations and Waves
• Sound Waves and Music
• Light and Color
• Reflection and Mirrors
• About the Physics Interactives
• Task Tracker
• Usage Policy
• Newtons Laws
• Vectors and Projectiles
• Forces in 2D
• Momentum and Collisions
• Circular and Satellite Motion
• Balance and Rotation
• Electromagnetism
• Waves and Sound
• Atomic Physics
• Forces in Two Dimensions
• Work, Energy, and Power
• Circular Motion and Gravitation
• Sound Waves
• 1-Dimensional Kinematics
• Circular, Satellite, and Rotational Motion
• Einstein's Theory of Special Relativity
• Waves, Sound and Light
• QuickTime Movies
• About the Concept Builders
• Pricing For Schools
• Directions for Version 2
• Measurement and Units
• Relationships and Graphs
• Rotation and Balance
• Vibrational Motion
• Reflection and Refraction
• Teacher Accounts
• Task Tracker Directions
• Kinematic Concepts
• Kinematic Graphing
• Wave Motion
• Sound and Music
• About CalcPad
• 1D Kinematics
• Vectors and Forces in 2D
• Simple Harmonic Motion
• Rotational Kinematics
• Rotation and Torque
• Rotational Dynamics
• Electric Fields, Potential, and Capacitance
• Transient RC Circuits
• Light Waves
• Units and Measurement
• Stoichiometry
• Molarity and Solutions
• Thermal Chemistry
• Acids and Bases
• Kinetics and Equilibrium
• Solution Equilibria
• Oxidation-Reduction
• Nuclear Chemistry
• Newton's Laws of Motion
• Work and Energy Packet
• Static Electricity Review
• NGSS Alignments
• 1D-Kinematics
• Projectiles
• Circular Motion
• Magnetism and Electromagnetism
• Graphing Practice
• About the ACT
• ACT Preparation
• For Teachers
• Other Resources
• Solutions Guide
• Solutions Guide Digital Download
• Motion in One Dimension
• Work, Energy and Power
• Algebra Based Physics
• Other Tools
• Frequently Asked Questions
• Purchasing the Download
• Purchasing the CD
• Purchasing the Digital Download
• About the NGSS Corner
• NGSS Search
• Force and Motion DCIs - High School
• Energy DCIs - High School
• Wave Applications DCIs - High School
• Force and Motion PEs - High School
• Energy PEs - High School
• Wave Applications PEs - High School
• Crosscutting Concepts
• The Practices
• Physics Topics
• NGSS Corner: Activity List
• NGSS Corner: Infographics
• About the Toolkits
• Position-Velocity-Acceleration
• Position-Time Graphs
• Velocity-Time Graphs
• Newton's First Law
• Newton's Second Law
• Newton's Third Law
• Terminal Velocity
• Projectile Motion
• Forces in 2 Dimensions
• Impulse and Momentum Change
• Momentum Conservation
• Work-Energy Fundamentals
• Work-Energy Relationship
• Roller Coaster Physics
• Satellite Motion
• Electric Fields
• Circuit Concepts
• Series Circuits
• Parallel Circuits
• Describing-Waves
• Wave Behavior Toolkit
• Standing Wave Patterns
• Resonating Air Columns
• Wave Model of Light
• Plane Mirrors
• Curved Mirrors
• Teacher Guide
• Using Lab Notebooks
• Current Electricity
• Light Waves and Color
• Reflection and Ray Model of Light
• Refraction and Ray Model of Light
• Classes (Legacy Version)
• Teacher Resources
• Subscriptions

• Newton's Laws
• Einstein's Theory of Special Relativity
• About Concept Checkers
• School Pricing
• Newton's Laws of Motion
• Newton's First Law
• Newton's Third Law
• Horizontally Launched Projectile Problems
• What is a Projectile?
• Motion Characteristics of a Projectile
• Horizontal and Vertical Velocity
• Horizontal and Vertical Displacement
• Initial Velocity Components
• Non-Horizontally Launched Projectile Problems

There are two basic types of projectile problems that we will discuss in this course. While the general principles are the same for each type of problem, the approach will vary due to the fact the problems differ in terms of their initial conditions. The two types of problems are:

A projectile is launched with an initial horizontal velocity from an elevated position and follows a parabolic path to the ground. Predictable unknowns include the initial speed of the projectile, the initial height of the projectile, the time of flight, and the horizontal distance of the projectile.

Examples of this type of problem are

• A pool ball leaves a 0.60-meter high table with an initial horizontal velocity of 2.4 m/s. Predict the time required for the pool ball to fall to the ground and the horizontal distance between the table's edge and the ball's landing location.

A soccer ball is kicked horizontally off a 22.0-meter high hill and lands a distance of 35.0 meters from the edge of the hill. Determine the initial horizontal velocity of the soccer ball.

A projectile is launched at an angle to the horizontal and rises upwards to a peak while moving horizontally. Upon reaching the peak, the projectile falls with a motion that is symmetrical to its path upwards to the peak. Predictable unknowns include the time of flight, the horizontal range, and the height of the projectile when it is at its peak.

• A football is kicked with an initial velocity of 25 m/s at an angle of 45-degrees with the horizontal. Determine the time of flight, the horizontal distance, and the peak height of the football.
• A long jumper leaves the ground with an initial velocity of 12 m/s at an angle of 28-degrees above the horizontal. Determine the time of flight, the horizontal distance, and the peak height of the long-jumper.

The second problem type will be the subject of the next part of Lesson 2 . In this part of Lesson 2, we will focus on the first type of problem - sometimes referred to as horizontally launched projectile problems. Three common kinematic equations that will be used for both type of problems include the following:

d = v i •t + 0.5*a*t 2 v f = v i + a•t v f 2  = v i 2  + 2*a•d  

Equations for the Horizontal Motion of a Projectile

The above equations work well for motion in one-dimension, but a projectile is usually moving in two dimensions - both horizontally and vertically. Since these two components of motion are independent of each other, two distinctly separate sets of equations are needed - one for the projectile's horizontal motion and one for its vertical motion. Thus, the three equations above are transformed into two sets of three equations. For the horizontal components of motion, the equations are

x = v i x •t + 0.5*a x *t 2

v f x  = v i x  + a x •t

v f x 2  = v i x 2  + 2*a x •x

Of these three equations, the top equation is the most commonly used. An application of projectile concepts to each of these equations would also lead one to conclude that any term with a x in it would cancel out of the equation since a x = 0 m/s/s . Once this cancellation of ax terms is performed, the only equation of usefulness is:

x = v i x •t

Equations for the Vertical Motion of a Projectile

For the vertical components of motion, the three equations are

y = v iy •t + 0.5*a y *t 2

v fy  = v iy  + a y •t

v fy 2  = v iy 2  + 2*a y •y

In each of the above equations, the vertical acceleration of a projectile is known to be -9.8 m/s/s (the acceleration of gravity). Furthermore, for the special case of the first type of problem (horizontally launched projectile problems), v iy = 0 m/s. Thus, any term with v iy in it will cancel out of the equation.

The two sets of three equations above are the kinematic equations that will be used to solve projectile motion problems.

Solving Projectile Problems

To illustrate the usefulness of the above equations in making predictions about the motion of a projectile, consider the solution to the following problem.

The solution of this problem begins by equating the known or given values with the symbols of the kinematic equations - x, y, v ix , v iy , a x , a y , and t. Because horizontal and vertical information is used separately, it is a wise idea to organized the given information in two columns - one column for horizontal information and one column for vertical information. In this case, the following information is either given or implied in the problem statement:

As indicated in the table, the unknown quantity is the horizontal displacement (and the time of flight) of the pool ball. The solution of the problem now requires the selection of an appropriate strategy for using the kinematic equations and the known information to solve for the unknown quantities. It will almost always be the case that such a strategy demands that one of the vertical equations be used to determine the time of flight of the projectile and then one of the horizontal equations be used to find the other unknown quantities (or vice versa - first use the horizontal and then the vertical equation). An organized listing of known quantities (as in the table above) provides cues for the selection of the strategy. For example, the table above reveals that there are three quantities known about the vertical motion of the pool ball. Since each equation has four variables in it, knowledge of three of the variables allows one to calculate a fourth variable. Thus, it would be reasonable that a vertical equation is used with the vertical values to determine time and then the horizontal equations be used to determine the horizontal displacement (x). The first vertical equation (y = v iy •t +0.5•a y •t 2 ) will allow for the determination of the time. Once the appropriate equation has been selected, the physics problem becomes transformed into an algebra problem. By substitution of known values, the equation takes the form of

Since the first term on the right side of the equation reduces to 0, the equation can be simplified to

If both sides of the equation are divided by -5.0 m/s/s, the equation becomes

By taking the square root of both sides of the equation, the time of flight can then be determined .

Once the time has been determined, a horizontal equation can be used to determine the horizontal displacement of the pool ball. Recall from the given information , v ix = 2.4 m/s and a x = 0 m/s/s. The first horizontal equation (x = v ix •t + 0.5•a x •t 2 ) can then be used to solve for "x." With the equation selected, the physics problem once more becomes transformed into an algebra problem. By substitution of known values, the equation takes the form of

Since the second term on the right side of the equation reduces to 0, the equation can then be simplified to

The answer to the stated problem is that the pool ball is in the air for 0.35 seconds and lands a horizontal distance of 0.84 m from the edge of the pool table.

The following procedure summarizes the above problem-solving approach.

• Carefully read the problem and list known and unknown information in terms of the symbols of the kinematic equations. For convenience sake, make a table with horizontal information on one side and vertical information on the other side.
• Identify the unknown quantity that the problem requests you to solve for.
• Select either a horizontal or vertical equation to solve for the time of flight of the projectile.
• With the time determined, use one of the other equations to solve for the unknown. (Usually, if a horizontal equation is used to solve for time, then a vertical equation can be used to solve for the final unknown quantity.)

One caution is in order. The sole reliance upon 4- and 5-step procedures to solve physics problems is always a dangerous approach. Physics problems are usually just that - problems! While problems can often be simplified by the use of short procedures as the one above, not all problems can be solved with the above procedure. While steps 1 and 2 above are critical to your success in solving horizontally launched projectile problems, there will always be a problem that doesn't fit the mold . Problem solving is not like cooking; it is not a mere matter of following a recipe. Rather, problem solving requires careful reading, a firm grasp of conceptual physics, critical thought and analysis, and lots of disciplined practice. Never divorce conceptual understanding and critical thinking from your approach to solving problems.

Check Your Understanding

Use y = v iy • t + 0.5 • a y • t 2 to solve for time; the time of flight is 2.12 seconds.

Now use x = v ix • t + 0.5 • a x • t 2 to solve for v ix

Note that a x is 0 m/s/s so the last term on the right side of the equation cancels. By substituting 35.0 m for x and 2.12 s for t, the v ix can be found to be 16.5 m/s.

We Would Like to Suggest ...

• Addition of Forces

• school Campus Bookshelves
• menu_book Bookshelves
• perm_media Learning Objects
• login Login
• how_to_reg Request Instructor Account
• hub Instructor Commons

Margin Size

• Download Page (PDF)
• Download Full Book (PDF)
• Periodic Table
• Physics Constants
• Scientific Calculator
• Reference & Cite
• Tools expand_more
• Readability

selected template will load here

This action is not available.

3.3: Projectile Motion

• Last updated
• Save as PDF
• Page ID 14447

\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)

\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)

\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)

( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)

\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)

\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)

\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)

\( \newcommand{\Span}{\mathrm{span}}\)

\( \newcommand{\id}{\mathrm{id}}\)

\( \newcommand{\kernel}{\mathrm{null}\,}\)

\( \newcommand{\range}{\mathrm{range}\,}\)

\( \newcommand{\RealPart}{\mathrm{Re}}\)

\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)

\( \newcommand{\Argument}{\mathrm{Arg}}\)

\( \newcommand{\norm}[1]{\| #1 \|}\)

\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)

\( \newcommand{\vectorA}[1]{\vec{#1}}      % arrow\)

\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}}      % arrow\)

\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)

\( \newcommand{\vectorC}[1]{\textbf{#1}} \)

\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)

\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)

\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)

Basic Equations and Parabolic Path

Projectile motion is a form of motion where an object moves in parabolic path; the path that the object follows is called its trajectory.

learning objectives

• Assess the effect of angle and velocity on the trajectory of the projectile; derive maximum height using displacement

Projectile Motion

Projectile motion is a form of motion where an object moves in a bilaterally symmetrical, parabolic path. The path that the object follows is called its trajectory. Projectile motion only occurs when there is one force applied at the beginning on the trajectory, after which the only interference is from gravity. In a previous atom we discussed what the various components of an object in projectile motion are. In this atom we will discuss the basic equations that go along with them in the special case in which the projectile initial positions are null (i.e. \(\mathrm{x_0=0}\) and \(\mathrm{y_0=0}\) ).

Initial Velocity

The initial velocity can be expressed as x components and y components:

\[\begin{align} \mathrm{u_x} & \mathrm{=u⋅ \cos θ} \\ \mathrm{u_y} & \mathrm{=u⋅ \sin θ} \end{align}\]

In this equation, \(\mathrm{u}\) stands for initial velocity magnitude and \(θ\) refers to projectile angle.

Time of Flight

The time of flight of a projectile motion is the time from when the object is projected to the time it reaches the surface. As we discussed previously, \(\mathrm{T}\) depends on the initial velocity magnitude and the angle of the projectile:

\[\begin{align} \mathrm{T} & \mathrm{=\dfrac{2⋅u_y}{g} } \\ \mathrm{T} & \mathrm{=\dfrac{2⋅u⋅\sin ⁡θ}{g}} \end{align}\]

Acceleration

In projectile motion, there is no acceleration in the horizontal direction. The acceleration, \(\mathrm{a}\), in the vertical direction is just due to gravity, also known as free fall:

\[\begin{align} \mathrm{a_x} & \mathrm{=0} \\ \mathrm{a_y} &\mathrm{=−g} \end{align}\]

The horizontal velocity remains constant, but the vertical velocity varies linearly, because the acceleration is constant. At any time, \(\mathrm{t}\), the velocity is:

\[\begin{align} \mathrm{u_x} & \mathrm{=u⋅ \cos θ} \\ \mathrm{u_y} & \mathrm{=u⋅ \sin θ−g⋅t} \end{align}\]

You can also use the Pythagorean Theorem to find velocity:

\[\mathrm{u=\sqrt{u_x^2+u_y^2}}\]

Displacement

At time, t, the displacement components are:

\[\begin{align} \mathrm{x} & \mathrm{=u⋅t⋅ \cos ⁡θ} \\ \mathrm{y} & \mathrm{=u⋅t⋅ \sin ⁡θ−\dfrac{1}{2}gt^2} \end{align}\]

The equation for the magnitude of the displacement is \(\mathrm{Δr=\sqrt{x^2+y^2}}\).

Parabolic Trajectory

We can use the displacement equations in the x and y direction to obtain an equation for the parabolic form of a projectile motion:

\[\mathrm{y=\tan θ⋅x−\dfrac{g}{2⋅u^2⋅ \cos ^2 θ}⋅x^2}\]

Maximum Height

The maximum height is reached when \(\mathrm{v_y=0}\). Using this we can rearrange the velocity equation to find the time it will take for the object to reach maximum height

\[\mathrm{t_h=\dfrac{u⋅\sin θ}{g}}\]

where \(\mathrm{t_h}\) stands for the time it takes to reach maximum height. From the displacement equation we can find the maximum height

\[\mathrm{h=\dfrac{u^2⋅ \sin ^2 ⁡θ}{2⋅g}}\]

The range of the motion is fixed by the condition \(\mathrm{y=0}\). Using this we can rearrange the parabolic motion equation to find the range of the motion:

\[\mathrm{R=\dfrac{u^2⋅ \sin ⁡2 θ}{g}.}\]

Range of Trajectory : The range of a trajectory is shown in this figure.

Projectiles at an Angle : This video gives a clear and simple explanation of how to solve a problem on Projectiles Launched at an Angle. I try to go step by step through this difficult problem to layout how to solve it in a super clear way. 2D kinematic problems take time to solve, take notes on the order of how I solved it. Best wishes. Tune into my other videos for more help. Peace.

Solving Problems

In projectile motion, an object moves in parabolic path; the path the object follows is called its trajectory.

• Identify which components are essential in determining projectile motion of an object

We have previously discussed projectile motion and its key components and basic equations. Using that information, we can solve many problems involving projectile motion. Before we do this, let’s review some of the key factors that will go into this problem-solving.

What is Projectile Motion?

Projectile motion is when an object moves in a bilaterally symmetrical, parabolic path. The path that the object follows is called its trajectory. Projectile motion only occurs when there is one force applied at the beginning, after which the only influence on the trajectory is that of gravity.

What are the Key Components of Projectile Motion?

The key components that we need to remember in order to solve projectile motion problems are:

• Initial launch angle, \(\mathrm{θ}\)
• Initial velocity, \(\mathrm{u}\)
• Time of flight, \(\mathrm{T}\)
• Acceleration, \(\mathrm{a}\)
• Horizontal velocity, \(\mathrm{v_x}\)
• Vertical velocity, \(\mathrm{v_y}\)
• Displacement, \(\mathrm{d}\)
• Maximum height, \(\mathrm{H}\)
• Range, \(\mathrm{R}\)

How To Solve Any Projectile Motion Problem (The Toolbox Method) : Introducing the “Toolbox” method of solving projectile motion problems! Here we use kinematic equations and modify with initial conditions to generate a “toolbox” of equations with which to solve a classic three-part projectile motion problem.

Now, let’s look at two examples of problems involving projectile motion.

Example \(\PageIndex{1}\):

Let’s say you are given an object that needs to clear two posts of equal height separated by a specific distance. Refer to for this example. The projectile is thrown at \(\mathrm{25 \sqrt{2}}\) m/s at an angle of 45°. If the object is to clear both posts, each with a height of 30m, find the minimum: (a) position of the launch on the ground in relation to the posts and (b) the separation between the posts. For simplicity’s sake, use a gravity constant of 10. Problems of any type in physics are much easier to solve if you list the things that you know (the “givens”).

Diagram for Example 1 : Use this figure as a reference to solve example 1. The problem is to make sure the object is able to clear both posts.

Solution: The first thing we need to do is figure out at what time tt the object reaches the specified height. Since the motion is in a parabolic shape, this will occur twice: once when traveling upward, and again when the object is traveling downward. For this we can use the equation of displacement in the vertical direction, \(\mathrm{y−y_0}\) :

\[\mathrm{y−y_0=(v_y⋅t)−(\dfrac{1}{2}⋅g⋅t^2)}\]

We substitute in the appropriate variables:

\[\mathrm{v_y=u⋅ \sin θ=25 \sqrt{2} \dfrac{m}{s}⋅ \sin 45^{\circ}=25 \dfrac{m}{s}}\]

\[\mathrm{30m=25⋅t−\dfrac{1}{2}⋅10⋅t^2}\]

We can use the quadratic equation to find that the roots of this equation are 2s and 3s. This means that the projectile will reach 30m after 2s, on its way up, and after 3s, on its way down.

An object is launched from the base of an incline, which is at an angle of 30°. If the launch angle is 60° from the horizontal and the launch speed is 10 m/s, what is the total flight time? The following information is given: \(\mathrm{u=10 \frac{m}{s}; θ=60°; g=10 \frac{m}{s^2}}\).

Diagram for Example 2 : When dealing with an object in projectile motion on an incline, we first need to use the given information to reorient the coordinate system in order to have the object launch and fall on the same surface.

Solution: In order to account for the incline angle, we have to reorient the coordinate system so that the points of projection and return are on the same level. The angle of projection with respect to the \(\mathrm{x}\) direction is \(\mathrm{θ−α}\), and the acceleration in the \(\mathrm{y}\) direction is \(\mathrm{g⋅ \cos ⁡α}\). We replace \(\mathrm{θ}\) with \(\mathrm{θ−α}\) and \(\mathrm{g}\) with \(\mathrm{g⋅ \cos ⁡α}\):

\[\begin{align} \mathrm{T \; } & \mathrm{=\dfrac{2⋅u⋅ \sin (θ)}{g} = \dfrac{2⋅u⋅\sin (θ−α)}{g⋅ \cos (α)}=\dfrac{2⋅10⋅ \sin (60−30)}{10⋅\cos (30)} =\dfrac{20⋅ \sin (30)}{10⋅ \cos (30)} } \\ \mathrm{T \;} &\mathrm{=\dfrac{2}{\sqrt{3}}s} \end{align}\]

Zero Launch Angle

An object launched horizontally at a height \(\mathrm{H}\) travels a range \(\mathrm{v_0\sqrt{\frac{2H}{g}}}\) during a time of flight \(\mathrm{T=\sqrt{\frac{2H}{g}}}\).

• Explain the relationship between the range and the time of flight

Projectile motion is a form of motion where an object moves in a parabolic path. The path followed by the object is called its trajectory. Projectile motion occurs when a force is applied at the beginning of the trajectory for the launch (after this the projectile is subject only to the gravity).

One of the key components of the projectile motion, and the trajectory it follows, is the initial launch angle . The angle at which the object is launched dictates the range, height, and time of flight the object will experience while in projectile motion. shows different paths for the same object being launched at the same initial velocity and different launch angles. As illustrated by the figure, the larger the initial launch angle and maximum height, the longer the flight time of the object.

Projectile Trajectories : The launch angle determines the range and maximum height that an object will experience after being launched.This image shows that path of the same object being launched at the same speed but different angles.

We have previously discussed the effects of different launch angles on range, height, and time of flight. However, what happens if there is no angle, and the object is just launched horizontally? It makes sense that the object should be launched at a certain height (\(\mathrm{H}\)), otherwise it wouldn’t travel very far before hitting the ground. Let’s examine how an object launched horizontally at a height \(\mathrm{H}\) travels. In our case is when \(\mathrm{α}\) is 0.

Projectile motion : Projectile moving following a parabola.Initial launch angle is αα, and the velocity is \(\mathrm{v_0}\).

Duration of Flight

There is no vertical component in the initial velocity (\(\mathrm{v_0}\)) because the object is launched horizontally. Since the object travels distance \(\mathrm{H}\) in the vertical direction before it hits the ground, we can use the kinematic equation for the vertical motion:

\[\mathrm{(y−y_0)=−H=0⋅T−\dfrac{1}{2}gT^2}\]

Here, \(\mathrm{T}\) is the duration of the flight before the object its the ground. Therefore:

\[\mathrm{T=\sqrt{\dfrac{2H}{g}}}\]

In the horizontal direction, the object travels at a constant speed \(\mathrm{v_0}\) during the flight. Therefore, the range \(\mathrm{R}\) (in the horizontal direction) is given as:

\[\mathrm{R=v_0⋅T=v_0\sqrt{\dfrac{2H}{g}}}\]

General Launch Angle

The initial launch angle (0-90 degrees) of an object in projectile motion dictates the range, height, and time of flight of that object.

• Choose the appropriate equation to find range, maximum height, and time of flight

Projectile motion is a form of motion where an object moves in a bilaterally symmetrical, parabolic path. The path that the object follows is called its trajectory. Projectile motion only occurs when there is one force applied at the beginning of the trajectory, after which the only interference is from gravity.

One of the key components of projectile motion and the trajectory that it follows is the initial launch angle. This angle can be anywhere from 0 to 90 degrees. The angle at which the object is launched dictates the range, height, and time of flight it will experience while in projectile motion. shows different paths for the same object launched at the same initial velocity at different launch angles. As you can see from the figure, the larger the initial launch angle, the closer the object comes to maximum height and the longer the flight time. The largest range will be experienced at a launch angle up to 45 degrees.

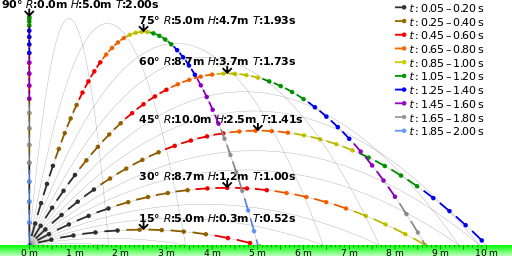
Launch Angle : The launch angle determines the range and maximum height that an object will experience after being launched. This image shows that path of the same object being launched at the same velocity but different angles.

The range, maximum height, and time of flight can be found if you know the initial launch angle and velocity, using the following equations:

\[\begin{align} \mathrm{R \;} & \mathrm{=\dfrac{v_i^2 \sin ^⁡2 θ_i}{g}} \\ \mathrm{h \;} & \mathrm{=\dfrac{v_i^2 \sin ^2 ⁡θ_i}{2g}} \\ \mathrm{T \;} & \mathrm{=\dfrac{2v_i \sin θ}{g}} \end{align}\]

Where R – Range, h – maximum height, T – time of flight, v i – initial velocity, θ i – initial launch angle, g – gravity.

Now that we understand how the launch angle plays a major role in many other components of the trajectory of an object in projectile motion, we can apply that knowledge to making an object land where we want it. If there is a certain distance, d, that you want your object to go and you know the initial velocity at which it will be launched, the initial launch angle required to get it that distance is called the angle of reach. It can be found using the following equation:

\[\mathrm{θ=\dfrac{1}{2} \sin ^{−1}(\dfrac{gd}{v^2})}\]

Key Points: Range, Symmetry, Maximum Height

Projectile motion is a form of motion where an object moves in parabolic path. The path that the object follows is called its trajectory.

• Construct a model of projectile motion by including time of flight, maximum height, and range

What is Projectile Motion ?

Projectile motion is a form of motion where an object moves in a bilaterally symmetrical, parabolic path. The path that the object follows is called its trajectory. Projectile motion only occurs when there is one force applied at the beginning on the trajectory, after which the only interference is from gravity. In this atom we are going to discuss what the various components of an object in projectile motion are, we will discuss the basic equations that go along with them in another atom, “Basic Equations and Parabolic Path”

Key Components of Projectile Motion:

Time of flight, t:.

The time of flight of a projectile motion is exactly what it sounds like. It is the time from when the object is projected to the time it reaches the surface. The time of flight depends on the initial velocity of the object and the angle of the projection, θθ. When the point of projection and point of return are on the same horizontal plane, the net vertical displacement of the object is zero.

All projectile motion happens in a bilaterally symmetrical path, as long as the point of projection and return occur along the same horizontal surface. Bilateral symmetry means that the motion is symmetrical in the vertical plane. If you were to draw a straight vertical line from the maximum height of the trajectory, it would mirror itself along this line.

Maximum Height, H:

The maximum height of a object in a projectile trajectory occurs when the vertical component of velocity, vyvy, equals zero. As the projectile moves upwards it goes against gravity, and therefore the velocity begins to decelerate. Eventually the vertical velocity will reach zero, and the projectile is accelerated downward under gravity immediately. Once the projectile reaches its maximum height, it begins to accelerate downward. This is also the point where you would draw a vertical line of symmetry.

Range of the Projectile, R:

The range of the projectile is the displacement in the horizontal direction. There is no acceleration in this direction since gravity only acts vertically. shows the line of range. Like time of flight and maximum height, the range of the projectile is a function of initial speed.

Range : The range of a projectile motion, as seen in this image, is independent of the forces of gravity.

• Objects that are projected from, and land on the same horizontal surface will have a vertically symmetrical path.
• The time it takes from an object to be projected and land is called the time of flight. This depends on the initial velocity of the projectile and the angle of projection.
• When the projectile reaches a vertical velocity of zero, this is the maximum height of the projectile and then gravity will take over and accelerate the object downward.
• The horizontal displacement of the projectile is called the range of the projectile, and depends on the initial velocity of the object.
• When solving problems involving projectile motion, we must remember all the key components of the motion and the basic equations that go along with them.
• Using that information, we can solve many different types of problems as long as we can analyze the information we are given and use the basic equations to figure it out.
• To clear two posts of equal height, and to figure out what the distance between these posts is, we need to remember that the trajectory is a parabolic shape and that there are two different times at which the object will reach the height of the posts.
• When dealing with an object in projectile motion on an incline, we first need to use the given information to reorientate the coordinate system in order to have the object launch and fall on the same surface.
• For the zero launch angle, there is no vertical component in the initial velocity.
• The duration of the flight before the object hits the ground is given as \(\mathrm{T=\sqrt{\frac{2H}{g}}}\).
• In the horizontal direction, the object travels at a constant speed v 0 during the flight. The range R (in the horizontal direction) is given as: \(\mathrm{R=v_0⋅T=v_0\sqrt{\dfrac{2H}{g}}}\).
• If the same object is launched at the same initial velocity, the height and time of flight will increase proportionally to the initial launch angle.
• An object launched into projectile motion will have an initial launch angle anywhere from 0 to 90 degrees.
• The range of an object, given the initial launch angle and initial velocity is found with: \(\mathrm{R=\dfrac{v_i^2 \sin ^⁡2 θ_i}{g}}\).
• The maximum height of an object, given the initial launch angle and initial velocity is found with: \(\mathrm{ｈ=\dfrac{v_i^2 \sin ^2 ⁡θ_i}{2g}}\).
• The time of flight of an object, given the initial launch angle and initial velocity is found with: \(\mathrm{T=\dfrac{2v_i \sin θ}{g}}\) .
• The angle of reach is the angle the object must be launched at in order to achieve a specific distance: \(\mathrm{θ=\dfrac{1}{2} \sin ^{−1}(\dfrac{gd}{v^2})}\).
• Objects that are projected from and land on the same horizontal surface will have a path symmetric about a vertical line through a point at the maximum height of the projectile.
• The time it takes from an object to be projected and land is called the time of flight. It depends on the initial velocity of the projectile and the angle of projection.
• The maximum height of the projectile is when the projectile reaches zero vertical velocity. From this point the vertical component of the velocity vector will point downwards.
• The horizontal displacement of the projectile is called the range of the projectile and depends on the initial velocity of the object.
• If an object is projected at the same initial speed, but two complementary angles of projection, the range of the projectile will be the same.
• trajectory : The path of a body as it travels through space.
• symmetrical : Exhibiting symmetry; having harmonious or proportionate arrangement of parts; having corresponding parts or relations.
• reorientate : to orientate anew; to cause to face a different direction
• gravity : Resultant force on Earth’s surface, of the attraction by the Earth’s masses, and the centrifugal pseudo-force caused by the Earth’s rotation.
• bilateral symmetry : the property of being symmetrical about a vertical plane

LICENSES AND ATTRIBUTIONS

CC LICENSED CONTENT, SHARED PREVIOUSLY

• Curation and Revision. Provided by : Boundless.com. License : CC BY-SA: Attribution-ShareAlike

CC LICENSED CONTENT, SPECIFIC ATTRIBUTION

• Sunil Kumar Singh, Features of Projectile Motion. September 18, 2013. Provided by : OpenStax CNX. Located at : http://cnx.org/content/m13847/latest/ . License : CC BY: Attribution
• Projectile motion. Provided by : Wikipedia. Located at : en.Wikipedia.org/wiki/Projectile_motion . License : CC BY-SA: Attribution-ShareAlike
• trajectory. Provided by : Wiktionary. Located at : en.wiktionary.org/wiki/trajectory . License : CC BY-SA: Attribution-ShareAlike
• symmetrical. Provided by : Wiktionary. Located at : en.wiktionary.org/wiki/symmetrical . License : CC BY-SA: Attribution-ShareAlike
• Projectiles at an Angle. Located at : http://www.youtube.com/watch?v=4jNE3eTVEgo . License : Public Domain: No Known Copyright . License Terms : Standard YouTube license
• Sunil Kumar Singh, Features of Projectile Motion. January 25, 2013. Provided by : OpenStax CNX. Located at : http://cnx.org/content/m13847/latest/ . License : CC BY: Attribution
• Sunil Kumar Singh, Projectile Motion on an Incline. September 18, 2013. Provided by : OpenStax CNX. Located at : http://cnx.org/content/m14614/latest/ . License : CC BY: Attribution
• reorientate. Provided by : Wiktionary. Located at : en.wiktionary.org/wiki/reorientate . License : CC BY-SA: Attribution-ShareAlike
• Sunil Kumar Singh, Projectile Motion on an Incline. February 2, 2013. Provided by : OpenStax CNX. Located at : http://cnx.org/content/m14614/latest/ . License : CC BY: Attribution
• How To Solve Any Projectile Motion Problem (The Toolbox Method). Located at : http://www.youtube.com/watch?v=M8xCj2VPHas . License : Public Domain: No Known Copyright . License Terms : Standard YouTube license
• Sunil Kumar Singh, Features of Projectile Motion. February 2, 2013. Provided by : OpenStax CNX. Located at : http://cnx.org/content/m13847/latest/ . License : CC BY: Attribution
• Trajectory of a projectile. Provided by : Wikipedia. Located at : en.Wikipedia.org/wiki/Trajectory_of_a_projectile . License : CC BY-SA: Attribution-ShareAlike
• Trajectory. Provided by : Wikipedia. Located at : en.Wikipedia.org/wiki/Trajectory . License : CC BY-SA: Attribution-ShareAlike
• Ideal projectile motion for different angles. Provided by : Wikipedia. Located at : en.Wikipedia.org/wiki/File:Ideal_projectile_motion_for_different_angles.svg . License : CC BY-SA: Attribution-ShareAlike
• Ferde hajitas1. Provided by : Wikipedia. Located at : en.Wikipedia.org/wiki/File:Ferde_hajitas1.svg . License : CC BY-SA: Attribution-ShareAlike
• Sunil Kumar Singh, Features of Projectile Motion. September 17, 2013. Provided by : OpenStax CNX. Located at : http://cnx.org/content/m13847/latest/ . License : CC BY: Attribution
• bilateral symmetry. Provided by : Wiktionary. Located at : en.wiktionary.org/wiki/bilateral_symmetry . License : CC BY-SA: Attribution-ShareAlike
• gravity. Provided by : Wiktionary. Located at : en.wiktionary.org/wiki/gravity . License : CC BY-SA: Attribution-ShareAlike
• trajectory. Provided by : Wiktionary. Located at : http://en.wiktionary.org/wiki/trajectory . License : CC BY-SA: Attribution-ShareAlike
• Ideal projectile motion for different angles. Provided by : Wikipedia. Located at : http://en.Wikipedia.org/wiki/File:Ideal_projectile_motion_for_different_angles.svg . License : CC BY-SA: Attribution-ShareAlike

• Mastering Projectile Motion: Physics Problem-Solving Made Easy

Welcome to Warren Institute! In today's article, we will delve into the fascinating world of Physics and explore how to solve projectile motion problems. Whether you're a student or a curious mind, understanding the principles behind projectile motion is crucial in grasping the fundamentals of Physics. With the help of step-by-step explanations and examples, we will demystify this concept and equip you with the necessary tools to solve any projectile motion problem that comes your way. So, let's dive in and unravel the secrets behind projectile motion in Physics!

Understanding Projectile Motion: An Introduction

Breaking down the components of projectile motion, applying mathematical formulas to solve projectile motion problems, tips and tricks for solving projectile motion problems, what are the key principles or formulas used to solve projectile motion problems in physics, how do you break down a projectile motion problem into its horizontal and vertical components for easier analysis, what are the common mistakes to avoid when solving projectile motion problems, how can i determine the initial velocity, angle, or time of flight in a projectile motion problem given certain variables, are there any specific strategies or techniques that can help improve my problem-solving skills in projectile motion.

In this section, we will provide a brief introduction to projectile motion in physics and explain its importance in mathematics education.

Projectile motion refers to the motion of an object that is launched into the air and moves along a curved path under the influence of gravity. It is an essential concept in physics as it helps us understand the motion of objects such as projectiles, rockets, or even sports balls.

Understanding projectile motion requires a solid understanding of kinematics, which is a branch of physics that deals with the motion of objects without considering the forces causing the motion. By studying projectile motion, students can enhance their problem-solving skills and gain a deeper understanding of mathematical concepts such as vectors, trigonometry , and calculus.

In this section, we will break down the different components of projectile motion and explain how they can be solved mathematically.

Projectile motion can be analyzed by breaking it down into two independent components: horizontal motion and vertical motion.

The horizontal motion remains constant throughout the entire trajectory, while the vertical motion is influenced by gravity. By understanding these two components, students can apply mathematical formulas and equations to solve complex projectile motion problems.

In this section, we will discuss the mathematical formulas and equations that can be used to solve projectile motion problems.

Key formulas in projectile motion include the range equation, the maximum height equation, and the time of flight equation. These formulas involve variables such as initial velocity, launch angle, time, and gravity.

By applying these formulas and equations, students can calculate various parameters of projectile motion, such as the maximum height reached, the time of flight, and the range of the projectile.

In this section, we will provide some tips and tricks to help students solve projectile motion problems more effectively.

Visualizing the problem, breaking it down into components, and utilizing trigonometric identities are some useful strategies when solving projectile motion problems. Additionally, practicing with a variety of problem types and seeking assistance from teachers or online resources can further enhance problem-solving skills in this area.

frequently asked questions

The key principles or formulas used to solve projectile motion problems in physics include Newton's laws of motion , kinematic equations , and trigonometric functions . These principles help determine the initial velocity, angle of projection, time of flight, maximum height, and range of a projectile.

To break down a projectile motion problem into its horizontal and vertical components for easier analysis, we can use trigonometry . We start by separating the initial velocity into its horizontal and vertical components using the sine and cosine functions. The horizontal component remains constant throughout the motion, while the vertical component is affected by gravity. By analyzing these components separately, we can solve for various parameters such as time of flight, maximum height, and range.

One common mistake to avoid when solving projectile motion problems is neglecting air resistance. In many cases, the effects of air resistance are small and can be ignored, but in some situations, it can significantly affect the motion of a projectile. Another mistake is assuming that the initial velocity is always horizontal or vertical. It's important to decompose the initial velocity into horizontal and vertical components correctly. Additionally, some students may forget to account for the acceleration due to gravity when calculating the vertical motion of the projectile. Lastly, overlooking the concept of time is another mistake to avoid. Make sure to consider the time of flight, which is the total time the projectile is in the air.

To determine the initial velocity, angle, or time of flight in a projectile motion problem, you can use equations of motion and basic trigonometry.

Initial Velocity: If the range and angle are known, you can use the equation R = (V^2 * sin(2θ))/g, where R is the range, V is the initial velocity, θ is the angle of projection, and g is the acceleration due to gravity. Solve for V to find the initial velocity.

Angle: If the initial velocity and range are known, you can use the equation tan(θ) = (gR)/(2V^2), where θ is the angle of projection, R is the range, V is the initial velocity, and g is the acceleration due to gravity. Solve for θ to find the angle.

Time of Flight: The time of flight can be determined using the equation T = (2V * sin(θ))/g, where T is the time of flight, V is the initial velocity, θ is the angle of projection, and g is the acceleration due to gravity.

By rearranging these equations and using the given variables, you can solve for the desired unknowns in the projectile motion problem.

Practice is key to improving problem-solving skills in projectile motion. It is important to work on a variety of problems that involve different scenarios and applications of the concept. Additionally, breaking down the problem into smaller steps and identifying relevant equations and variables can help in finding the solution. It is also beneficial to visualize the problem and draw diagrams to better understand the motion. Finally, seeking guidance from a teacher or tutor can provide valuable insights and feedback to further enhance problem-solving abilities in projectile motion.

In conclusion, understanding how to solve projectile motion problems in physics is crucial for students in their mathematics education. By mastering the concepts of displacement, velocity, and acceleration, as well as applying the appropriate formulas, students can accurately analyze the motion of projectiles. Additionally, recognizing the importance of breaking down the problem into horizontal and vertical components allows for a more systematic approach to solving projectile motion problems. Practicing these problem-solving techniques and honing mathematical skills will not only enhance students' understanding of physics but also contribute to their overall mathematical proficiency. So, let's launch our knowledge and project our abilities to excel in the fascinating world of projectile motion!

If you want to know other articles similar to Mastering Projectile Motion: Physics Problem-Solving Made Easy you can visit the category General Education .

Michaell Miller

Michael Miller is a passionate blog writer and advanced mathematics teacher with a deep understanding of mathematical physics. With years of teaching experience, Michael combines his love of mathematics with an exceptional ability to communicate complex concepts in an accessible way. His blog posts offer a unique and enriching perspective on mathematical and physical topics, making learning fascinating and understandable for all.

• Understanding Acids and Bases: A Basic Introduction to Organic Chemistry
• Exploring the Simple Cubic unit cell in Chemistry.

We Need Theorists and Researchers to Generate and Refine Innovative Strategies

Michelle's Creative Poster for an Engaging Class Presentation

Interactive Learning Activity: Drag the Appropriate Label to the Respective Target

Deciphering Stereochemical Configuration in Tetrahedral Centers: A Comprehensive Guide

Visualizing the End Result: Drawing the Final Product of Reaction Series

Drag Tiles to Boxes to Match Correct Pairs: Engaging Math Education Activity

• Science Notes Posts
• Contact Science Notes
• Todd Helmenstine Biography
• Anne Helmenstine Biography
• Free Printable Periodic Tables (PDF and PNG)
• Periodic Table Wallpapers
• Interactive Periodic Table
• Periodic Table Posters
• How to Grow Crystals
• Chemistry Projects
• Fire and Flames Projects
• Holiday Science
• Chemistry Problems With Answers
• Physics Problems
• Unit Conversion Example Problems
• Chemistry Worksheets
• Biology Worksheets
• Periodic Table Worksheets
• Physical Science Worksheets
• Science Lab Worksheets
• My Amazon Books

Projectile Motion Example Problem – Physics Homework Help 1

Throwing or shooting a projectile follows a parabolic course. If you know the initial velocity and angle of elevation of the projectile, you can find its time aloft, maximum height or range. You can also its altitude and distance travelled if given a time. This example problem shows how to do all of these.

Projectile Motion Example Problem: A cannon is fired with muzzle velocity of 150 m/s at an angle of elevation = 45°. Gravity = 9.8 m/s 2 . a) What is the maximum height the projectile reaches? b) What is the total time aloft? c) How far away did the projectile land? (Range) d) Where is the projectile at 10 seconds after firing?

Let’s set up what we know. First, let’s define our variables.

V 0 = initial velocity = muzzle velocity = 150 m/s v x = horizontal velocity component v y = vertical velocity component θ = angle of elevation = 45° h = maximum height R = range x = horizontal position at t=10 s y = vertical position at t=10 s m = mass of projectile g = acceleration due to gravity = 9.8 m/s 2

Part a) Find h.

The formulas we will be using are:

d = v 0 t + ½at 2

v f – v 0 = at

In order to find the distance h, we need to know two things: the velocity at h and the amount of time it takes to get there. The first is easy. The vertical component of the velocity is equal to zero at point h. This is the point where the upward motion is stopped and the projectile begins to fall back to Earth.

The initial vertical velocity is v 0y = v 0 ·sinθ v 0y = 150 m/s · sin(45°) v 0y = 106.1 m/s

Now we know the beginning and final velocity. The next thing we need is the acceleration.

The only force acting on the projectile is the force of gravity. Gravity has a magnitude of g and a direction in the negative y direction.

F = ma = -mg

solve for a

Now we have enough information to find the time. We know the initial vertical velocity (V 0y ) and the final vertical velocity at h (v hy = 0)

v hy – v 0y  = at 0 – v 0y = -9.8 m/s 2 ·t 0 – 106.1 m/s = -9.8 m/s 2 ·t

Solve for t

t = 10.8 s

Now solve the first equation for h

h = v 0y t + ½at 2 h = (106.1 m/s)(10.8 s) + ½(-9.8 m/s 2 )(10.8 s) 2 h = 1145.9 m – 571.5 m h = 574.4 m

The highest height the projectile reaches is 574.4 meters.

Part b: Find total time aloft.

We’ve already done most of the work to get this part of the question if you stop to think. The projectile’s trip can be broken into two parts: going up and coming down.

t total = t up + t down

The same acceleration force acts on the projectile in both directions. The time down takes the same amount of time it took to go up.

t up = t down

t total = 2 t up

we found t up in Part a of the problem: 10.8 seconds

t total = 2 (10.8 s) t total = 21.6 s

The total time aloft for the projectile is 21.6 seconds.

Part c: Find range R

To find the range, we need to know the initial velocity in the x direction.

v 0x = v 0 cosθ v 0x = 150 m/s·cos(45) v 0x = 106.1 m/s

To find the range R, use the equation:

R = v 0x t + ½at 2

There is no force acting along the x-axis. This means the acceleration in the x-direction is zero. The equation of motion is reduced to:

R = v 0x t + ½(0)t 2 R = v 0x t

The range is the point where the projectile strikes the ground which happens at the time we found in Part b of the problem.

R = 106.1 m/s · 21.6s R = 2291.8 m

The projectile landed 2291.8 meters from the canon.

Part d: Find the position at t = 10 seconds.

The position has two components: horizontal and vertical position. The horizontal position, x, is far downrange the projectile is after firing and the vertical component is the current altitude, y, of the projectile.

To find these positions, we will use the same equation:

First, let’s do the horizontal position. There is no acceleration in the horizontal direction so the second half of the equation is zero, just like in Part c.

We are given t = 10 seconds. V 0x was calculated in Part c of the problem.

x = 106.1 m/s · 10 s x = 1061 m

Now do the same thing for the vertical position.

y = v 0y t + ½at 2

We saw in Part b that v 0y = 109.6 m/s and a = -g = -9.8 m/s 2 . At t = 10 s:

y = 106.1 m/s · 10 s + ½(-9.8 m/s 2 )(10 s) 2 y = 1061 – 490 m y = 571 m

At t=10 seconds, the projectile is at (1061 m, 571 m) or 1061 m downrange and at an altitude of 571 meters.

If you need to know the velocity of the projectile at a specific time, you can use the formula

v – v 0  = at

and solve for v. Just remember velocity is a vector and will have both x and y components.

This specific example can be easily adapted for any initial velocity and any angle of elevation. If the cannon is fired on another planet with a different force of gravity, just change the value of g accordingly.

Related Posts

One thought on “ projectile motion example problem – physics homework help ”.

no you are not one who is correct

Comments are closed.

If you're seeing this message, it means we're having trouble loading external resources on our website.

If you're behind a web filter, please make sure that the domains *.kastatic.org and *.kasandbox.org are unblocked.

To log in and use all the features of Khan Academy, please enable JavaScript in your browser.

Physics library

Course: physics library   >   unit 2.

• Horizontally launched projectile

What is 2D projectile motion?

• Visualizing vectors in 2 dimensions
• Projectile at an angle
• Launching and landing on different elevations
• Total displacement for projectile
• 2D projectile motion: Identifying graphs for projectiles
• 2D projectile motion: Vectors and comparing multiple trajectories
• What are velocity components?
• Unit vectors and engineering notation
• Unit vector notation
• Unit vector notation (part 2)

What's a 2D projectile?

How do we handle 2d projectile motion mathematically, horizontal direction:, vertical direction:, what's confusing about 2d projectile motion, what do solved examples involving 2d projectile motion look like, example 1: horizontally launched water balloon, example 2: pumpkin launched at an angle, want to join the conversation.

• Upvote Button navigates to signup page
• Downvote Button navigates to signup page
• Flag Button navigates to signup page

Article Content

• AP Physics 1 | Beginner Guides
• 2D Motion , Projectile Motion
• Unit 1, Lesson 5 of 5

Unit 1.5 | Solving Projectile Motion (Motion in 2 Dimensions)

• 10 questions

Writer | Coach | USC - Physics B.S & Business B.A. | Fremont, CA

Unit 1 Breakdown

You are on Lesson 5 of 5

• Unit 1.1 | Understanding vectors and the Standard Units used in Physics
• Unit 1.2 | The Kinematic (motion) variables: Displacement, Velocity, and Acceleration
• Unit 1.3 | Graphing motion
• Unit 1.4 | Using Kinematic Equations in 1 Dimension
• Unit 1.5 | Projectile Motion: Using Kinematic Equations in 2 Dimensions [Current Lesson]

In this lesson you will learn: 

• The concepts behind projectile motion
• Breaking down velocity vectors into their components
• Labeling components and variables correctly
• Setting direction on the coordinate plane
• Framework projectile motion problems
• Solving several projectile motion problems step by step

LRN Projectile Motion

Watch this video first before moving on to the sections below.

Labeling components

As we saw in the video above it is important to keep track of direction of each variable.

For example, initial velocity in the horizontal direction should be written as v ox . Which means the “initial velocity in the x direction.”

Time is the only variable that does not have a direction.

Here is a chart of all their variables and their directions. Note, that this is purely for you to keep track of variables. You can switch them to whatever works best for you.

PS Solving Projectile Problems

In this video we will cover:

• The easiest way to solve any projectile problem (a framework)
• Solving an easy, medium, then hard projectile problem

Final Problem In Video

This is the final question given in the video above.

A spider crawling across a table leaps onto a magazine blocking its path. The initial velocity of the spider is 0.91 m/s at an angle of 40° above the table, and it lands on the magazine 0.08 seconds after leaving the table. Ignore air resistance. How thick is the magazine in mm?

Answer: 15.4 mm

To solve this: Make the x and y chart. In this case we have enough variables on the y-side, to solve for the vertical displacement (∆y aka the thickness of the book).

The most commonly made mistake here is setting direction. If we set down as negative, then velocity should be positive and gravity will be negative.

Plugging the y variables into the equation ∆y = v oy + 1/2at 2 will give a final answer of .00154 meters or 15.4 mm.

Recap and Framework

Let’s create a framework by breaking down all the steps we took above to solve a projectile motion problem:

• Draw a diagram
• Split any velocity vectors into x and y component
• Fill out kinematic variables on your x and y chart
• Identify what you’re solving for and see if you will use the x, y, or both sides of the chart to solve it
• Pick an equation and solve!

A more detailed outline of the projectile motion frame work can be found here .

Now you can attempt some questions on your own using the framework above.

PQ – Projectile Motion

These problems are much harder. Re-watch the video above to see the step by step process of solving ALL projectile motion problems.

• A ball is kicked horizontally at 8.0 m/s from a cliff 80m high. How far from the base of the cliff will the stone strike the ground? (32 m)
• A shell is fired from a cliff at an initial velocity of 800 m/s at a 30° angle below the horizontal. How long will it take to reach the ground 150m below? (.37 seconds)
• How far does he jump? (7.75 m)
• What is the maximum height he reaches? (.71 m)
• Determine the time taken by the projectile to hit point P at ground level. (5.5 seconds)
• Determine the range X of the projectile as measured from the of the cliff. (147 m)
• At the instant the projectile hits point P, find the horizontal and the vertical components of its velocity. (v x = 26.7 m/s; v y = -35.2 m/s)
• Find the speed of the projectile at point P. (44.2 m/s)
• How long is the bullet in the air? (35.4 seconds)
• What is the maximum height reached by the bullet? (4601 m)

For more practice, be sure to check out the course materials page .

The Next Unit – Unit 2 Preview

Congrats! You have officially completed the first Unit of AP Physics 1 and many other curriculums. You should be able to solve the most difficult kinematic problems with ease. For more practice be sure to check out the course materials page.

In the next unit (Unit 2: Forces Unpacked), we will a major Physics topic. You may have heard of Newton’s law and now you will be learning and applying it in depth. Further more, everything you have learned so far will also be applied!

Units in AP Physics 1

Unit 1 – Linear Kinematics

Unit 2 – Linear Forces

Unit 3 – Circular Motion

Unit 4 – Energy 

Unit 5 – Momentum 

Unit 6 – Torque 

Unit 7 – Oscillations 

Reading Key

Nerd-notes is free for everyone, help me to keep nerd-notes alive, unlocking the 5 secrets to scoring a 5 on the ap physics 1 exam, circular motion speed review, here is how 1000 ap physics students scored a 5 on ap physics 1, elite tutoring for physics.

• 1-to-1 sessions
• 1 program hours
• 3 spots left

5 Weeks for a 5 on the AP Physics 1 Exam

• 18 program hours
• 10 spots left

Prepare for High School Physics

• 20 program hours

NEW! PHY instantly solves any question

NEW! Elite Members get 40% off Physics Tutoring

Thanks for reading Nerd-Notes.

Login or create a FREE account to continue reading.

By continuing, you agree to the updated Terms of Sale , Terms of Use , and Privacy Policy .

We use site cookies to improve your experience. By continuing to browse on this website, you accept the use of cookies as outlined in our privacy policy .

Advertisement

How to Use the Mnemonic 'SOHCAHTOA' in Trigonometry

• Share Content on Facebook
• Share Content on LinkedIn
• Share Content on Flipboard
• Share Content on Reddit
• Share Content via Email

The mnemonic device SOHCAHTOA helps budding mathematicians remember the trigonometric functions sine (sin), cosine (cos) and tangent (tan), which they need to solve for triangles' missing sides and angles.

But to really understand how this memory tool is useful, it's necessary to first refresh yourself on the basics of right-angled triangles, which are the main focus in trigonometry.

What Are Right Triangles?

Trigonometric functions and ratios, using the trigonometric ratios, using the inverse trigonometric ratios, pythagorean theorem, solving for missing sides and angles, trigonometric identities, real-life applications.

A right-angled triangle, also known as a right triangle, has one angle that is exactly 90 degrees. The side opposite this right angle is the longest side, called the hypotenuse.

The other two sides are referred to as the opposite side and the adjacent side, depending on their relationship to the angle being measured or referenced.

Mathematicians need a way to solve for and relate the angles of a triangle to the length of its sides. To do this, they use the fundamental math functions called trigonometric functions , which have applications across science, engineering and everyday life.

Defined based on the ratios of the side lengths in a right-angled triangle, the trigonometric functions are:

• Sine (sin θ) : The ratio of the length of the opposite side to the hypotenuse (O/H).
• Cosine (cos θ) : The ratio of the length of the adjacent side to the hypotenuse (A/H).
• Tangent (tan θ) : The ratio of the length of the opposite side to the adjacent side (O/A).

These ratios depend on the angle θ, an acute angle (less than 90 degrees) in the triangle.

What Does SOHCAHTOA Stand for?

Here’s how the mnemonic device SOHCAHTOA helps mathematicians and math students remember the trigonometric functions and ratios.

• SOH : Sine = Opposite / Hypotenuse
• CAH : Cosine = Adjacent / Hypotenuse
• TOA : Tangent = Opposite / Adjacent

Mathematicians have to calculate the unknown side lengths or angles in a right triangle all the time. To do this, they apply the trigonometric functions.

For example, if you know the value of angle θ, you can find the two sides of a right-angled triangle.

Trigonometric Function Example

Suppose you have a right triangle with:

• Angle θ = 30 degrees
• Adjacent side = a = 5

You want to find the length of the opposite side b .

The tangent of an angle in a right-angled triangle is the ratio of the length of the opposite side to the length of the adjacent side, so:

You know that:

• Adjacent side a = 5

So, using the tangent function looks like this:

You know from trigonometric tables or by using a calculator that:

Now, to find b :

The length of the opposite side b is approximately 2.885 units.

If you know the lengths of two sides of a triangle, you can find the angle θ using the inverse trigonometric functions:

• sin -1 (also called arcsin) for sine
• cos -1 (also called arccos) for cosine
• tan -1 (also called arctan) for tangent

Inverse Trigonometric Function Example

• Adjacent side (to angle θ) = a = 5
• Opposite side (to angle θ) = b = 3

You want to find the angle θ.

To find the angle θ, you can use one of the inverse trigonometric functions :

Using a calculator, you can then determine:

In other words, the angle θ is approximately 30.96 degrees, making it also an acute angle.

Another essential concept in trigonometry is the Pythagorean theorem, which Pythagoras , a philosopher in ancient Greece devised. It states:

where a and b are the lengths of the legs (opposite and adjacent sides), and c is the hypotenuse.

The Pythagorean theorem is useful when you know the two sides of a right triangle and are trying to find a missing side.

To solve for missing sides, you can rearrange the trigonometric equations. For example:

Using a calculator, you can compute the sine, cosine or tangent of the angle θ, and then multiply by the known side length to find the unknown side.

Example Calculation

Consider a right-angled triangle where the hypotenuse is 10 units, and the angle θ is 30 degrees. To find the opposite side, you would use the sine function:

Since sin⁡30° =0.5:

Trig identities are equations involving trigonometric functions that are true for every value of the variable. They include fundamental identities like this basic Pythagorean identity:

Other common identities include angle sum and difference identities, double angle identities, half-angle identities, reciprocal identities and co-function identities. These identities are useful when you're trying to simplify trigonometric expressions and solving equations.

It's easy to view trigonometry as just a theoretical concept, but that's far from the case. It actually has practical applications across many different fields, including engineering, physics and astronomy, and even in everyday problem-solving scenarios like navigation and construction.

Engineers use trigonometric ratios to analyze forces in structures like bridges and buildings, or to design mechanical systems in engines . With these ratios, physicists can determine the trajectory, range and height of objects in projectile motion, and astronomers can calculate the necessary angles for precisely aligning their telescopes to specific celestial objects or events.

We created this article in conjunction with AI technology, then made sure it was fact-checked and edited by a HowStuffWorks editor.

Please copy/paste the following text to properly cite this HowStuffWorks.com article: