solving word problems involving operations

Reading & Math for K-5

• Kindergarten
• Learning numbers
• Comparing numbers
• Place Value
• Roman numerals
• Subtraction
• Multiplication
• Order of operations
• Drills & practice
• Measurement
• Factoring & prime factors
• Proportions
• Shape & geometry
• Data & graphing
• Word problems
• Children's stories
• Leveled Stories
• Context clues
• Cause & effect
• Compare & contrast
• Fact vs. fiction
• Fact vs. opinion
• Main idea & details
• Story elements
• Conclusions & inferences
• Sounds & phonics
• Words & vocabulary
• Reading comprehension
• Early writing
• Numbers & counting
• Simple math
• Social skills
• Other activities
• Dolch sight words
• Fry sight words
• Multiple meaning words
• Prefixes & suffixes
• Vocabulary cards
• Other parts of speech
• Punctuation
• Capitalization
• Narrative writing
• Opinion writing
• Informative writing
• Cursive alphabet
• Cursive letters
• Cursive letter joins
• Cursive words
• Cursive sentences
• Cursive passages
• Grammar & Writing

Breadcrumbs

• Word Problems
• 4 Operations

Download & Print From only $2.60

4 Operations word problems

Add / subtract / multiply / divide.

These grade 5 math word problems involve the 4 basic operations : addition, subtraction, multiplication and division. Some questions will have more than one step. The last question on each worksheet asks students to write an equation with a variable representing the unknown quantity.

These worksheets are available to members only.

Join K5 to save time, skip ads and access more content. Learn More

More word problem worksheets

Explore all of our math word problem worksheets , from kindergarten through grade 5.

What is K5?

K5 Learning offers free worksheets , flashcards  and inexpensive  workbooks  for kids in kindergarten to grade 5. Become a member  to access additional content and skip ads.

Our members helped us give away millions of worksheets last year.

We provide free educational materials to parents and teachers in over 100 countries. If you can, please consider purchasing a membership ($24/year) to support our efforts.

Members skip ads and access exclusive features.

Learn about member benefits

This content is available to members only.

• Forgot Password?

Math Word Problems

Welcome to the math word problems worksheets page at Math-Drills.com! On this page, you will find Math word and story problems worksheets with single- and multi-step solutions on a variety of math topics including addition, multiplication, subtraction, division and other math topics. It is usually a good idea to ensure students already have a strategy or two in place to complete the math operations involved in a particular question. For example, students may need a way to figure out what 7 × 8 is or have previously memorized the answer before you give them a word problem that involves finding the answer to 7 × 8.

There are a number of strategies used in solving math word problems; if you don't have a favorite, try the Math-Drills.com problem-solving strategy:

• Question : Understand what the question is asking. What operation or operations do you need to use to solve this question? Ask for help to understand the question if you can't do it on your own.
• Estimate : Use an estimation strategy, so you can check your answer for reasonableness in the evaluate step. Try underestimating and overestimating, so you know what range the answer is supposed to be in. Be flexible in rounding numbers if it will make your estimate easier.
• Strategize : Choose a strategy to solve the problem. Will you use mental math, manipulatives, or pencil and paper? Use a strategy that works for you. Save the calculator until the evaluate stage.
• Calculate : Use your strategy to solve the problem.
• Evaluate : Compare your answer to your estimate. If you under and overestimated, is the answer in the correct range. If you rounded up or down, does the answer make sense (e.g. is it a little less or a little more than the estimate). Also check with a calculator.

Most Popular Math Word Problems this Week

Arithmetic Word Problems

• Addition Word Problems One-Step Addition Word Problems Using Single-Digit Numbers One-Step Addition Word Problems Using Two-Digit Numbers
• Subtraction Word Problems Subtraction Facts Word Problems With Differences from 5 to 12
• Multiplication Word Problems One-Step Multiplication Word Problems up to 10 × 10
• Division Word Problems Division Facts Word Problems with Quotients from 5 to 12
• Multi-Step Word Problems Easy Multi-Step Word Problems

Copyright © 2005-2024 Math-Drills.com You may use the math worksheets on this website according to our Terms of Use to help students learn math.

If you're seeing this message, it means we're having trouble loading external resources on our website.

If you're behind a web filter, please make sure that the domains *.kastatic.org and *.kasandbox.org are unblocked.

To log in and use all the features of Khan Academy, please enable JavaScript in your browser.

Praxis Core Math

Course: praxis core math   >   unit 1.

• Algebraic properties | Lesson
• Algebraic properties | Worked example
• Solution procedures | Lesson
• Solution procedures | Worked example
• Equivalent expressions | Lesson
• Equivalent expressions | Worked example
• Creating expressions and equations | Lesson
• Creating expressions and equations | Worked example

Algebraic word problems | Lesson

• Algebraic word problems | Worked example
• Linear equations | Lesson
• Linear equations | Worked example
• Quadratic equations | Lesson
• Quadratic equations | Worked example

What are algebraic word problems?

What skills are needed.

• Translating sentences to equations
• Solving linear equations with one variable
• Evaluating algebraic expressions
• Solving problems using Venn diagrams

How do we solve algebraic word problems?

• Define a variable.
• Write an equation using the variable.
• Solve the equation.
• If the variable is not the answer to the word problem, use the variable to calculate the answer.

What's a Venn diagram?

• 7 + 10 − 13 = 4 ‍   brought both food and drinks.
• 7 − 4 = 3 ‍   brought only food.
• 10 − 4 = 6 ‍   brought only drinks.
• Your answer should be
• an integer, like 6 ‍  
• a simplified proper fraction, like 3 / 5 ‍  
• a simplified improper fraction, like 7 / 4 ‍  
• a mixed number, like 1   3 / 4 ‍  
• an exact decimal, like 0.75 ‍  
• a multiple of pi, like 12   pi ‍   or 2 / 3   pi ‍  
• (Choice A)   $ 4 ‍   A $ 4 ‍  
• (Choice B)   $ 5 ‍   B $ 5 ‍  
• (Choice C)   $ 9 ‍   C $ 9 ‍  
• (Choice D)   $ 14 ‍   D $ 14 ‍  
• (Choice E)   $ 20 ‍   E $ 20 ‍  
• (Choice A)   10 ‍   A 10 ‍  
• (Choice B)   12 ‍   B 12 ‍  
• (Choice C)   24 ‍   C 24 ‍  
• (Choice D)   30 ‍   D 30 ‍  
• (Choice E)   32 ‍   E 32 ‍  
• (Choice A)   4 ‍   A 4 ‍  
• (Choice B)   10 ‍   B 10 ‍  
• (Choice C)   14 ‍   C 14 ‍  
• (Choice D)   18 ‍   D 18 ‍  
• (Choice E)   22 ‍   E 22 ‍  

Things to remember

Want to join the conversation.

• Upvote Button navigates to signup page
• Downvote Button navigates to signup page
• Flag Button navigates to signup page

• Get started with computers
• Learn Microsoft Office
• Apply for a job
• Improve my work skills
• Design nice-looking docs
• Getting Started
• Smartphones & Tablets
• Typing Tutorial
• Online Learning
• Basic Internet Skills
• Online Safety
• Social Media
• Zoom Basics
• Google Docs
• Google Sheets
• Career Planning
• Resume Writing
• Cover Letters
• Job Search and Networking
• Business Communication
• Entrepreneurship 101
• Careers without College
• Job Hunt for Today
• 3D Printing
• Freelancing 101
• Personal Finance
• Sharing Economy
• Decision-Making
• Graphic Design
• Photography
• Image Editing
• Learning WordPress
• Language Learning
• Critical Thinking
• For Educators
• Translations
• Staff Picks
• English expand_more expand_less

Algebra Topics  - Introduction to Word Problems

Algebra topics  -, introduction to word problems, algebra topics introduction to word problems.

Algebra Topics: Introduction to Word Problems

Lesson 9: introduction to word problems.

/en/algebra-topics/solving-equations/content/

What are word problems?

A word problem is a math problem written out as a short story or scenario. Basically, it describes a realistic problem and asks you to imagine how you would solve it using math. If you've ever taken a math class, you've probably solved a word problem. For instance, does this sound familiar?

Johnny has 12 apples. If he gives four to Susie, how many will he have left?

You could solve this problem by looking at the numbers and figuring out what the problem is asking you to do. In this case, you're supposed to find out how many apples Johnny has left at the end of the problem. By reading the problem, you know Johnny starts out with 12 apples. By the end, he has 4 less because he gave them away. You could write this as:

12 - 4 = 8 , so you know Johnny has 8 apples left.

Word problems in algebra

If you were able to solve this problem, you should also be able to solve algebra word problems. Yes, they involve more complicated math, but they use the same basic problem-solving skills as simpler word problems.

You can tackle any word problem by following these five steps:

• Read through the problem carefully, and figure out what it's about.
• Represent unknown numbers with variables.
• Translate the rest of the problem into a mathematical expression.
• Solve the problem.
• Check your work.

We'll work through an algebra word problem using these steps. Here's a typical problem:

The rate to rent a small moving van is $30 per day, plus $0.50 per mile. Jada rented a van to drive to her new home. It took two days, and the van cost $360. How many miles did she drive?

It might seem complicated at first glance, but we already have all of the information we need to solve it. Let's go through it step by step.

Step 1: Read through the problem carefully.

With any problem, start by reading through the problem. As you're reading, consider:

• What question is the problem asking?
• What information do you already have?

Let's take a look at our problem again. What question is the problem asking? In other words, what are you trying to find out?

The rate to rent a small moving van is $30 per day, plus $0.50 per mile. Jada rented a van to drive to her new home. It took 2 days, and the van cost $360. How many miles did she drive?

There's only one question here. We're trying to find out how many miles Jada drove . Now we need to locate any information that will help us answer this question.

There are a few important things we know that will help us figure out the total mileage Jada drove:

• The van cost $30 per day.
• In addition to paying a daily charge, Jada paid $0.50 per mile.
• Jada had the van for 2 days.
• The total cost was $360 .

Step 2: Represent unknown numbers with variables.

In algebra, you represent unknown numbers with letters called variables . (To learn more about variables, see our lesson on reading algebraic expressions .) You can use a variable in the place of any amount you don't know. Looking at our problem, do you see a quantity we should represent with a variable? It's often the number we're trying to find out.

Since we're trying to find the total number of miles Jada drove, we'll represent that amount with a variable—at least until we know it. We'll use the variable m for miles . Of course, we could use any variable, but m should be easy to remember.

Step 3: Translate the rest of the problem.

Let's take another look at the problem, with the facts we'll use to solve it highlighted.

The rate to rent a small moving van is $30 per day , plus $0.50 per mile . Jada rented a van to drive to her new home. It took 2 days , and the van cost $360 . How many miles did she drive?

We know the total cost of the van, and we know that it includes a fee for the number of days, plus another fee for the number of miles. It's $30 per day, and $0.50 per mile. A simpler way to say this would be:

$30 per day plus $0.50 per mile is $360.

If you look at this sentence and the original problem, you can see that they basically say the same thing: It cost Jada $30 per day and $0.50 per mile, and her total cost was $360 . The shorter version will be easier to translate into a mathematical expression.

Let's start by translating $30 per day . To calculate the cost of something that costs a certain amount per day, you'd multiply the per-day cost by the number of days—in other words, 30 per day could be written as 30 ⋅ days, or 30 times the number of days . (Not sure why you'd translate it this way? Check out our lesson on writing algebraic expressions .)

$30 per day and $.50 per mile is $360

$30 ⋅ day + $.50 ⋅ mile = $360

As you can see, there were a few other words we could translate into operators, so and $.50 became + $.50 , $.50 per mile became $.50 ⋅ mile , and is became = .

Next, we'll add in the numbers and variables we already know. We already know the number of days Jada drove, 2 , so we can replace that. We've also already said we'll use m to represent the number of miles, so we can replace that too. We should also take the dollar signs off of the money amounts to make them consistent with the other numbers.

30 ⋅ 2 + .5 ⋅ m = 360

Now we have our expression. All that's left to do is solve it.

Step 4: Solve the problem.

This problem will take a few steps to solve. (If you're not sure how to do the math in this section, you might want to review our lesson on simplifying expressions .) First, let's simplify the expression as much as possible. We can multiply 30 and 2, so let's go ahead and do that. We can also write .5 ⋅ m as 0.5 m .

60 + .5m = 360

Next, we need to do what we can to get the m alone on the left side of the equals sign. Once we do that, we'll know what m is equal to—in other words, it will let us know the number of miles in our word problem.

We can start by getting rid of the 60 on the left side by subtracting it from both sides .

The only thing left to get rid of is .5 . Since it's being multiplied with m , we'll do the reverse and divide both sides of the equation with it.

.5 m / .5 is m and 300 / 0.50 is 600 , so m = 600 . In other words, the answer to our problem is 600 —we now know Jada drove 600 miles.

Step 5: Check the problem.

To make sure we solved the problem correctly, we should check our work. To do this, we can use the answer we just got— 600 —and calculate backward to find another of the quantities in our problem. In other words, if our answer for Jada's distance is correct, we should be able to use it to work backward and find another value, like the total cost. Let's take another look at the problem.

According to the problem, the van costs $30 per day and $0.50 per mile. If Jada really did drive 600 miles in 2 days, she could calculate the cost like this:

$30 per day and $0.50 per mile

30 ⋅ day + .5 ⋅ mile

30 ⋅ 2 + .5 ⋅ 600

According to our math, the van would cost $360, which is exactly what the problem says. This means our solution was correct. We're done!

While some word problems will be more complicated than others, you can use these basic steps to approach any word problem. On the next page, you can try it for yourself.

Let's practice with a couple more problems. You can solve these problems the same way we solved the first one—just follow the problem-solving steps we covered earlier. For your reference, these steps are:

If you get stuck, you might want to review the problem on page 1. You can also take a look at our lesson on writing algebraic expressions for some tips on translating written words into math.

Try completing this problem on your own. When you're done, move on to the next page to check your answer and see an explanation of the steps.

A single ticket to the fair costs $8. A family pass costs $25 more than half of that. How much does a family pass cost?

Here's another problem to do on your own. As with the last problem, you can find the answer and explanation to this one on the next page.

Flor and Mo both donated money to the same charity. Flor gave three times as much as Mo. Between the two of them, they donated $280. How much money did Mo give?

Problem 1 Answer

Here's Problem 1:

A single ticket to the fair costs $8. A family pass costs $25 more than half that. How much does a family pass cost?

Answer: $29

Let's solve this problem step by step. We'll solve it the same way we solved the problem on page 1.

Step 1: Read through the problem carefully

The first in solving any word problem is to find out what question the problem is asking you to solve and identify the information that will help you solve it . Let's look at the problem again. The question is right there in plain sight:

So is the information we'll need to answer the question:

• A single ticket costs $8 .
• The family pass costs $25 more than half the price of the single ticket.

Step 2: Represent the unknown numbers with variables

The unknown number in this problem is the cost of the family pass . We'll represent it with the variable f .

Step 3: Translate the rest of the problem

Let's look at the problem again. This time, the important facts are highlighted.

A single ticket to the fair costs $8 . A family pass costs $25 more than half that . How much does a family pass cost?

In other words, we could say that the cost of a family pass equals half of $8, plus $25 . To turn this into a problem we can solve, we'll have to translate it into math. Here's how:

• First, replace the cost of a family pass with our variable f .

f equals half of $8 plus $25

• Next, take out the dollar signs and replace words like plus and equals with operators.

f = half of 8 + 25

• Finally, translate the rest of the problem. Half of can be written as 1/2 times , or 1/2 ⋅ :

f = 1/2 ⋅ 8 + 25

Step 4: Solve the problem

Now all we have to do is solve our problem. Like with any problem, we can solve this one by following the order of operations.

• f is already alone on the left side of the equation, so all we have to do is calculate the right side.
• First, multiply 1/2 by 8 . 1/2 ⋅ 8 is 4 .
• Next, add 4 and 25. 4 + 25 equals 29 .

That's it! f is equal to 29. In other words, the cost of a family pass is $29 .

Step 5: Check your work

Finally, let's check our work by working backward from our answer. In this case, we should be able to correctly calculate the cost of a single ticket by using the cost we calculated for the family pass. Let's look at the original problem again.

We calculated that a family pass costs $29. Our problem says the pass costs $25 more than half the cost of a single ticket. In other words, half the cost of a single ticket will be $25 less than $29.

• We could translate this into this equation, with s standing for the cost of a single ticket.

1/2s = 29 - 25

• Let's work on the right side first. 29 - 25 is 4 .
• To find the value of s , we have to get it alone on the left side of the equation. This means getting rid of 1/2 . To do this, we'll multiply each side by the inverse of 1/2: 2 .

According to our math, s = 8 . In other words, if the family pass costs $29, the single ticket will cost $8. Looking at our original problem, that's correct!

So now we're sure about the answer to our problem: The cost of a family pass is $29 .

Number Line

• \mathrm{Lauren's\:age\:is\:half\:of\:Joe's\:age.\:Emma\:is\:four\:years\:older\:than\:Joe.\:The\:sum\:of\:Lauren,\:Emma,\:and\:Joe's\:age\:is\:54.\:How\:old\:is\:Joe?}
• \mathrm{Kira\:went\:for\:a\:drive\:in\:her\:new\:car.\:She\:drove\:for\:142.5\:miles\:at\:a\:speed\:of\:57\:mph.\:For\:how\:many\:hours\:did\:she\:drive?}
• \mathrm{The\:sum\:of\:two\:numbers\:is\:249\:.\:Twice\:the\:larger\:number\:plus\:three\:times\:the\:smaller\:number\:is\:591\:.\:Find\:the\:numbers.}
• \mathrm{If\:2\:tacos\:and\:3\:drinks\:cost\:12\:and\:3\:tacos\:and\:2\:drinks\:cost\:13\:how\:much\:does\:a\:taco\:cost?}
• \mathrm{You\:deposit\:3000\:in\:an\:account\:earning\:2\%\:interest\:compounded\:monthly.\:How\:much\:will\:you\:have\:in\:the\:account\:in\:15\:years?}
• How do you solve word problems?
• To solve word problems start by reading the problem carefully and understanding what it's asking. Try underlining or highlighting key information, such as numbers and key words that indicate what operation is needed to perform. Translate the problem into mathematical expressions or equations, and use the information and equations generated to solve for the answer.
• How do you identify word problems in math?
• Word problems in math can be identified by the use of language that describes a situation or scenario. Word problems often use words and phrases which indicate that performing calculations is needed to find a solution. Additionally, word problems will often include specific information such as numbers, measurements, and units that needed to be used to solve the problem.
• Is there a calculator that can solve word problems?
• Symbolab is the best calculator for solving a wide range of word problems, including age problems, distance problems, cost problems, investments problems, number problems, and percent problems.
• What is an age problem?
• An age problem is a type of word problem in math that involves calculating the age of one or more people at a specific point in time. These problems often use phrases such as 'x years ago,' 'in y years,' or 'y years later,' which indicate that the problem is related to time and age.

word-problems-calculator

• High School Math Solutions – Systems of Equations Calculator, Elimination A system of equations is a collection of two or more equations with the same set of variables. In this blog post,...

Please add a message.

Message received. Thanks for the feedback.

• Benefits to Participating Communities
• Participating School Districts
• Evaluations and Results
• Recognition Accorded
• National Advisory Committee
• Establishing New Institutes
• Topical Index of Curriculum Units
• View Topical Index of Curriculum Units
• Search Curricular Resources
• View Volumes of Curriculum Units from National Seminars
• Find Curriculum Units Written in Seminars Led by Yale Faculty
• Find Curriculum Units Written by Teachers in National Seminars
• Browse Curriculum Units Developed in Teachers Institutes
• On Common Ground
• Reports and Evaluations
• Articles and Essays
• Documentation
• Video Programs

Have a suggestion to improve this page?

To leave a general comment about our Web site, please click here

Share this page with your network.

Dr. Word Problem - Solving Word Problems with the Four Operations Using Singapore Bar Models

Introduction.

Dr. Word Problem and the Four Operations is a unit focused on the four operations of arithmetic. This unit explores the use of Singapore bar models as a tool for solving word problems. The unit teaches students how to represent word problems with Singapore bar models beginning with a basic addition fact. The lessons are scaffold to help the students to understand how to draw models to represent subtraction, multiplication, and division. The final phase addresses representing multi-step problems with bar models.

While the students at my school are high achievers in mathematics, word problems seem to be the weakest area of the math curriculum for them. My fourth graders typically have a difficult time deciding which operation a word problem requires. Often I find my students using some of the coping strategies and limited strategies that Sowder (1995) describes. One coping strategy that they use is adding the numbers that they find in the problem, regardless of what the word problem states. Another poor strategy choice is "Limited strategy 5, looking for isolated key words to tell which operation to use". This strategy is taught over and over at each grade level. Since each word problem is slightly different, children cannot figure out a strategy that automatically works for each one. Therefore, students try to develop coping strategies. Some teachers on the other hand are either teaching some of these limited strategies that are described in their teacher's manual, or they are teaching their students the way they were taught in school. This unit tries to look at word problems in a different way, so that students can develop a greater mathematical understanding that will enable them to be more successful problem solvers.

Through my work on this project, after analyzing word problems and looking for similarities and differences I have come to the following conclusions. When working with the simpler problems, students can learn and become more familiar with the basic structure of word problems. However, beyond the basic structure students will encounter differences within each problem. The subtle differences among very similar problems further complicate this subject matter. As students deal with multi-step problems there definitely is not a set approach to take. The students must truly understand what is going on mathematically in each situation.

So in this unit I have examined problems and grouped them into suites. The words and situations are different within the suites, but the basic structure is the same. By examining the different dimensions of the suites of problems, I have learned to approach problem solving in a clearer, more systematic way than I did in the past. This unit not only looks at the suites of problems, but also introduces the students to the Singapore bar models as a strategy for solving word problems.

The Singapore bar models provide the students with a visual component to help them understand what is going on mathematically in the problem. These models can be used to represent the four operations pictorially. The Singapore bar models are easy to draw and can be used to solve algebra problems without writing an equation. Hopefully, the combination of bar models and analyzing suites of problems will lead students and teachers to more successful problem solving and help to avoid some of the pitfalls caused by coping and limited strategies.

Attaching symbolic meaning to words is a difficult task for students at all levels. In fourth grade the students have just learned the four operations. This is really the first time that they have four operations to choose from when solving a problem. Their level of understanding the operations of multiplication and division, in particular, is in the beginning stages. So, word problems further complicate the issue because they have to figure out the words, the operation needed, and then they have to attach symbols to the words. This unit is intended to help students learn which mathematical operations to use when certain actions are presented in a problem. Upon completion of the unit I expect the students will have developed a greater understanding of the concepts of all four operations, and especially multiplication and division.

This unit is designed to be taught over a three-week period for approximately forty-five minutes per lesson. Beginning with addition, the students will experience suites of addition problems and the related subtraction problems. Next, the unit will move into multiplication and the related division problems. At the start, the suite of problems will involve one diagram focused around one scenario. These problems will have the same story line, but will be slightly different. The students will later face individual problems, not connected to their related counterparts, and the problems will involve one of the four operations. This is a more typical representation of the real world, where a word problem stands alone. After the students have learned the four operations and how to represent problems from all of the operations using a Singapore bar model, they will study two-step problems involving the four operations in any combination. The culminating activity will be for the students to put together a collection of word problems that include all four operations focused around a theme. My students will write their own word problems around one scenario of their choice, and also illustrate their scenarios with Singapore bar models.

A key feature of this unit is that it requires students to spend a significant portion of time working on word problems. Through this intensive exposure to word problems the students should become more comfortable with them and less afraid of them. Problem solving is a very important aspect of many of the high stakes tests that have become so important to school districts throughout the United States. On high stakes tests, the concepts are often embedded within a word problem. Some students automatically give up when they see the words. Others that know the concept have a difficult time understanding the task and are not able show what they know. By developing the confidence and the skills to tackle word problems, students will not only improve their math skills with the four basic operations, but also will hopefully transfer their knowledge to solving problems involving decimals, fractions, and all other areas of math.

The Singapore bar models were chosen for several reasons. First, Singapore leads the world in math achievement so the strategies that the Singapore approach teaches are proven to be successful. Secondly, the bar pictures are a visual representation of the words. They may help children translate from words to symbols, particularly if the words and language are difficult for them.

Another reason why this unit is appropriate and necessary for fourth graders is because the four operations are a major component of elementary math. Students need to understand the connectedness of the four operations and this unit ties the different operations together. According to Liping Ma, (Ma, 1999), there are two relationships that connect the four basic operations. These two relationships are the "derived operation" and the "inverse operation". An example of the "derived operation" is, "Multiplication is an operation derived from the operation of addition. It solves certain kinds of addition problems in an easier way" (Ma, 1999, p. 113). The inverse relationship is the other connection that Ma refers to in her explanation of the connections between the four operations. Subtraction is the inverse of addition because subtraction of a fixed number from a variable number is the inverse of addition of (the same) fixed number to a variable number. Multiplication and division are also inverse operations. Ma's (1999) analogy describes the importance of connecting the operations with this analogy:

"These two kinds of relationships tightly connect the four operations. Because all of the topics of elementary mathematics are related to the four operations, understanding of the relationships among the four operations, then, becomes a road system that connects all of elementary mathematics. With this road system, one can go anywhere in the domain" (Ma, 1999, p.113).

By developing a connected road system, I seek to help my students to develop a better understanding of arithmetic.

As students solve math and word problems, they need to be flexible and know different ways to approach the problems. While this unit is focused on one strategy, the Singapore bar models, the goal is to teach the students this technique so that they can increase their repertoire of strategies. Students will be required to use the bar models as I teach this unit. However, once this unit is completed, the students may or may not select the bar models as their approach to solving a given problem. As with any other strategy, some students will find that it works well for them and others may prefer a different method.

Why did I choose to use the Singapore bar models? Singapore students were first in the 1995, 1999 and 2003 Trends in International Mathematics and Science Study (TIMSS), which is designed to measure trends in students' mathematics and science achievement in four-year cycles. The TIMSS 2003 results were released on December 14, 2004. (New AIR Study Compares the Quality of U.S. Math Instruction with Singapore Recognized World Leader U.S. Trails, But Both Nations Could Learn from Each Other 2/7/05 http://www.air.org/news/ documents/Singapore.htm.)

Based on these findings I thought it would be interesting to expose my students and myself to Singapore models. Word problems seem to be particularly difficult for students. These models seem to simplify the problems and provide a visual representation to help the students to think about the problem. According to the Cognitively Guided Instruction model, "It is not children's manipulations of materials that is important; it is their understanding of the principles involved in the manipulations" (Carpenter, et al 1999, p. 68).

Based on a study conducted by the American Institute for Research (Leinwand et al., 2005) it is important to look to Singapore and to learn from how this country teaches math to its students. This report states:

"It is unreasonable to assume that Singaporean students have mathematical abilities inherently superior to those of U.S. students; rather, there must be something about the system that Singapore has developed to teach mathematics that is better than the system we use in the United States. That's why it's important to take a closer look, and see how the U.S can learn and how the U.S can improve."

Teacher Objectives

This unit has objectives for the teacher and for the students. First, I will discuss objectives for the teachers. In Ma's book (1999), the author compares math teachers in China and in the United States. There are striking differences in the knowledge base of the teachers. The Chinese teachers are more knowledgeable than American teachers in their own math skills and methods for solving equations. The book then analyzes reasons why. One main reason is summarized well with the analogy of the taxi driver. She compares the way the Chinese teachers understand math to a proficient taxi driver who knows all of the roads and many ways to get to the same place. Ma associates the American teachers to a newcomer in an area. A newcomer may know a few roads and one way to get to a location. Ma states, "A teacher's map of school mathematics must be more complicated and flexible" (Ma, 1999, p. 123).

A major objective of the seminar that led to the creation of this unit is for teachers to analyze and categorize word problems based on similarities and differences. It is this in depth analysis that really begins to help the teacher become a more proficient "taxi driver". At first glance, categorizing the word problems seems to be an easy task, but comparing similarities and differences goes beyond grouping the problems based on the operation involved. It looks more closely at the components and the structure of the problems the students are solving. There are several categories within each operation. Below the categories are defined and an example of each type of problem is given. These categories are from Children's Mathematics Cognitively Guided Instruction (Carpenter, et al., 1999). Additional insight has been added based on the article (Sowder, 1995).

Student Objectives

This unit clearly meets national and state standards since the four operations are such an integral part of elementary mathematics. In addition to simply learning the four operations, this unit looks at the relationships shared by the various operations. The inverse relationship of addition and subtraction, the inverse relationship of multiplication and division, and the relationship that addition and multiplication share are all explored through problem solving. By making connections between related word problems and examining different ways to solve the same word problems, students should develop a better understanding of the relationships between the four operations. As stated earlier, developing an understanding of the connectedness that the operations share is crucial to student learning.

Going beyond the basic calculations, students need to think critically and to develop problem-solving skills. This unit not only provides opportunities to develop these skills, but also teaches new strategies and approaches for dealing with word problems. The suites of problems should help make students aware of the connections that closely related problems share, and thus help them to develop a greater mathematical understanding. The expectation is that if students have a better understanding of the four operations they can use this knowledge to closely examine word problems and become better problem solvers.

As the strategies and activities of this unit unfold, the students will learn the Singapore bar model approach. This is just one strategy to help students solve word problems. It is visual and a more abstract "manipulative" to help students solve problems. This method will provide the students with one more approach or option when they face a word problem.

Addition and Subtraction Categories

Join problems involve the operation of addition. Within this category there are three different types. The most basic kind is when two quantities are joined and the result is unknown. Here is an example:

Daquan has 3 toy cars. His grandma buys him 2 more for his birthday. How many cars does he have now?

This problem would be solved with addition. Daquan starts with 3 toy cars and then 2 more toy cars that his grandma gives him are added to his collection. The answer is unknown, but by adding 3 and 2 the answer can be determined.

start unknown change

The answer is 5 toy cars.

This same scenario could be written slightly differently so that the change is unknown. In this situation,

Daquan has 3 toy cars. His grandma gives him some for his birthday. Now Daquan has 5 toy cars. How many toy cars did Daquan's grandma give him?

The representation of this problem is:

The [] represents 2 toy cars in this problem.

Even though this is an addition problem, the subtraction equation 5 - 3 = 2 is used to solve it. Larry Sowder (1995) refers to this type of problem as a missing addend problem. Missing addend problems are confusing for children because the real world operation is addition, as the equation shows. However, mathematically it is solved with subtraction.

The third type of join problem is when the start is unknown. This is another missing addend problem.

Daquan has some toy cars. His grandma gives him 2 more for his birthday. Now he has 5 toy cars. How many toy cars did Daquan have before his birthday?

The problem looks like this:

The answer is 3 toy cars. Once again subtraction 5 - 2 = 3 is used to solve for the unknown start.

This dimension is composed of the same three subcategories: result unknown, change unknown, and start unknown.

Sydney has 7 pieces of gum. She gives 2 pieces to her friends. How many pieces of gum does she have left?

This is a result unknown because you know how many Sydney started with and you know the change. The part that is unknown is the result. Subtraction is used to separate the 2 pieces of gum that Sydney gave away from the 7 pieces that she had in the beginning.

start result unknown change

The [] is 5 pieces of gum in this problem.

In the next problem, Sydney has an unknown change and once again subtraction is used to solve this type of problem.

Sydney had 7 pieces of gum. She gave some to her friends. Now she has 5 pieces left.

The number sentence below is used to solve for the answer, which is 2.

7 ñ [] = 5

She gave her friends 2 pieces of gum.

The last type in this suite of problems is when the start is unknown. This is another type of problem, missing start , that Sowder claims especially causes trouble for children. It seems to be a subtraction problem, but requires addition to solve it.

Sydney had some pieces of gum. She gave 2 pieces to her friends. She has 5 pieces left. How many pieces did she have before she gave some to her friends?

The number sentence for this problem can be written

The addition equation 2+ 5 = [] is used to solve this problem. She had 7 pieces of gum.

In the join and separate suites, three very similar problems can be created. Two of the problems deal with subtraction and one problem deals with addition. It is by revealing this kind of relationship between the problems and the operations that fosters a deeper understanding for the students.

Part-Part-Whole Problems

This dimension only has two different subcategories. As the name suggests either a part will be unknown or a whole will be unknown. There is no preferred order for a missing part. This is in contrast to the first two categories of problems, in which time provided a basis for ordering the numbers in the problem. An example of an unknown whole is:

There are 12 boys in the class and there are 10 girls in the class. How many students are in the class?

Addition is used to solve this problem. The major difference between the part-part-whole problems and the join problems is that there is no change over time: an action is not being performed and a quantity is not being changed or moved. The boy part and the girl part coexist and together equal the whole part. Students are not being added or removed.

The schema for this problem can be written

12 + 10 = []

The whole equals 22 students.

An example with an unknown part is:

There are 22 students in the class. There are 12 boys. How many are girls?

The problem is looking at the part of the class that is unknown. Despite the addition equation, subtraction is used to solve this one. A number sentence for this one could be

12 + [] = 22

The missing part is found by subtracting. The answer is 10 girls.

22 - 12 = []

Compare Problems

These problems are similar to the part-part-whole problems because there is no action involved. There is a set of objects or people and the problem is comparing the quantities. Carpenter (1999) classifies these problems based on three parts: the referent, the compared set, and the difference.

Mark has 6 baseball cards. Tom has 2. How many more baseball cards does Mark have?

referent compared difference unknown

In this problem, the difference is what is missing. Mark's baseball cards are the referent and Tom's cards are the compared set. These two variables are known. When difference is unknown subtraction is used to solve the problem.

Tom has 2 baseball cards and this is 4 less than Mark has, how many baseball cards does Mark have?

Here the unknown is the referent. The compared set and the difference are known. In order to solve this type of compare problem, addition would be needed. The appropriate number sentence is

4 + 2 = [].

A similar problem with an unknown compared set would look like this:

Mark has 6 baseball cards. Tom has less baseball cards than Mark. If the difference between their baseball card collections is 4, then how many baseball cards does Tom have?

In this problem subtraction is used.

6 - 4 = [].

Multiplication and Division Categories

Grouping and partitioning problems.

This category shares some similarities with the join category for addition and subtraction. Again, there can be three different unknowns depending how the problem is set up. When the compare category was analyzed three problems could be created. Two were subtraction and one was addition. Similarly with this category, three problems can be created, two for division and one for multiplication.

In interpreting these problems, it is important to take into account that there are two interpretations of division: measurement and partitive. Measurement division is when the number of the groups is unknown, and partitive is when the size of the group is unknown. It is important for students to learn that, although these problems may seem quite different, they are both solved by the same operation, division.

Let's say Zachary makes bags of lollipops for his friends. He puts 4 lollipops in each bag and makes 5 bags. How many lollipops did he use altogether?

This problem asks for the total, which indicates that it is a multiplication problem. So the equation would be:

In the next problem, the number of bags is unknown. This is measurement division.

Zachary has 20 lollipops. He makes some bags of lollipops for his friends. He puts four lollipops in each bag. How many bags can he make?

Equations representing this problem are

20 / 4 = [] or [] x 4 = 20.

As the second equation suggests, this problem could also be written as a missing factor problem using the multiplication.

A third type of problem calls for partitive division because the number in each group is unknown.

Zachary has 20 lollipops. He makes 5 bags. How many lollipops will he put in each bag?

20 / 5 = [] or [] x 5 = 20.

Rate Problems

There is also a group of problems known as rate problems. These are different from the previous group because they do not deal with objects that can be counted. They are a little more abstract, especially for children. The rate problems can also be divided into the same types: multiplication, measurement division, and partitive division.

A monkey eats 3 pounds of bananas a day. How many pounds of bananas will the monkey eat in 5 days?

This is a multiplication problem. We can represent it by

3 x 5 = [].

The next two problems will involve division.

A monkey eats 3 pounds of bananas a day. At this rate, how many days will 15 pounds of bananas feed the monkey?

This is a measurement division problem because the measurement or the number of days is missing. It can be schematized by the equations

15 / 3 = [] or 3x [] = 15.

A monkey eats 15 pounds of bananas in 5 days. If the monkey eats the same amount each day, how many pounds does the monkey eat in one day?

This problem is partitive division because the unknown is the amount or the part that the monkey eats in each day. The equation below will solve this problem.

15 /5 = [] or 5x [] = 15

Price Problems

These are similar to the previous group, but the rate involves a price. Oranges sell for 6 cents each. How much would 7 oranges cost? This is a multiplication problem and the equation to solve it is:

In the measurement division problem, the number of oranges is unknown.

Oranges sell for 6 cents each. How many can you buy for 42 cents?

Equations that capture this equations are

42/ 6 = [] or 6 x [] = 42.

The last one in this trio is the partitive division example.

You have 42 cents and you buy 7 oranges. How much does each orange cost?

Equations for this one are

42 /7 = [] or 7 x [] = 42

Multiplicative Comparisons

In these problems one quantity is described in relation to the other. For example:

The bowling ball weighs 5 times as much as the basketball. If the basketball weighs 4 pounds, how much does the bowling ball weigh?

Multiplication is used to solve this type of problem. It is represented by the equation

The equation looks like the other multiplication problems, but it is different from all of the other problems presented so far. The key concept is that one quantity is presented in relation to the other. One quantity is described in terms of how many times larger it is compared to the other.

This is by far the most daunting category. Based on the four operations, considering order, there are 16 possible types of problems. However, when all of the categories mentioned above are considered, there are a much greater number of combinations. My collection of multi-step problems, (see appendix) are by no means exhaustive of all of the different combinations. After collecting the problems, solving them, and classifying them, I soon realized the challenge of grouping them. I decided to group them based on the first step. Now I will discuss some of them from each category in more detail.

Here is problem number twenty-nine:

8 students sold 272 concert tickets at $3 each. Each student sold the same number of tickets. How much did each student collect?

The first step is to divide, since this is a grouping/partition problem. I must divide the number of tickets by the number of students. That means that I am doing partitive division. 272 divided by 8 equals 34. The next step is to multiply. This is a price problem with multiplication. 34 times $3 equals $102. Each student collected 102 dollars.

Interestingly enough, this problem could also be solved a different way. The number of tickets could have been multiplied by the ticket price first, to find the total amount of money collected. Then this would be a price problem with multiplication. Then the next step would be to divide the total amount of money, $816 by the 8 students. This would be a partitive division problem since I am dividing into a certain number of groups. The solution is $816 divided by 8 yields the same answer of $102. These alternative solution methods reflect the fact that multiplication is commutative (and that division by 8 is multiplication by 1/8).

Regardless of the order, the same operations and classification for those operations was used in the two methods of solving this problem. Certainly, my classifications of these multi-step problems are not the only way they can be grouped. However, it is a very reasonable first pass. Remember, Ma urged teachers to be more flexible. So, if a different way is chosen, then that is showing flexibility, which is a good thing. For the purpose of brevity, I am not going to continue to explore multiple ways of solving each problem as I go through the multi-step domain.

Here is problem 30:

Mr. Stone bought some books for $301. Each book cost $7. If his wife carried 18 books and he carried the rest. How many books did Mr. Stone carry?

This problem falls into the price category and uses measurement division in the first step because the total number of books has to be found. To do this, the total cost $301 is divided by the cost of one book. After performing this computation, we know that Mr. Stone had 43 books in total. The next step is to subtract, but since I am analyzing the problem, just knowing to subtract is not enough. I know that Mr. Stone and his wife are carrying them some place, presumably to the same place, so the books are not being separated. So, I considered this to be a part-part-whole problem involving a missing part. Now, I can complete the calculation by subtracting 18 from 43. The difference is 25, so Mr. Stone carried 25 books.

In number 33,

Rebecca bought four boxes of doughnuts. There were 6 doughnuts in each box. She divided the doughnuts equally among eight people. How many doughnuts did each person receive?

Based on my classification by first step, this is a grouping/partitioning problem involving multiplication. Six times four equals twenty-four. The next step is a grouping/partition problem that uses partitive division. 24 doughnuts divided by 8 people. So the answer is 3. Each person received 3 doughnuts. Interestingly all of the numbers in this problem are factors of 24.

Number 37 states:

A toy car costs $5. A toy airplane costs 4 times as much as the toy car. How much more does the toy airplane cost than the toy car?

The first step for me is multiplicative comparison. The cost of the car is multiplied by four since the airplane costs four times as much as the car. Therefore, $5 times 4 equals $20. The next step is to compare the prices using subtraction to find the unknown difference. The difference is $15 since $20 minus $5 equals $15.

In problem 40,

Rani had $47. After paying for 3kg of shrimp, she had $20 left. Find the cost of 1 kg of shrimp.

The first step is subtraction. It is a separate problem with an unknown change. Rani had $47. She spent some unknown amount and now is left with $20. After performing the calculation ($47-$20), the unknown change is determined to be $27. The next step is to divide $27 by 3. This is a price problem using partitive division. The cost of 1 kg would be $9.

A final problem from my collection of multi-step problems is from the part-part-whole domain. Number forty-three states:

A farmer had 2,000 chickens and ducks. After selling some of them, he had 650 chickens and 520 ducks left. How many chickens and ducks did he sell altogether?

First I chose to perform addition to find how many chickens and ducks a farmer has left after selling some of them. 650 chickens plus 520 ducks equals 1,170 chicken and ducks. Now I am left with a separate problem to find the unknown difference. I know that it is a separate problem because the chickens and ducks are being sold and separated from the group. 2,000 take away 1,170 equals 830 chicken and ducks that were sold.

Strategies for Teaching Students to Use the Singapore Bar Models

I plan to begin with basic addition facts that are less than 20. I will teach the students how to draw Singapore bar models to illustrate addition and subtraction facts. The problem will be presented in a very straightforward manner such as, the sum of 7 and 8, or the difference of 15 and 8. Once the students can create these, I plan to progress to two digit numbers followed by three digit numbers. This step will involve a slightly different model with the use of brackets to represent the number for which each part stands. The next step is for the students, when presented with a word problem, to make sense of the words and draw a bar model to represent it. From this point, I will show the students how one model can represent different problems within a given scenario. Typically, there are several scenarios for the same problem. All parts of the problem are represented in the diagram and different problems can be written based on the parts that are known and unknown. The problems that are generated include problems related by inverse relationships. This strategy strengthens the students' understanding of the relationships shared by the four operations. I plan to have the students draw one illustration for each scenario. As the students become proficient the task will change. I expect the students to move into writing several related word problems for a given model.

When the students have mastered addition and subtraction word problems by modeling with bar diagrams, I will teach them how to construct a similar model for multiplication and division. Initially I plan to provide a word problem that involves a basic fact such as 3 x7 and demonstrate and discuss the bar model representation. After sharing a few models, I will ask the students to construct the models given a basic fact. From this stage, I will teach the students how to name several problems with the same bar model. This process again involves the inverse relationships and the fact that multiplication is derived form addition. The final step is to have the students write several different scenarios for the same bar model involving multiplication and division.

The final stage is to tackle multi-step problems with a bar picture that combines different operations. These are significantly more complicated, but by this stage the students should be comfortable with the models. I will begin by guiding the students through models of each dimension. Then the students will work in groups to categorize problems based on the two operations needed to solve the problem. Next the students will solve the problems. I expect this stage will take more practice than the previous ones.

A teaching strategy that I will use for instruction and for having the children model their problems is found on the interactive Thinking Blocks website. Using this website as a teaching tool, I will initially show the students the video demonstrations through the computer onto the projection screen. After the class views the video, we will create our own problem. The students will participate in the process by manipulating the online models to create and solve the problem. Then the students could use this tool and work with partners to model and solve a problem given to them. This site can also be used for remediation, centers, for class work and homework, for enrichment, and as a teaching tool/resource.

The initial introduction to the Singapore bar models will involve simple straightforward addition and subtraction problems. An example of each kind follows and both of the problems come from the Primary Mathematics 3A Workbook (1992).

The sum of 9 and 7 is ____.

The difference between 9 and 7 is ____.

Using a basic fact less than 20 will introduce the students to how the models work. I will give the students several examples using basic facts less than 20 until they grasp the concept. Then I will introduce them to the slightly more abstract diagram where the units are no longer represented individually. For example:

Find the sum of 65 and 89.

The sum of 65 and 89 is ______.

This model above is representative of the way the models appear once the students have been introduced to them. The end-to-end model is preferable since it is linear and makes a connection to measurement. I will have the students solve several problems that are just numerical, using the end-to end model. Then the students will use construction paper cut-outs to create models on their paper. This will provide a hands-on way to create the models. Once the students have made some with pre-cut models, they will draw their own representations. In making all models, one requirement will be that the related equation should also be written.

Following the work with straightforward equations, the next step will be to move the students into using the models to represent word problems. Initially we will work with addition and subtraction. The same model can often represent several word problems related by the commutative property or the inverse relationship shared by addition and subtraction. Developing these concepts through problem solving will help to make these connections more meaningful for the students and will lead to greater motivation.

Ryan's sister is 15 years old. How old is Ryan if he is 4 years older than his sister?

The equation that goes with this problem is 15+4=19 years or 4+15=19 years. A few other problems that could be asked using this same model, but changing where the unknown is, are as follows:

Ryan is 19 years old. His sister is 15 years old. How many years older is Ryan?

The equation for this problem is: 15+4=19 years.

Ryan is 19 years old. He is 4 years older than his sister. How old is his sister?

The equation for this problem is: 19 - 4 = 15 years old

Once the students are solving word problems, they will be required to write the equation and also to label the answers. The focus will be on being able to solve the problem with a bar model while also showing a corresponding equation. The numbers within the word problem will get more challenging. Similar problems with three digit numbers would be the next step to take with the students. The students will work with the same model for several related scenarios where each time the unknown is different. These related problems will continue to show inverse relationships and the commutative property. Once the students are comfortable with the models and with writing related problems, I will be able to move quickly to more difficult numbers since addition and subtraction through the millions has already been covered.

In the next activity I will teach the students to use the bar models to show multiplication and division. I will once again begin on the computer with the projection screen. I will use the Thinking Blocks website. This time I will model a multiplication problem. The Singapore bar models would be cumbersome to draw with a large multiplier, as 7 x 36. These examples come from the Primary Mathematics Workbook 3A (1992).

Roy had 3 times as many comic books as Samy. If Samy had 7 comic books, how many comic books did Roy have?

Roy had ____ comic books? The equation for this problem is 7x3=21. Once again by changing the unknown a new problem is created.

In this next problem the inverse relationship of division is represented. Roy has 21 comic books. Roy has 3 times as many comic books as Samy has. How many comic books does Samy have?

Much as I did with addition and subtraction, I am pairing up the inverse operations of multiplication and division to help the students to build a critical piece of understanding. By developing this understanding of the relationship between multiplication and division, the students will not only learn their division facts more easily, but also become better problem solvers. Carpenter, Franke, & Levi (2003) assert, "understanding the relation between multiplication and division is critical for learning division number facts and for dealing flexibly with problem situations involving multiplication and division."

Again, once the students learn how to use the model to represent division, the difficulty can increase to the level of their division skills. More difficult division problems can be solved later in the year, once long division has been taught.

The multi-step domain will be considerably more complicated to teach. I am focusing only on two-step problems. Mathematically there are 16 different combinations of the four operations since the order must be taken into account. Initially, I will group these problems together based on their types, or their similarities and differences. I will share examples of problems with the corresponding bar model. After taking the students through examples of each dimension, I will have the students work in cooperative groups to read word problems, look at the bar model and identify the two operations. They will also have to figure out the proper order in which to complete the operations. Once the students are proficient with this skill, I will move them into actually solving these two-step problems. This will begin as group work then it will progress to partner work and independent work.

Meihua saved $24. She saved 3 times as much as Weilin.

Write the missing numbers in the boxes as you answer each question below.

(a) How much did Weilin save?

(b) How much did they save together?

I will provide the students with many opportunities to solve the multi-step problems since these vary so much. The students will participate in discussions about the problems because my students can learn so much from each other. While practicing these problems it would be fun to give each student a word problem written on an index card. We could go outside and the students could draw the Singapore bar models and solve the problem on the blacktop using sidewalk chalk. The key to conquering the multi-step problems will be keeping it fun. This will be more frustrating for some students, but by mixing up the activities and keeping it fun the students will be more motivated.

Appendix A: Collection of Problems

Addition and subtraction.

Starter Problems for Singapore Bar Models

1) The sum of 6 and 8 is _____.

2) The sum of 9 and 7 is _____.

3) Find the sum of 65 and 89.

4) The difference between 9 and 7 is _____.

5) Find the difference between 96 and 68.

6) Find the difference between 387 and 512.

7) When 376 is subtracted from a number the answer is 825. Find the number.

Join R - Result Unknown C - Change Unknown S - Start Unknown

8R) Nancy has 5 M&M's. Jermaine adds 3 M&M's to her pile. How many do they have altogether?

8C) Nancy has 5 M&M's. Jermaine adds his to her collection. Now there are 8 M & M's. How many did Jermaine have?

8S) Nancy has some M&M's. If Jermaine adds 3 to her collection they will have 8. How many does Nancy have at the start?

Separate R - Result Unknown C - Change Unknown S - Start Unknown

9C) Mrs. Rogers made 150 pineapple tarts for a party. After the party, there were 16 tarts left. How many tarts were eaten at the party?

9R) Mrs. Rogers made 150 pineapple tarts for a party. The guests ate 134 tarts. How many tarts were left?

9S) Mrs. Rogers made some tarts for the party. The guests ate 134 tarts. She had 16 left. How many tarts did Mrs. Rogers make?

Part Part Whole P- Part Unknown W - Whole Unknown

10P) Sharon is blowing up 52 balloons for a party. She has 24 left to blow up. How many has she already blown up?

10P) Sharon is blowing up 52 balloons for a party. She has blown up 28. How many balloons does she have left to blow up?

10W) Sharon is blowing up balloons for a party. She has blown up 28. She has 24 left to blow up. How many balloons will she have blown up when she is finished?

Compare D - Difference Unknown C - Compare Unknown R - Referent Unknown

11R) Bob's sister 23 years old. She is 9 years older than Bob. How old is Bob?

11C) Bob is 14 years old. His sister is 9 years older. How old is his sister?

11D) Bob is 14 years old. His sister is 23 years old. How many years is the age difference between them?

12C) Mr. Jones traveled 386 km on Monday. He traveled 147 km more on Sunday than Monday. How many kilometers did he travel on Sunday?

13D) Randy collected 124 matchboxes. Josiah collected 283 matchboxes. Who collected more matchboxes? How many more?

14R) There are 3,402 boys at the parade. There are 987 more boys than girls. How many girls are there? How many pupils are there altogether?

Multiplication and Division

15) Find the product of 41 x 5.

16) Find the product of 3 x 52.

Grouping/Partitioning Problems

M - Multiplication DM - Division-Measurement DP - Division-Partitive

17DP) Tim printed 900 pamphlets. He packed them equally into 8 boxes. How many pamphlets were there in each box? How many pamphlets were left over?

18M) Jessica put some cookies equally into 3 jars. If each jar had 24 cookies, how many cookies were there altogether?

19DM) Molly sold 70 flowers. They were in bunches of 5. How many bunches of flowers did she sell?

M - Multiplication D - Division-Measurement DP - Division-Partitive

20DP) Molly saved $32 in 4 weeks. She saved the same amount of money each week. How much did she save in a week?

21M) Kayla used 4 kg of flour to make cakes. She used 18 eggs for each kilogram of flour. How many eggs did she use?

22DM) A bucket holds 7 liters of water. A tank holds 637 liters of water. How many buckets of water can the tank hold?

Price M - Multiplication DM - Division-Measurement D - Division-Partitive

23DM) Mrs. Gray paid $98 for the basket of fish. 1kg sells for $7. How many kg did she buy?

24DP) A fruit seller sold 12 kg of grapes. He collected $96. How much does 1kg cost?

25M) Mr. Smith sells fruit for $8 per kg. He sells 37 kg in one day. How much money did his customers spend on fruit at his fruit stand?

Multiplicative Comparison

26) Roy has 3 times as many comic books as Samy. If Samy had 7 comic books, how many comic books did Roy have?

27) There are 20 monkeys in a zoo. There are 4 times as many monkeys as tigers. How many tigers are there?

28) A florist has 145 yellow roses. She has 8 times as many red roses as yellow roses. How many more red roses than yellow roses does she have?

Multistep (annotated to show components)

1st step -Partitive Division

29) 8 students sold 272 concert tickets at $3 each. Each student sold the same number of tickets. How much money did each student collect? (partitive division/ price -muliplication)

1st step - Measurement Division

30) Mr. Stone bought some books for $301. Each book cost $7. His wife helped him carry 18 books and he carried the rest. How many books did he carry? (price- measurement division/ part-part-whole subtraction missing part)

31) Mr. Lee sold 462 flowers in bunches of 6 each. Each bunch sold for $5. How much money did he receive? (grouping/partition-division-measurement/ price-multiplication)

32) Miss Brown baked 600 pineapple tarts for sale. She packed them into packets of 6. She sold 45 packets. How many packets were not sold? (grouping/partition -measurement division/ separate- result unknown)

1st step Multiplication (grouping partition)

33) Rebecca bought four boxes of doughnuts. There were 6 doughnuts in each box. She divided the doughnuts equally among eight people. How many doughnuts did each person receive? (grouping/partition-multiplication/grouping/partition partitive division)

34) Jordan had 9 bags of paper plates. There were 10 plates in each bag. 25 plates were used for a party. How many plates were left? (grouping/partition- multiplication/ separate- result unknown)

35) Steven bought 7 planks, each 3 m long. He bought another plank 2 m long. Find the total length of the 8 planks. (rate - multiplication/ join - addition - result unknown)

36) Marty reads 8 pages of a book a day. After reading the book for 4 days, she still has 5 pages to read. How many pages are in the book? (rate -multiplication/part-part-whole-addition)

1st step Multiplicative Comparison

37) A toy car costs $5. A toy airplane costs 4 times as much as the toy car. How much more does the toy airplane cost than the toy car? (multiplicative comparison/ compare-subtract- result unknown)

38) A pole is 3m long. A rope is 8 times as long as the pole. If the rope is divided into 4 pieces, what is the length of each piece of rope? (multiplicative comparison/ grouping/partition - partitive division)

39) There are 650 men and twice as many women working in a factory. How many workers are there in the factory? (multiplicative comparison/ part-part-whole - addition- missing whole)

1st step Subtract

40) Rani had $47. After paying for 3kg of shrimp, she had $20 left. Find the cost of 1kg of shrimp. (price- subtract -unknown change/ price - partitive division)

41) A computer cost $1,900. A television set was $650 cheaper than the computer. Mr. Ray bought both the computer and the television set. How much did he pay? (compare - subtract - difference unknown/ join- addition- result unknown)

42) Pablo earns $3,000 a month. He spends $2,200 a month and saves the rest. How much money can he save in 6 months? (price - separate -difference unknown/ price - multiplication)

1st step Part-Part-Whole

43) A farmer had 2,000 chickens and ducks. After selling some of them, he had 650 chickens and 520 ducks left. How many chickens and ducks did he sell altogether? (part-part-whole - addition - missing whole/ separate- difference unknown)

44) 4 men and 5 women shared the cost of a present equally. The cost of the present was $117. How much did each of them pay? (part-part-whole - addition - whole unknown/ price - partitive division)

Appendix B: Resources for Classroom Use

The following resources are used in these lessons: an internet connection, a computer, an LCD projector, a collection of word problems, construction paper, glue, sidewalk chalk, paper and pencils.

Appendix C: Annotated Bibliography

Carpenter, T., Fennema, E., Franke, M., Levi, L., & Empson, S. (1999). Children's

mathematics: Cognitively guided instruction. Portsmouth, NH: Heinemann. This was a great book that clearly identifies different types of word problems.

Carpenter, T., Franke, M., & Levi, L. (2003) Thinking mathematically: Integrating

arithmetic & algebra in elementary school. Portsmouth, NH: Heinemann. This was not very useful for this unit, but is important for developing algebra concepts early.

Leinwand, Steven, (2005). New AIR study compares the quality of U.S. math instruction

with Singapore recognized world leader U.S. trails, but both nations could learn from

each other. 2/7/05 http://www.air.org/news/documents/ Singapore.htm. This website shares the results of a study that ranks Singapore the world leader in math achievement.

Ma, Liping. (1999). Knowing and teaching elementary mathematics. Mahwah, NJ:

Lawrence Erlbaum Associates, Publishers. This book compares Chinese teachers to American teachers and reveals some striking differences.

Singapore primary math texts, U.S. Edition Curriculum Planning and Development Division, Ministry of Education, Singapore: Federal Publications. These textbooks and workbooks have a more simplistic appearance, but are more challenging than the corresponding U.S. grade level. These books show bar models and present place value in a slightly different way.

Sowder, Larry, (1995). Addressing the story-problem problem. In J. Sowder & B.

Schappelle (Eds.), Providing a foundation for teaching mathematics in the middle

grades (123-131). New York: State University of New York Press. An interesting article that looks at limited and desired strategies for problem solving.

Thinking Blocks, (2006) http://www.thinkingblocks.com. Date last accessed 7/3/07. This is a great website and offers many uses for teachers and students.

Appendix D: Implementing District Standards:

Virginia Standards of Learning Grade 4: The essential knowledge and skills for each of these objectives lists problem solving.

4.6 The student will add and subtract whole numbers written in vertical and horizontal form, choosing appropriately between paper and pencil methods and calculators.

4.7 The student will find the product of two whole numbers when one factor has two digits or fewer and the other factor has three digits or fewer.

4.8 The student will estimate and find the quotient of two whole numbers, given a one- digit divisor.

Comments (1)

Send us your comment

Solving Word Questions

With LOTS of examples!

In Algebra we often have word questions like:

Example: Sam and Alex play tennis.

On the weekend Sam played 4 more games than Alex did, and together they played 12 games.

How many games did Alex play?

How do we solve them?

The trick is to break the solution into two parts:

Turn the English into Algebra.

Then use Algebra to solve.

Turning English into Algebra

To turn the English into Algebra it helps to:

• Read the whole thing first
• Do a sketch if possible
• Assign letters for the values
• Find or work out formulas

You should also write down what is actually being asked for , so you know where you are going and when you have arrived!

Also look for key words:

Thinking Clearly

Some wording can be tricky, making it hard to think "the right way around", such as:

Example: Sam has 2 dollars less than Alex. How do we write this as an equation?

• Let S = dollars Sam has
• Let A = dollars Alex has

Now ... is that: S − 2 = A

or should it be: S = A − 2

or should it be: S = 2 − A

The correct answer is S = A − 2

( S − 2 = A is a common mistake, as the question is written "Sam ... 2 less ... Alex")

Example: on our street there are twice as many dogs as cats. How do we write this as an equation?

• Let D = number of dogs
• Let C = number of cats

Now ... is that: 2D = C

or should it be: D = 2C

Think carefully now!

The correct answer is D = 2C

( 2D = C is a common mistake, as the question is written "twice ... dogs ... cats")

Let's start with a really simple example so we see how it's done:

Example: A rectangular garden is 12m by 5m, what is its area ?

Turn the English into Algebra:

• Use w for width of rectangle: w = 12m
• Use h for height of rectangle: h = 5m

Formula for Area of a Rectangle : A = w × h

We are being asked for the Area.

A = w × h = 12 × 5 = 60 m 2

The area is 60 square meters .

Now let's try the example from the top of the page:

Example: Sam and Alex play Tennis. On the weekend Sam played 4 more games than Alex did, and together they played 12 games. How many games did Alex play?

• Use S for how many games Sam played
• Use A for how many games Alex played

We know that Sam played 4 more games than Alex, so: S = A + 4

And we know that together they played 12 games: S + A = 12

We are being asked for how many games Alex played: A

Which means that Alex played 4 games of tennis.

Check: Sam played 4 more games than Alex, so Sam played 8 games. Together they played 8 + 4 = 12 games. Yes!

A slightly harder example:

Example: Alex and Sam also build tables. Together they make 10 tables in 12 days. Alex working alone can make 10 in 30 days. How long would it take Sam working alone to make 10 tables?

• Use a for Alex's work rate
• Use s for Sam's work rate

12 days of Alex and Sam is 10 tables, so: 12a + 12s = 10

30 days of Alex alone is also 10 tables: 30a = 10

We are being asked how long it would take Sam to make 10 tables.

30a = 10 , so Alex's rate (tables per day) is: a = 10/30 = 1/3

Which means that Sam's rate is half a table a day (faster than Alex!)

So 10 tables would take Sam just 20 days.

Should Sam be paid more I wonder?

And another "substitution" example:

Example: Jenna is training hard to qualify for the National Games. She has a regular weekly routine, training for five hours a day on some days and 3 hours a day on the other days. She trains altogether 27 hours in a seven day week. On how many days does she train for five hours?

• The number of "5 hour" days: d
• The number of "3 hour" days: e

We know there are seven days in the week, so: d + e = 7

And she trains 27 hours in a week, with d 5 hour days and e 3 hour days: 5d + 3e = 27

We are being asked for how many days she trains for 5 hours: d

The number of "5 hour" days is 3

Check : She trains for 5 hours on 3 days a week, so she must train for 3 hours a day on the other 4 days of the week.

3 × 5 hours = 15 hours, plus 4 × 3 hours = 12 hours gives a total of 27 hours

Some examples from Geometry:

Example: A circle has an area of 12 mm 2 , what is its radius?

• Use A for Area: A = 12 mm 2
• Use r for radius

And the formula for Area is: A = π r 2

We are being asked for the radius.

We need to rearrange the formula to find the area

Example: A cube has a volume of 125 mm 3 , what is its surface area?

Make a quick sketch:

• Use V for Volume
• Use A for Area
• Use s for side length of cube
• Volume of a cube: V = s 3
• Surface area of a cube: A = 6s 2

We are being asked for the surface area.

First work out s using the volume formula:

Now we can calculate surface area:

An example about Money:

Example: Joel works at the local pizza parlor. When he works overtime he earns 1¼ times the normal rate. One week Joel worked for 40 hours at the normal rate of pay and also worked 12 hours overtime. If Joel earned $660 altogether in that week, what is his normal rate of pay?

• Joel's normal rate of pay: $N per hour
• Joel works for 40 hours at $N per hour = $40N
• When Joel does overtime he earns 1¼ times the normal rate = $1.25N per hour
• Joel works for 12 hours at $1.25N per hour = $(12 × 1¼N) = $15N
• And together he earned $660, so:

$40N + $(12 × 1¼N) = $660

We are being asked for Joel's normal rate of pay $N.

So Joel’s normal rate of pay is $12 per hour

Joel’s normal rate of pay is $12 per hour, so his overtime rate is 1¼ × $12 per hour = $15 per hour. So his normal pay of 40 × $12 = $480, plus his overtime pay of 12 × $15 = $180 gives us a total of $660

More about Money, with these two examples involving Compound Interest

Example: Alex puts $2000 in the bank at an annual compound interest of 11%. How much will it be worth in 3 years?

This is the compound interest formula:

So we will use these letters:

• Present Value PV = $2,000
• Interest Rate (as a decimal): r = 0.11
• Number of Periods: n = 3
• Future Value (the value we want): FV

We are being asked for the Future Value: FV

Example: Roger deposited $1,000 into a savings account. The money earned interest compounded annually at the same rate. After nine years Roger's deposit has grown to $1,551.33 What was the annual rate of interest for the savings account?

The compound interest formula:

• Present Value PV = $1,000
• Interest Rate (the value we want): r
• Number of Periods: n = 9
• Future Value: FV = $1,551.33

We are being asked for the Interest Rate: r

So the annual rate of interest is 5%

Check : $1,000 × (1.05) 9 = $1,000 × 1.55133 = $1,551.33

And an example of a Ratio question:

Example: At the start of the year the ratio of boys to girls in a class is 2 : 1 But now, half a year later, four boys have left the class and there are two new girls. The ratio of boys to girls is now 4 : 3 How many students are there altogether now?

• Number of boys now: b
• Number of girls now: g

The current ratio is 4 : 3

Which can be rearranged to 3b = 4g

At the start of the year there was (b + 4) boys and (g − 2) girls, and the ratio was 2 : 1

b + 4 g − 2 = 2 1

Which can be rearranged to b + 4 = 2(g − 2)

We are being asked for how many students there are altogether now: b + g

There are 12 girls !

And 3b = 4g , so b = 4g/3 = 4 × 12 / 3 = 16 , so there are 16 boys

So there are now 12 girls and 16 boys in the class, making 28 students altogether .

There are now 16 boys and 12 girls, so the ratio of boys to girls is 16 : 12 = 4 : 3 At the start of the year there were 20 boys and 10 girls, so the ratio was 20 : 10 = 2 : 1

And now for some Quadratic Equations :

Example: The product of two consecutive even integers is 168. What are the integers?

Consecutive means one after the other. And they are even , so they could be 2 and 4, or 4 and 6, etc.

We will call the smaller integer n , and so the larger integer must be n+2

And we are told the product (what we get after multiplying) is 168, so we know:

n(n + 2) = 168

We are being asked for the integers

That is a Quadratic Equation , and there are many ways to solve it. Using the Quadratic Equation Solver we get −14 and 12.

Check −14: −14(−14 + 2) = (−14)×(−12) = 168 YES

Check 12: 12(12 + 2) = 12×14 = 168 YES

So there are two solutions: −14 and −12 is one, 12 and 14 is the other.

Note: we could have also tried "guess and check":

• We could try, say, n=10: 10(12) = 120 NO (too small)
• Next we could try n=12: 12(14) = 168 YES

But unless we remember that multiplying two negatives make a positive we might overlook the other solution of (−14)×(−12).

Example: You are an Architect. Your client wants a room twice as long as it is wide. They also want a 3m wide veranda along the long side. Your client has 56 square meters of beautiful marble tiles to cover the whole area. What should the length of the room be?

Let's first make a sketch so we get things right!:

• the length of the room: L
• the width of the room: W
• the total Area including veranda: A
• the width of the room is half its length: W = ½L
• the total area is the (room width + 3) times the length: A = (W+3) × L = 56

We are being asked for the length of the room: L

This is a quadratic equation , there are many ways to solve it, this time let's use factoring :

And so L = 8 or −14

There are two solutions to the quadratic equation, but only one of them is possible since the length of the room cannot be negative!

So the length of the room is 8 m

L = 8, so W = ½L = 4

So the area of the rectangle = (W+3) × L = 7 × 8 = 56

There we are ...

... I hope these examples will help you get the idea of how to handle word questions. Now how about some practice?

• Math Article
• Word Problems On Integers

Integers: Word Problems On Integers

An arithmetic operation is an elementary branch of mathematics. Arithmetical operations include addition, subtraction, multiplication and division. Arithmetic operations are applicable to different types of numbers including integers.

Integers are a special group of numbers that do not have a fractional or a decimal part. It includes positive numbers, negative numbers and zero.  Arithmetic operations on integers are similar to that of whole numbers. Since integers can be positive or negative numbers i.e. as these numbers are preceded either by a positive (+) or a negative sign (-), it makes them a little confusing concept. Therefore, they are different from whole numbers . Let us now see how various arithmetical operations can be performed on integers with the help of a few word problems. Solve the following word problems using various rules of operations of integers.

Word problems on integers Examples:

Example 1: Shyak has overdrawn his checking account by Rs.38.  The bank debited him Rs.20 for an overdraft fee.  Later, he deposited Rs.150.  What is his current balance?

Solution:  Given,

Total amount deposited= Rs. 150

Amount overdrew by Shyak= Rs. 38

Amount charged by bank= Rs. 20

⇒ Debit amount= -20

Total amount debited = (-38) + (-20) = -58

Current balance= Total deposit +Total Debit

Hence, the current balance is Rs. 92.

Example 2: Anna is a microbiology student. She was doing research on optimum temperature for the survival of different strains of bacteria. Studies showed that bacteria X need optimum temperature of -31˚C while bacteria Y need optimum temperature of -56˚C. What is the temperature difference?

Solution: Given,

Optimum temperature for bacteria X = -31˚C

Optimum temperature for bacteria Y= -56˚C

Temperature difference= Optimum temperature for bacteria X – Optimum temperature for bacteria Y

⇒ (-31) – (-56)

Hence, temperature difference is 25˚C.

Example 3: A submarine submerges at the rate of 5 m/min. If it descends from 20 m above the sea level, how long will it take to reach 250 m below sea level?

Initial position = 20 m    (above sea level)

Final position = 250 m    (below sea level)

Total depth it submerged = (250+20) = 270 m

Thus, the submarine travelled 270 m below sea level.

Time taken to submerge 1 meter = 1/5 minutes

Time taken to submerge 270 m = 270 (1/5) = 54 min

Hence, the submarine will reach 250 m below sea level in 54 minutes.

To solve more problems on the topic, download BYJU’S – The Learning App and watch interactive videos. Also, take free tests to practice for exams.

Leave a Comment Cancel reply

Your Mobile number and Email id will not be published. Required fields are marked *

Request OTP on Voice Call

Post My Comment

Register with BYJU'S & Download Free PDFs

Register with byju's & watch live videos.

• + ACCUPLACER Mathematics
• + ACT Mathematics
• + AFOQT Mathematics
• + ALEKS Tests
• + ASVAB Mathematics
• + ATI TEAS Math Tests
• + Common Core Math
• + DAT Math Tests
• + FSA Tests
• + FTCE Math
• + GED Mathematics
• + Georgia Milestones Assessment
• + GRE Quantitative Reasoning
• + HiSET Math Exam
• + HSPT Math
• + ISEE Mathematics
• + PARCC Tests
• + Praxis Math
• + PSAT Math Tests
• + PSSA Tests
• + SAT Math Tests
• + SBAC Tests
• + SIFT Math
• + SSAT Math Tests
• + STAAR Tests
• + TABE Tests
• + TASC Math
• + TSI Mathematics
• + ACT Math Worksheets
• + Accuplacer Math Worksheets
• + AFOQT Math Worksheets
• + ALEKS Math Worksheets
• + ASVAB Math Worksheets
• + ATI TEAS 6 Math Worksheets
• + FTCE General Math Worksheets
• + GED Math Worksheets
• + 3rd Grade Mathematics Worksheets
• + 4th Grade Mathematics Worksheets
• + 5th Grade Mathematics Worksheets
• + 6th Grade Math Worksheets
• + 7th Grade Mathematics Worksheets
• + 8th Grade Mathematics Worksheets
• + 9th Grade Math Worksheets
• + HiSET Math Worksheets
• + HSPT Math Worksheets
• + ISEE Middle-Level Math Worksheets
• + PERT Math Worksheets
• + Praxis Math Worksheets
• + PSAT Math Worksheets
• + SAT Math Worksheets
• + SIFT Math Worksheets
• + SSAT Middle Level Math Worksheets
• + 7th Grade STAAR Math Worksheets
• + 8th Grade STAAR Math Worksheets
• + THEA Math Worksheets
• + TABE Math Worksheets
• + TASC Math Worksheets
• + TSI Math Worksheets
• + AFOQT Math Course
• + ALEKS Math Course
• + ASVAB Math Course
• + ATI TEAS 6 Math Course
• + CHSPE Math Course
• + FTCE General Knowledge Course
• + GED Math Course
• + HiSET Math Course
• + HSPT Math Course
• + ISEE Upper Level Math Course
• + SHSAT Math Course
• + SSAT Upper-Level Math Course
• + PERT Math Course
• + Praxis Core Math Course
• + SIFT Math Course
• + 8th Grade STAAR Math Course
• + TABE Math Course
• + TASC Math Course
• + TSI Math Course
• + Number Properties Puzzles
• + Algebra Puzzles
• + Geometry Puzzles
• + Intelligent Math Puzzles
• + Ratio, Proportion & Percentages Puzzles
• + Other Math Puzzles

How to Perform Operations of Decimals: Word Problems

Greetings, budding mathematicians! Today, we're going to jump into an adventure with decimals. Working with decimals is a fundamental math skill that you'll use in many aspects of life, from managing money to measuring distances.

Introduction to Decimal Operations

First things first, let’s talk about decimals. A decimal is a way of representing a number that’s less than one, or a number that’s a whole and a fraction together. When you work with decimals, the same rules apply as when you’re working with whole numbers, but the decimal point plays a crucial role.

There are four major operations that you can perform on decimals:

• Subtraction
• Multiplication

Now, let’s tackle some word problems involving decimal operations.

Step-By-Step Guide to Solving Word Problems with Decimal Operations

Step 1: understand the problem.

First and foremost, carefully read through the problem. Identify what you know and what you need to find out.

Step 2: Plan the Solution

Next, decide which operation (addition, subtraction, multiplication, or division) will help you solve the problem. This will depend on what the problem is asking.

Step 3: Carry Out the Operation

Perform the operation on the decimals. Remember to align the decimal points when you’re adding or subtracting, and apply the rules for multiplication and division of decimals correctly.

Step 4: Check the Answer

Finally, check if your answer makes sense in the context of the problem.

Consider this problem: Laura bought \(2.3\) kilograms of apples and \(1.5\) kilograms of grapes. How many kilograms of fruit did she buy in total?

Step 1: Understand that you know the weight of apples and grapes separately, and you need to find the total weight.

Step 2: Realize that to find the total, you need to add the weights together.

Step 3: Add the weights: \(2.3\ kg + 1.5\ kg = 3.8\ kg\).

Step 4: Check that the answer makes sense. Laura bought \(2.3\ kg\) of apples and \(1.5\ kg\) of grapes, so it makes sense that she bought \(3.8\ kg\) in total.

Keep practicing with different word problems, and you’ll get the hang of decimal operations in no time. Remember, the more you practice, the better you’ll get. Happy calculating!

by: Effortless Math Team about 11 months ago (category: Articles )

Effortless Math Team

Related to this article, more math articles.

• How to Use Grids to Multiply One-digit Numbers By Teen Numbers
• Graphing Histograms
• How to Master the Concept of Continuity over an Interval
• FREE 8th Grade Georgia Milestones Assessment System Math Practice Test
• How to Prepare for FTCE General Knowledge Math Test?
• How to Solve Trig Ratios of General Angles? (+FREE Worksheet!)
• Dollars and Sense: How to Tackle Money Word Problems with Confidence
• How to Multiply Matrix? (+FREE Worksheet!)
• Top 10 ATI TEAS 7 Math Practice Questions
• How Is the Accuplacer Test Scored?

What people say about "How to Perform Operations of Decimals: Word Problems - Effortless Math: We Help Students Learn to LOVE Mathematics"?

No one replied yet.

Leave a Reply Cancel reply

You must be logged in to post a comment.

Mastering Grade 6 Math Word Problems The Ultimate Guide to Tackling 6th Grade Math Word Problems

Mastering grade 5 math word problems the ultimate guide to tackling 5th grade math word problems, mastering grade 7 math word problems the ultimate guide to tackling 7th grade math word problems, mastering grade 2 math word problems the ultimate guide to tackling 2nd grade math word problems, mastering grade 8 math word problems the ultimate guide to tackling 8th grade math word problems, mastering grade 4 math word problems the ultimate guide to tackling 4th grade math word problems, mastering grade 3 math word problems the ultimate guide to tackling 3rd grade math word problems.

• ATI TEAS 6 Math
• ISEE Upper Level Math
• SSAT Upper-Level Math
• Praxis Core Math
• 8th Grade STAAR Math

Limited time only!

Save Over 45 %

It was $89.99 now it is $49.99

Login and use all of our services.

Effortless Math services are waiting for you. login faster!

Register Fast!

Password will be generated automatically and sent to your email.

After registration you can change your password if you want.

• Math Worksheets
• Math Courses
• Math Topics
• Math Puzzles
• Math eBooks
• GED Math Books
• HiSET Math Books
• ACT Math Books
• ISEE Math Books
• ACCUPLACER Books
• Premium Membership
• Youtube Videos

Effortless Math provides unofficial test prep products for a variety of tests and exams. All trademarks are property of their respective trademark owners.

• Bulk Orders
• Refund Policy

Rational Number Word Problems

These lessons help Grade 7 students learn how to solve real-world and mathematical problems involving the four operations with rational numbers.

Related Pages Common Core for Grade 7 Common Core for Mathematics More Lessons for Grade 7

Common Core: 7.NS.3

Suggested Learning Targets

I can solve mathematical and real-world problems involving four operations with rational numbers. (Tom had pieces of rope. Rope 1 was 5 ½ feet long. Rope 2 was 74 inches long. Rope 3 was 1 ½ yards long. What is the total length of rope?)

I can justify the steps taken to solve multi-step mathematical and real-world problems involving rational numbers.

Solve real-world problems involving the four operations with rational numbers (7.NS.3) In this lesson you will learn to use addition and subtraction to solve real-world problems involving decimals by analyzing the situation described in the problem.

Example 1: A recipe for trail mix calls for 3/4 cup dried fruits, 1/2 cup mixed nuts, and 1/3 cup granola. How many cups of trail mix does this recipe make?

Example 2: Anastasia was 19 1/4 inches at birth. At her 3 month checkup, she measures 23 1/2 inches. How much has she grown?

Example 3: The Darwin D. Martin house, built by Frank Lloyd Wright, has a rectangular stained glass window with a length of 41 1/2 feet and a width of 26 1/4 feet. What is the area of the window?

Example 4: You are an editor for your school yearbook. Each row of photos is 8 5/8 inches wide, including the margin. Each photo is 1 1/4 inches wide, the space between each photo is 1/8 inch, and each margin is 1/4 inch. How many photos can fit in one row?

Rational number word problem

Example: Most liquids when cooled will simply shrink. Water on the other hand, actually expands when it is frozen. Its volume will increase by about 9 percent, Suppose you have one third of a gallon of water that gets frozen. What is the volume of the ice?

Example: In the year 1944, computers weighed as much as 4,500 kilograms. A modern laptop weighs around 2.7 kilograms. What is the ratio of how much computers weighed in 1944 to how much a modern laptop weighs? (Express your answer as a ratio of two integers)

Example: Shares of stock represents how much of a company or person owns. Puff incorporated is owned by Peter, Paul and Mary. Peter owns 4,050 shares, Paul owns 2,510 shares and Mary owns 5600 shares. Suppose the company made a profit this year of $1,500,000. If each shareholder gets a proportion of the total profit that is equal to the proportion of the share they own. How much money does Mary receive? (Round to the nearest penny)

Rational Number Word Problems and PEMDAS

The science teacher is filling her new fish aquarium. The aquarium holds 40 gallons. If she fills the aquarium 4/5 of the way full, how many gallons will she need?

A baker needs to make 8 batches of cookies for a party. If each batch requires 2 3/4 cups of flour, how many cups will he need?

Mike is putting up a fence in the back yard. The fence comes in sections that are 4 2/3 feet long. If the yard is 34 feet long, how many sections will he need to buy?

Bella volunteers to make cookies for her math class. Each batch of cookies requires 1 2/3 cups of flour. If she has 12 cups of flour, how many batches of cookies can she make?

Trey is buying a computer on sale. The original price was $1,200. If the sale price is 5/6 of the original price, how much did Trey pay for the computer?

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.

High Impact Tutoring Built By Math Experts

Personalized standards-aligned one-on-one math tutoring for schools and districts

In order to access this I need to be confident with:

Fraction word prob.

Fraction word problems

Here you will learn about fraction word problems, including solving math word problems within a real-world context involving adding fractions, subtracting fractions, multiplying fractions, and dividing fractions.

Students will first learn about fraction word problems as part of number and operations—fractions in 4 th grade.

What are fraction word problems?

Fraction word problems are math word problems involving fractions that require students to use problem-solving skills within the context of a real-world situation.

To solve a fraction word problem, you must understand the context of the word problem, what the unknown information is, and what operation is needed to solve it. Fraction word problems may require addition, subtraction, multiplication, or division of fractions.

After determining what operation is needed to solve the problem, you can apply the rules of adding, subtracting, multiplying, or dividing fractions to find the solution.

For example,

Natalie is baking 2 different batches of cookies. One batch needs \cfrac{3}{4} cup of sugar and the other batch needs \cfrac{2}{4} cup of sugar. How much sugar is needed to bake both batches of cookies?

You can follow these steps to solve the problem:

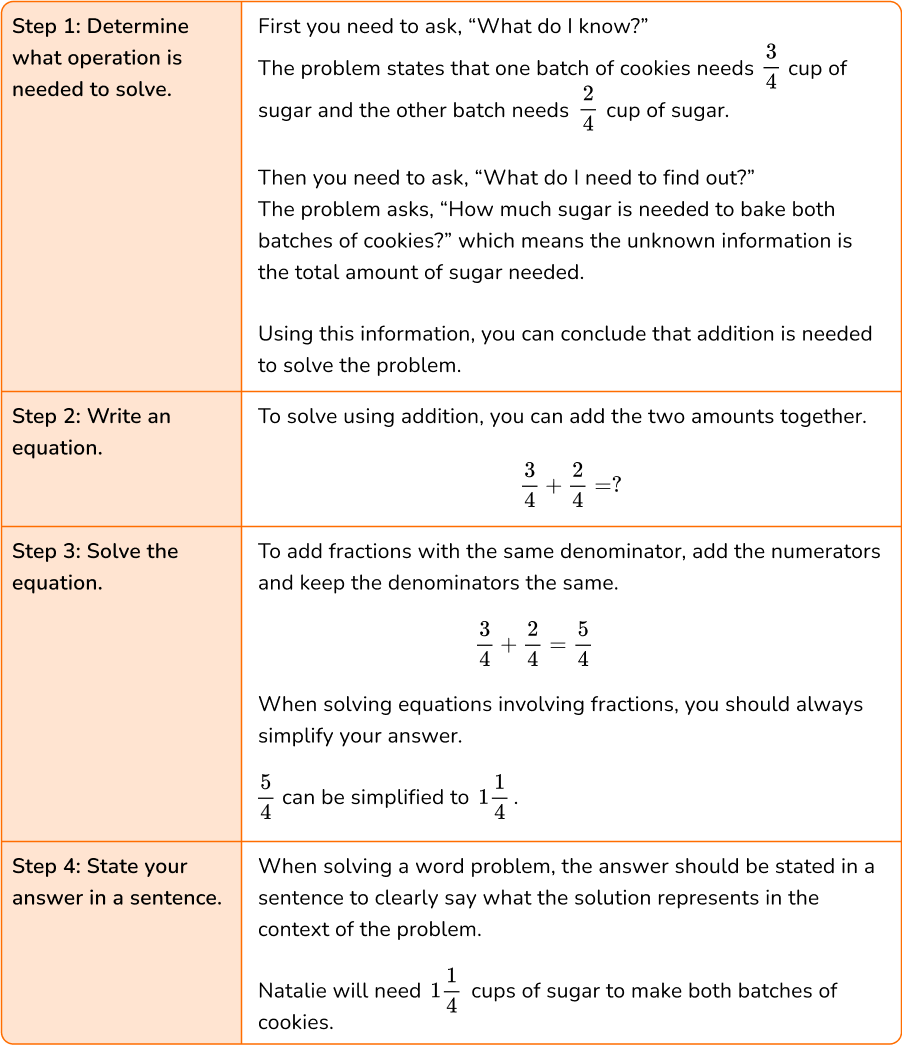
Step-by-step guide: Adding and subtracting fractions

Step-by-step guide: Adding fractions

Step-by-step guide: Subtracting fractions

Step-by-step guide: Multiplying and dividing fractions

Step-by-step guide: Multiplying fractions

Step-by-step guide: Dividing fractions

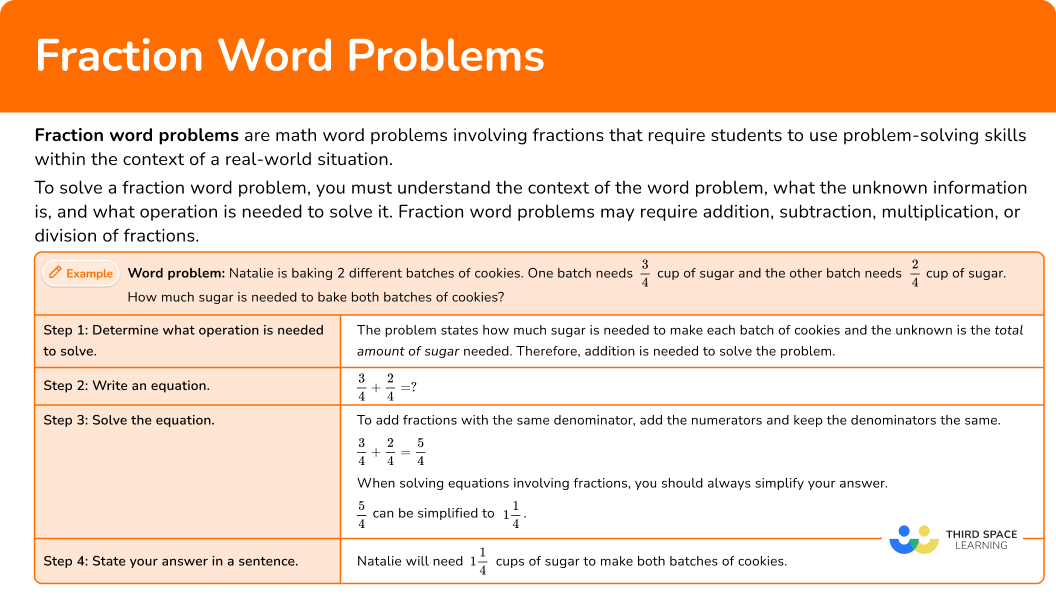
Common Core State Standards

How does this relate to 4 th grade math to 6 th grade math?

• Grade 4: Number and Operations—Fractions (4.NF.B.3d) Solve word problems involving addition and subtraction of fractions referring to the same whole and having like denominators, e.g., by using visual fraction models and equations to represent the problem.
• Grade 4: Number and Operations—Fractions (4.NF.B.4c) Solve word problems involving multiplication of a fraction by a whole number, e.g., by using visual fraction models and equations to represent the problem. For example, if each person at a party will eat \cfrac{3}{8} of a pound of roast beef, and there will be 5 people at the party, how many pounds of roast beef will be needed? Between what two whole numbers does your answer lie?
• Grade 5: Number and Operations—Fractions (5.NF.A.2) Solve word problems involving addition and subtraction of fractions referring to the same whole, including cases of unlike denominators, e.g., by using visual fraction models or equations to represent the problem. Use benchmark fractions and number sense of fractions to estimate mentally and assess the reasonableness of answers. For example, recognize an incorrect result \cfrac{2}{5}+\cfrac{1}{2}=\cfrac{3}{7} by observing that \cfrac{3}{7}<\cfrac{1}{2} .
• Grade 5: Number and Operations—Fractions (5.NF.B.6) Solve real world problems involving multiplication of fractions and mixed numbers, e.g., by using visual fraction models or equations to represent the problem.
• Grade 5: Number and Operations—Fractions (5.NF.B.7c) Solve real world problems involving division of unit fractions by non-zero whole numbers and division of whole numbers by unit fractions, e.g., by using visual fraction models and equations to represent the problem. For example, how much chocolate will each person get if 3 people share \cfrac{1}{2} \: lb of chocolate equally? How many \cfrac{1}{3} cup servings are in 2 cups of raisins?
• Grade 6: The Number System (6.NS.A.1) Interpret and compute quotients of fractions, and solve word problems involving division of fractions by fractions, e.g., by using visual fraction models and equations to represent the problem. For example, create a story context for \cfrac{2}{3} \div \cfrac{4}{5} and use a visual fraction model to show the quotient; use the relationship between multiplication and division to explain that \cfrac{2}{3} \div \cfrac{4}{5}=\cfrac{8}{9} because \cfrac{3}{4} of \cfrac{8}{9} is \cfrac{2}{3}. (In general, \cfrac{a}{b} \div \cfrac{c}{d}=\cfrac{a d}{b c} \, ) How much chocolate will each person get if 3 people share \cfrac{1}{2} \: lb of chocolate equally? How many \cfrac{3}{4} cup servings are in \cfrac{2}{3} of a cup of yogurt? How wide is a rectangular strip of land with length \cfrac{3}{4} \: m and area \cfrac{1}{2} \: m^2?

[FREE] Fraction Operations Worksheet (Grade 4 to 6)

Use this quiz to check your grade 4 to 6 students’ understanding of fraction operations. 10+ questions with answers covering a range of 4th to 6th grade fraction operations topics to identify areas of strength and support!

How to solve fraction word problems

In order to solve fraction word problems:

Determine what operation is needed to solve.

Write an equation.

Solve the equation.

State your answer in a sentence.

Fraction word problem examples

Example 1: adding fractions (like denominators).

Julia ate \cfrac{3}{8} of a pizza and her brother ate \cfrac{2}{8} of the same pizza. How much of the pizza did they eat altogether?

The problem states how much pizza Julia ate and how much her brother ate. You need to find how much pizza Julia and her brother ate altogether , which means you need to add.

2 Write an equation.

3 Solve the equation.

To add fractions with like denominators, add the numerators and keep the denominators the same.

4 State your answer in a sentence.

The last step is to go back to the word problem and write a sentence to clearly say what the solution represents in the context of the problem.

Julia and her brother ate \cfrac{5}{8} of the pizza altogether.

Example 2: adding fractions (unlike denominators)

Tim ran \cfrac{5}{6} of a mile in the morning and \cfrac{1}{3} of a mile in the afternoon. How far did Tim run in total?

The problem states how far Tim ran in the morning and how far he ran in the afternoon. You need to find how far Tim ran in total , which means you need to add.

To add fractions with unlike denominators, first find a common denominator and then change the fractions accordingly before adding.

\cfrac{5}{6}+\cfrac{1}{3}= \, ?

The least common multiple of 6 and 3 is 6, so 6 can be the common denominator.

That means \cfrac{1}{3} will need to be changed so that its denominator is 6. To do this, multiply the numerator and the denominator by 2.

\cfrac{1 \times 2}{3 \times 2}=\cfrac{2}{6}

Now you can add the fractions and simplify the answer.

\cfrac{5}{6}+\cfrac{2}{6}=\cfrac{7}{6}=1 \cfrac{1}{6}

Tim ran a total of 1 \cfrac{1}{6} miles.

Example 3: subtracting fractions (like denominators)

Pia walked \cfrac{4}{7} of a mile to the park and \cfrac{3}{7} of a mile back home. How much farther did she walk to the park than back home?

The problem states how far Pia walked to the park and how far she walked home. Since you need to find the difference ( how much farther ) between the two distances, you need to subtract.

To subtract fractions with like denominators, subtract the numerators and keep the denominators the same.

\cfrac{4}{7}-\cfrac{3}{7}=\cfrac{1}{7}

Pia walked \cfrac{1}{7} of a mile farther to the park than back home.

Example 4: subtracting fractions (unlike denominators)

Henry bought \cfrac{7}{8} pound of beef from the grocery store. He used \cfrac{1}{3} of a pound of beef to make a hamburger. How much of the beef does he have left?

The problem states how much beef Henry started with and how much he used. Since you need to find how much he has left , you need to subtract.

To subtract fractions with unlike denominators, first find a common denominator and then change the fractions accordingly before subtracting.

\cfrac{7}{8}-\cfrac{1}{3}= \, ?

The least common multiple of 8 and 3 is 24, so 24 can be the common denominator.

That means both fractions will need to be changed so that their denominator is 24.

To do this, multiply the numerator and the denominator of each fraction by the same number so that it results in a denominator of 24. This will give you an equivalent fraction for each fraction in the problem.

\begin{aligned}&\cfrac{7 \times 3}{8 \times 3}=\cfrac{21}{24} \\\\ &\cfrac{1 \times 8}{3 \times 8}=\cfrac{8}{24} \end{aligned}

Now you can subtract the fractions.

\cfrac{21}{24}-\cfrac{8}{24}=\cfrac{13}{24}

Henry has \cfrac{13}{24} of a pound of beef left.

Example 5: multiplying fractions

Andre has \cfrac{3}{4} of a candy bar left. He gives \cfrac{1}{2} of the remaining bit of the candy bar to his sister. What fraction of the whole candy bar does Andre have left now?

It could be challenging to determine the operation needed for this problem; many students may automatically assume it is subtraction since you need to find how much of the candy bar is left.

However, since you know Andre started with a fraction of the candy bar and you need to find a fraction OF a fraction, you need to multiply.

The difference here is that Andre did NOT give his sister \cfrac{1}{2} of the candy bar, but he gave her \cfrac{1}{2} of \cfrac{3}{4} of a candy bar.

To solve the word problem, you can ask, “What is \cfrac{1}{2} of \cfrac{3}{4}? ” and set up the equation accordingly. Think of the multiplication sign as meaning “of.”

\cfrac{1}{2} \times \cfrac{3}{4}= \, ?

To multiply fractions, multiply the numerators and multiply the denominators.

\cfrac{1}{2} \times \cfrac{3}{4}=\cfrac{3}{8}

Andre gave \cfrac{1}{2} of \cfrac{3}{4} of a candy bar to his sister, which means he has \cfrac{1}{2} of \cfrac{3}{4} left. Therefore, Andre has \cfrac{3}{8} of the whole candy bar left.

Example 6: dividing fractions

Nia has \cfrac{7}{8} cup of trail mix. How many \cfrac{1}{4} cup servings can she make?

The problem states the total amount of trail mix Nia has and asks how many servings can be made from it.

To solve, you need to divide the total amount of trail mix (which is \cfrac{7}{8} cup) by the amount in each serving ( \cfrac{1}{4} cup) to find out how many servings she can make.

To divide fractions, multiply the dividend by the reciprocal of the divisor.

\begin{aligned}& \cfrac{7}{8} \div \cfrac{1}{4}= \, ? \\\\ & \downarrow \downarrow \downarrow \\\\ &\cfrac{7}{8} \times \cfrac{4}{1}=\cfrac{28}{8} \end{aligned}

You can simplify \cfrac{28}{8} to \cfrac{7}{2} and then 3 \cfrac{1}{2}.

Nia can make 3 \cfrac{1}{2} cup servings.

Teaching tips for fraction word problems

• Encourage students to look for key words to help determine the operation needed to solve the problem. For example, subtracting fractions word problems might ask students to find “how much is left” or “how much more” one fraction is than another.
• Provide students with an answer key to word problem worksheets to allow them to obtain immediate feedback on their solutions. Encourage students to attempt the problems independently first, then check their answers against the key to identify any mistakes and learn from them. This helps reinforce problem-solving skills and confidence.
• Be sure to incorporate real-world situations into your math lessons. Doing so allows students to better understand the relevance of fractions in everyday life.
• As students progress and build a strong foundational understanding of one-step fraction word problems, provide them with multi-step word problems that involve more than one operation to solve.
• Take note that students will not divide a fraction by a fraction as shown above until 6 th grade (middle school), but they will divide a unit fraction by a whole number and a whole number by a fraction in 5 th grade (elementary school), where the same mathematical rules apply to solving.
• There are many alternatives you can use in place of printable math worksheets to make practicing fraction word problems more engaging. Some examples are online math games and digital workbooks.

Easy mistakes to make

• Misinterpreting the problem Misreading or misunderstanding the word problem can lead to solving for the wrong quantity or using the wrong operation.
• Not finding common denominators When adding or subtracting fractions with unlike denominators, students may forget to find a common denominator, leading to an incorrect answer.
• Forgetting to simplify Unless a problem specifically says not to simplify, fractional answers should always be written in simplest form.

Related fractions operations lessons

• Fractions operations
• Multiplicative inverse
• Reciprocal math
• Fractions as divisions

Practice fraction word problem questions

1. Malia spent \cfrac{5}{6} of an hour studying for a math test. Then she spent \cfrac{1}{3} of an hour reading. How much longer did she spend studying for her math test than reading?

Malia spent \cfrac{1}{2} of an hour longer studying for her math test than reading.

Malia spent \cfrac{5}{18} of an hour longer studying for her math test than reading.

Malia spent \cfrac{1}{2} of an hour longer reading than studying for her math test.

Malia spent 1 \cfrac{1}{6} of an hour longer studying for her math test than reading.

To find the difference between the amount of time Malia spent studying for her math test than reading, you need to subtract. Since the fractions have unlike denominators, you need to find a common denominator first.

You can use 6 as the common denominator, so \cfrac{1}{3} becomes \cfrac{3}{6}. Then you can subtract.

\cfrac{3}{6} can then be simplified to \cfrac{1}{2}.

Finally, you need to choose the answer that correctly answers the question within the context of the situation. Therefore, the correct answer is “Malia spent \cfrac{1}{2} of an hour longer studying for her math test than reading.”

2. A square garden is \cfrac{3}{4} of a meter wide and \cfrac{8}{9} of a meter long. What is its area?

The area of the garden is 1\cfrac{23}{36} square meters.

The area of the garden is \cfrac{27}{32} square meters.

The area of the garden is \cfrac{2}{3} square meters.

The perimeter of the garden is \cfrac{2}{3} meters.

To find the area of a square, you multiply the length and width. So to solve, you multiply the fractional lengths by mulitplying the numerators and multiplying the denominators.

\cfrac{24}{36} can be simplified to \cfrac{2}{3}. 

Therefore, the correct answer is “The area of the garden is \cfrac{2}{3} square meters.”

3. Zoe ate \cfrac{3}{8} of a small cake. Liam ate \cfrac{1}{8} of the same cake. How much more of the cake did Zoe eat than Liam?

Zoe ate \cfrac{3}{64} more of the cake than Liam.

Zoe ate \cfrac{1}{4} more of the cake than Liam.

Zoe ate \cfrac{1}{8} more of the cake than Liam.

Liam ate \cfrac{1}{4} more of the cake than Zoe.

To find how much more cake Zoe ate than Liam, you subtract. Since the fractions have the same denominator, you subtract the numerators and keep the denominator the same.

\cfrac{2}{8} can be simplified to \cfrac{1}{4}. 

Therefore, the correct answer is “Zoe ate \cfrac{1}{4} more of the cake than Liam.”

4. Lila poured \cfrac{11}{12} cup of pineapple and \cfrac{2}{3} cup of mango juice in a bottle. How many cups of juice did she pour into the bottle altogether?

Lila poured 1 \cfrac{7}{12} cups of juice in the bottle altogether.

Lila poured \cfrac{1}{4} cups of juice in the bottle altogether.

Lila poured \cfrac{11}{18} cups of juice in the bottle altogether.

Lila poured 1 \cfrac{3}{8} cups of juice in the bottle altogether.

To find the total amount of juice that Lila poured into the bottle, you need to add. Since the fractions have unlike denominators, you need to find a common denominator first.

You can use 12 as the common denominator, so \cfrac{2}{3} becomes \cfrac{8}{12}.  Then you can add.

\cfrac{19}{12} can be simplified to 1 \cfrac{7}{12}. 

Therefore, the correct answer is “Lila poured 1 \cfrac{7}{12} cups of juice in the bottle altogether.”

5. Killian used \cfrac{9}{10} of a gallon of paint to paint his living room and \cfrac{7}{10} of a gallon to paint his bedroom. How much paint did Killian use in all?

Killian used \cfrac{2}{10} gallons of paint in all.

Killian used \cfrac{1}{5} gallons of paint in all.

Killian used \cfrac{63}{100} gallons of paint in all.

Killian used 1 \cfrac{3}{5} gallons of paint in all.

To find the total amount of paint Killian used, you add the amount he used for the living room and the amount he used for the kitchen. Since the fractions have the same denominator, you add the numerators and keep the denominators the same.

\cfrac{16}{10} can be simplified to 1 \cfrac{6}{10} and then further simplified to 1 \cfrac{3}{5}.

Therefore, the correct answer is “Killian used 1 \cfrac{3}{5} gallons of paint in all.”

6. Evan pours \cfrac{4}{5} of a liter of orange juice evenly among some cups.

He put \cfrac{1}{10} of a liter into each cup. How many cups did Evan fill?

Evan filled \cfrac{2}{25} cups.

Evan filled 8 cups.

Evan filled \cfrac{9}{10} cups.

Evan filled 7 cups.

To find the number of cups Evan filled, you need to divide the total amount of orange juice by the amount being poured into each cup. To divide fractions, you mulitply the first fraction (the dividend) by the reciprocal of the second fraction (the divisor).

\cfrac{40}{5} can be simplifed to 8.

Therefore, the correct answer is “Evan filled 8 cups.”

Fraction word problems FAQs

Fraction word problems are math word problems involving fractions that require students to use problem-solving skills within the context of a real-world situation. Fraction word problems may involve addition, subtraction, multiplication, or division of fractions.

To solve fraction word problems, first you need to determine the operation. Then you can write an equation and solve the equation based on the arithmetic rules for that operation.

Fraction word problems and decimal word problems are similar because they both involve solving math problems within real-world contexts. Both types of problems require understanding the problem, determining the operation needed to solve it (addition, subtraction, multiplication, division), and solving it based on the arithmetic rules for that operation.

The next lessons are

Still stuck.

At Third Space Learning, we specialize in helping teachers and school leaders to provide personalized math support for more of their students through high-quality, online one-on-one math tutoring delivered by subject experts.

Each week, our tutors support thousands of students who are at risk of not meeting their grade-level expectations, and help accelerate their progress and boost their confidence.

Find out how we can help your students achieve success with our math tutoring programs .

[FREE] Common Core Practice Tests (Grades 3 to 6)

Prepare for math tests in your state with these Grade 3 to Grade 6 practice assessments for Common Core and state equivalents.

40 multiple choice questions and detailed answers to support test prep, created by US math experts covering a range of topics!

Privacy Overview