how to solve linear equations with fractional coefficients

• school Campus Bookshelves
• menu_book Bookshelves
• perm_media Learning Objects
• login Login
• how_to_reg Request Instructor Account
• hub Instructor Commons
• Download Page (PDF)
• Download Full Book (PDF)
• Periodic Table
• Physics Constants
• Scientific Calculator
• Reference & Cite
• Tools expand_more
• Readability

selected template will load here

This action is not available.

8.6: Solve Equations with Fraction or Decimal Coefficients

• Last updated
• Save as PDF
• Page ID 5022

\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)

\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)

\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)

( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)

\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)

\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)

\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)

\( \newcommand{\Span}{\mathrm{span}}\)

\( \newcommand{\id}{\mathrm{id}}\)

\( \newcommand{\kernel}{\mathrm{null}\,}\)

\( \newcommand{\range}{\mathrm{range}\,}\)

\( \newcommand{\RealPart}{\mathrm{Re}}\)

\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)

\( \newcommand{\Argument}{\mathrm{Arg}}\)

\( \newcommand{\norm}[1]{\| #1 \|}\)

\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)

\( \newcommand{\vectorA}[1]{\vec{#1}}      % arrow\)

\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}}      % arrow\)

\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)

\( \newcommand{\vectorC}[1]{\textbf{#1}} \)

\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)

\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)

\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)

Learning Objectives

Solve equations with fraction coefficients.

• Solve equations with decimal coefficients

be prepared!

Before you get started, take this readiness quiz.

• Multiply: 8 • \(\dfrac{3}{8}\). If you missed this problem, review Example 4.3.10 .
• Find the LCD of \(\dfrac{5}{6}\) and \(\dfrac{1}{4}\). If you missed this problem, review Example 4.8.1 .
• Multiply: 4.78 by 100. If you missed this problem, review Example 5.3.8 .

Solve Equations with Fraction Coefficients

Let’s use the General Strategy for Solving Linear Equations introduced earlier to solve the equation \(\dfrac{1}{8}x + \dfrac{1}{2} = \dfrac{1}{4}\).

This method worked fine, but many students don’t feel very confident when they see all those fractions. So we are going to show an alternate method to solve equations with fractions. This alternate method eliminates the fractions.

We will apply the Multiplication Property of Equality and multiply both sides of an equation by the least common denominator of all the fractions in the equation. The result of this operation will be a new equation, equivalent to the first, but with no fractions. This process is called clearing the equation of fractions . Let’s solve the same equation again, but this time use the method that clears the fractions.

Example \(\PageIndex{1}\):

Solve: \(\dfrac{1}{8} x + \dfrac{1}{2} = \dfrac{1}{4}\).

Exercise \(\PageIndex{1}\):

Solve: \(\dfrac{1}{4} x + \dfrac{1}{2} = \dfrac{5}{8}\).

\(x = \frac{1}{2}\)

Exercise \(\PageIndex{2}\):

Solve: \(\dfrac{1}{6} y - \dfrac{1}{3} = \dfrac{1}{6}\).

Notice in Example 8.37 that once we cleared the equation of fractions, the equation was like those we solved earlier in this chapter. We changed the problem to one we already knew how to solve! We then used the General Strategy for Solving Linear Equations.

HOW TO: SOLVE EQUATIONS WITH FRACTION COEFFICIENTS BY CLEARING THE FRACTIONS

Step 1. Find the least common denominator of all the fractions in the equation.

Step 2. Multiply both sides of the equation by that LCD. This clears the fractions.

Step 3. Solve using the General Strategy for Solving Linear Equations.

Example \(\PageIndex{2}\):

Solve: 7 = \(\dfrac{1}{2} x + \dfrac{3}{4} x − \dfrac{2}{3} x\).

We want to clear the fractions by multiplying both sides of the equation by the LCD of all the fractions in the equation.

Exercise \(\PageIndex{3}\):

Solve: 6 = \(\dfrac{1}{2} v + \dfrac{2}{5} v − \dfrac{3}{4} v\).

Exercise \(\PageIndex{4}\):

Solve: -1 = \(\dfrac{1}{2} u + \dfrac{1}{4} u − \dfrac{2}{3} u\).

In the next example, we’ll have variables and fractions on both sides of the equation.

Example \(\PageIndex{3}\):

Solve: \(x + \dfrac{1}{3} = \dfrac{1}{6} x − \dfrac{1}{2}\).

Exercise \(\PageIndex{5}\):

Solve: \(a + \dfrac{3}{4} = \dfrac{3}{8} a − \dfrac{1}{2}\).

Exercise \(\PageIndex{6}\):

Solve: \(c + \dfrac{3}{4} = \dfrac{1}{2} c − \dfrac{1}{4}\).

In Example 8.40, we’ll start by using the Distributive Property. This step will clear the fractions right away!

Example \(\PageIndex{4}\):

Solve: 1 = \(\dfrac{1}{2}\)(4x + 2).

Exercise \(\PageIndex{7}\):

Solve: −11 = \(\dfrac{1}{2}\)(6p + 2).

Exercise \(\PageIndex{8}\):

Solve: 8 = \(\dfrac{1}{3}\)(9q + 6).

Many times, there will still be fractions, even after distributing.

Example \(\PageIndex{5}\):

Solve: \(\dfrac{1}{2}\)(y − 5) = \(\dfrac{1}{4}\)(y − 1).

Exercise \(\PageIndex{9}\):

Solve: \(\dfrac{1}{5}\)(n + 3) = \(\dfrac{1}{4}\)(n + 2).

Exercise \(\PageIndex{10}\):

Solve: \(\dfrac{1}{2}\)(m − 3) = \(\dfrac{1}{4}\)(m − 7).

Solve Equations with Decimal Coefficients

Some equations have decimals in them. This kind of equation will occur when we solve problems dealing with money and percent. But decimals are really another way to represent fractions. For example, 0.3 = \(\dfrac{3}{10}\) and 0.17 = \(\dfrac{17}{100}\). So, when we have an equation with decimals, we can use the same process we used to clear fractions—multiply both sides of the equation by the least common denominator.

Example \(\PageIndex{6}\):

Solve: 0.8x − 5 = 7.

The only decimal in the equation is 0.8. Since 0.8 = \(\dfrac{8}{10}\), the LCD is 10. We can multiply both sides by 10 to clear the decimal.

Exercise \(\PageIndex{11}\):

Solve: 0.6x − 1 = 11.

Exercise \(\PageIndex{12}\):

Solve: 1.2x − 3 = 9.

Example \(\PageIndex{7}\):

Solve: 0.06x + 0.02 = 0.25x − 1.5.

Look at the decimals and think of the equivalent fractions.

\[0.06 = \dfrac{6}{100}, \qquad 0.02 = \dfrac{2}{100}, \qquad 0.25 = \dfrac{25}{100}, \qquad 1.5 = 1 \dfrac{5}{10}\]

Notice, the LCD is 100. By multiplying by the LCD we will clear the decimals.

Exercise \(\PageIndex{13}\):

Solve: 0.14h + 0.12 = 0.35h − 2.4.

Exercise \(\PageIndex{14}\):

Solve: 0.65k − 0.1 = 0.4k − 0.35.

The next example uses an equation that is typical of the ones we will see in the money applications in the next chapter. Notice that we will distribute the decimal first before we clear all decimals in the equation.

Example \(\PageIndex{8}\):

Solve: 0.25x + 0.05(x + 3) = 2.85.

Exercise \(\PageIndex{15}\):

Solve: 0.25n + 0.05(n + 5) = 2.95.

Exercise \(\PageIndex{16}\):

Solve: 0.10d + 0.05(d − 5) = 2.15.

ACCESS ADDITIONAL ONLINE RESOURCES

Solve an Equation with Fractions with Variable Terms on Both Sides

Ex 1: Solve an Equation with Fractions with Variable Terms on Both Sides

Ex 2: Solve an Equation with Fractions with Variable Terms on Both Sides

Solving Multiple Step Equations Involving Decimals

Ex: Solve a Linear Equation With Decimals and Variables on Both Sides

Ex: Solve an Equation with Decimals and Parentheses

Practice Makes Perfect

In the following exercises, solve the equation by clearing the fractions.

• \(\dfrac{1}{4} x − \dfrac{1}{2} = − \dfrac{3}{4}\)
• \(\dfrac{3}{4} x − \dfrac{1}{2} = \dfrac{1}{4}\)
• \(\dfrac{5}{6} y − \dfrac{2}{3} = − \dfrac{3}{2}\)
• \(\dfrac{5}{6} y − \dfrac{1}{3} = − \dfrac{7}{6}\)
• \(\dfrac{1}{2} a + \dfrac{3}{8} = \dfrac{3}{4}\)
• \(\dfrac{5}{8} b + \dfrac{1}{2} = − \dfrac{3}{4}\)
• 2 = \(\dfrac{1}{3} x − \dfrac{1}{2} x + \dfrac{2}{3} x\)
• 2 = \(\dfrac{3}{5} x − \dfrac{1}{3} x + \dfrac{2}{5} x\)
• \(\dfrac{1}{4} m − \dfrac{4}{5} m + \dfrac{1}{2} m\) = −1
• \(\dfrac{5}{6} n − \dfrac{1}{4} n − \dfrac{1}{2} n\) = −2
• \(x + \dfrac{1}{2} = \dfrac{2}{3} x − \dfrac{1}{2}\)
• \(x + \dfrac{3}{4} = \dfrac{1}{2} x − \dfrac{5}{4}\)
• \(\dfrac{1}{3} w + \dfrac{5}{4} = w − \dfrac{1}{4}\)
• \(\dfrac{3}{2} z + \dfrac{1}{3} = z − \dfrac{2}{3}\)
• \(\dfrac{1}{2} x − \dfrac{1}{4} = \dfrac{1}{12} x + \dfrac{1}{6}\)
• \(\dfrac{1}{2} a − \dfrac{1}{4} = \dfrac{1}{6} a + \dfrac{1}{12}\)
• \(\dfrac{1}{3} b + \dfrac{1}{5} = \dfrac{2}{5} b − \dfrac{3}{5}\)
• \(\dfrac{1}{3} x + \dfrac{2}{5} = \dfrac{1}{5} x − \dfrac{2}{5}\)
• 1 = \(\dfrac{1}{6}\)(12x − 6)
• 1 = \(\dfrac{1}{5}\)(15x − 10)
• \(\dfrac{1}{4}\)(p − 7) = \(\dfrac{1}{3}\)(p + 5)
• \(\dfrac{1}{5}\)(q + 3) = \(\dfrac{1}{2}\)(q − 3)
• \(\dfrac{1}{2}\)(x + 4) = \(\dfrac{3}{4}\)
• \(\dfrac{1}{3}\)(x + 5) = \(\dfrac{5}{6}\)

In the following exercises, solve the equation by clearing the decimals.

• 0.6y + 3 = 9
• 0.4y − 4 = 2
• 3.6j − 2 = 5.2
• 2.1k + 3 = 7.2
• 0.4x + 0.6 = 0.5x − 1.2
• 0.7x + 0.4 = 0.6x + 2.4
• 0.23x + 1.47 = 0.37x − 1.05
• 0.48x + 1.56 = 0.58x − 0.64
• 0.9x − 1.25 = 0.75x + 1.75
• 1.2x − 0.91 = 0.8x + 2.29
• 0.05n + 0.10(n + 8) = 2.15
• 0.05n + 0.10(n + 7) = 3.55
• 0.10d + 0.25(d + 5) = 4.05
• 0.10d + 0.25(d + 7) = 5.25
• 0.05(q − 5) + 0.25q = 3.05
• 0.05(q − 8) + 0.25q = 4.10

Everyday Math

• Coins Taylor has $2.00 in dimes and pennies. The number of pennies is 2 more than the number of dimes. Solve the equation 0.10d + 0.01(d + 2) = 2 for d, the number of dimes.
• Stamps Travis bought $9.45 worth of 49-cent stamps and 21-cent stamps. The number of 21-cent stamps was 5 less than the number of 49-cent stamps. Solve the equation 0.49s + 0.21(s − 5) = 9.45 for s, to find the number of 49-cent stamps Travis bought.

Writing Exercises

• Explain how to find the least common denominator of \(\dfrac{3}{8}, \dfrac{1}{6}\), and \(\dfrac{2}{3}\).
• If an equation has several fractions, how does multiplying both sides by the LCD make it easier to solve?
• If an equation has fractions only on one side, why do you have to multiply both sides of the equation by the LCD?
• In the equation 0.35x + 2.1 = 3.85, what is the LCD? How do you know?

(a) After completing the exercises, use this checklist to evaluate your mastery of the objectives of this section.

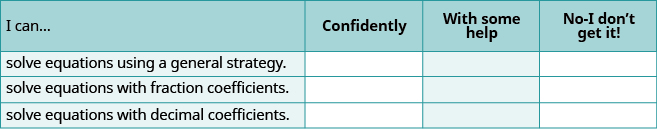
(b) Overall, after looking at the checklist, do you think you are well-prepared for the next Chapter? Why or why not?

Contributors and Attributions

Lynn Marecek (Santa Ana College) and MaryAnne Anthony-Smith (Formerly of Santa Ana College). This content is licensed under Creative Commons Attribution License v4.0 "Download for free at http://cnx.org/contents/[email protected] ."

8.4 Solve Equations with Fraction or Decimal Coefficients

Learning objectives.

By the end of this section, you will be able to:

Solve equations with fraction coefficients

• Solve equations with decimal coefficients

Be Prepared 8.10

Before you get started, take this readiness quiz.

Multiply: 8 · 3 8 . 8 · 3 8 . If you missed this problem, review Example 4.28

Be Prepared 8.11

Find the LCD of 5 6 and 1 4 . 5 6 and 1 4 . If you missed this problem, review Example 4.63

Be Prepared 8.12

Multiply: 4.78 4.78 by 100 . 100 . If you missed this problem, review Example 5.18

Solve Equations with Fraction Coefficients

Let’s use the General Strategy for Solving Linear Equations introduced earlier to solve the equation 1 8 x + 1 2 = 1 4 . 1 8 x + 1 2 = 1 4 .

This method worked fine, but many students don’t feel very confident when they see all those fractions. So we are going to show an alternate method to solve equations with fractions. This alternate method eliminates the fractions.

We will apply the Multiplication Property of Equality and multiply both sides of an equation by the least common denominator of all the fractions in the equation. The result of this operation will be a new equation, equivalent to the first, but with no fractions. This process is called clearing the equation of fractions . Let’s solve the same equation again, but this time use the method that clears the fractions.

Example 8.37

Solve: 1 8 x + 1 2 = 1 4 . 1 8 x + 1 2 = 1 4 .

Try It 8.73

Solve: 1 4 x + 1 2 = 5 8 . 1 4 x + 1 2 = 5 8 .

Try It 8.74

Solve: 1 6 y − 1 3 = 1 6 . 1 6 y − 1 3 = 1 6 .

Notice in Example 8.37 that once we cleared the equation of fractions, the equation was like those we solved earlier in this chapter. We changed the problem to one we already knew how to solve! We then used the General Strategy for Solving Linear Equations.

Solve equations with fraction coefficients by clearing the fractions.

• Step 1. Find the least common denominator of all the fractions in the equation.
• Step 2. Multiply both sides of the equation by that LCD. This clears the fractions.
• Step 3. Solve using the General Strategy for Solving Linear Equations.

Example 8.38

Solve: 7 = 1 2 x + 3 4 x − 2 3 x . 7 = 1 2 x + 3 4 x − 2 3 x .

We want to clear the fractions by multiplying both sides of the equation by the LCD of all the fractions in the equation.

Try It 8.75

Solve: 6 = 1 2 v + 2 5 v − 3 4 v . 6 = 1 2 v + 2 5 v − 3 4 v .

Try It 8.76

Solve: −1 = 1 2 u + 1 4 u − 2 3 u . −1 = 1 2 u + 1 4 u − 2 3 u .

In the next example, we’ll have variables and fractions on both sides of the equation.

Example 8.39

Solve: x + 1 3 = 1 6 x − 1 2 . x + 1 3 = 1 6 x − 1 2 .

Try It 8.77

Solve: a + 3 4 = 3 8 a − 1 2 . a + 3 4 = 3 8 a − 1 2 .

Try It 8.78

Solve: c + 3 4 = 1 2 c − 1 4 . c + 3 4 = 1 2 c − 1 4 .

In Example 8.40 , we’ll start by using the Distributive Property. This step will clear the fractions right away!

Example 8.40

Solve: 1 = 1 2 ( 4 x + 2 ) . 1 = 1 2 ( 4 x + 2 ) .

Try It 8.79

Solve: −11 = 1 2 ( 6 p + 2 ) . −11 = 1 2 ( 6 p + 2 ) .

Try It 8.80

Solve: 8 = 1 3 ( 9 q + 6 ) . 8 = 1 3 ( 9 q + 6 ) .

Many times, there will still be fractions, even after distributing.

Example 8.41

Solve: 1 2 ( y − 5 ) = 1 4 ( y − 1 ) . 1 2 ( y − 5 ) = 1 4 ( y − 1 ) .

Try It 8.81

Solve: 1 5 ( n + 3 ) = 1 4 ( n + 2 ) . 1 5 ( n + 3 ) = 1 4 ( n + 2 ) .

Try It 8.82

Solve: 1 2 ( m − 3 ) = 1 4 ( m − 7 ) . 1 2 ( m − 3 ) = 1 4 ( m − 7 ) .

Solve Equations with Decimal Coefficients

Some equations have decimals in them. This kind of equation will occur when we solve problems dealing with money and percent. But decimals are really another way to represent fractions. For example, 0.3 = 3 10 0.3 = 3 10 and 0.17 = 17 100 . 0.17 = 17 100 . So, when we have an equation with decimals, we can use the same process we used to clear fractions—multiply both sides of the equation by the least common denominator .

Example 8.42

Solve: 0.8 x − 5 = 7 . 0.8 x − 5 = 7 .

The only decimal in the equation is 0.8 . 0.8 . Since 0.8 = 8 10 , 0.8 = 8 10 , the LCD is 10 . 10 . We can multiply both sides by 10 10 to clear the decimal.

Try It 8.83

Solve: 0.6 x − 1 = 11 . 0.6 x − 1 = 11 .

Try It 8.84

Solve: 1.2 x − 3 = 9 . 1.2 x − 3 = 9 .

Example 8.43

Solve: 0.06 x + 0.02 = 0.25 x − 1.5 . 0.06 x + 0.02 = 0.25 x − 1.5 .

Look at the decimals and think of the equivalent fractions.

0.06 = 6 100 , 0.02 = 2 100 , 0.25 = 25 100 , 1.5 = 1 5 10 0.06 = 6 100 , 0.02 = 2 100 , 0.25 = 25 100 , 1.5 = 1 5 10

Notice, the LCD is 100 . 100 .

By multiplying by the LCD we will clear the decimals.

Try It 8.85

Solve: 0.14 h + 0.12 = 0.35 h − 2.4 . 0.14 h + 0.12 = 0.35 h − 2.4 .

Try It 8.86

Solve: 0.65 k − 0.1 = 0.4 k − 0.35 . 0.65 k − 0.1 = 0.4 k − 0.35 .

The next example uses an equation that is typical of the ones we will see in the money applications in the next chapter. Notice that we will distribute the decimal first before we clear all decimals in the equation.

Example 8.44

Solve: 0.25 x + 0.05 ( x + 3 ) = 2.85 . 0.25 x + 0.05 ( x + 3 ) = 2.85 .

Try It 8.87

Solve: 0.25 n + 0.05 ( n + 5 ) = 2.95 . 0.25 n + 0.05 ( n + 5 ) = 2.95 .

Try It 8.88

Solve: 0.10 d + 0.05 ( d − 5 ) = 2.15 . 0.10 d + 0.05 ( d − 5 ) = 2.15 .

ACCESS ADDITIONAL ONLINE RESOURCES

• Solve an Equation with Fractions with Variable Terms on Both Sides
• Ex 1: Solve an Equation with Fractions with Variable Terms on Both Sides
• Ex 2: Solve an Equation with Fractions with Variable Terms on Both Sides
• Solving Multiple Step Equations Involving Decimals
• Ex: Solve a Linear Equation With Decimals and Variables on Both Sides
• Ex: Solve an Equation with Decimals and Parentheses

Section 8.4 Exercises

Practice makes perfect.

In the following exercises, solve the equation by clearing the fractions.

1 4 x − 1 2 = − 3 4 1 4 x − 1 2 = − 3 4

3 4 x − 1 2 = 1 4 3 4 x − 1 2 = 1 4

5 6 y − 2 3 = − 3 2 5 6 y − 2 3 = − 3 2

5 6 y − 1 3 = − 7 6 5 6 y − 1 3 = − 7 6

1 2 a + 3 8 = 3 4 1 2 a + 3 8 = 3 4

5 8 b + 1 2 = − 3 4 5 8 b + 1 2 = − 3 4

2 = 1 3 x − 1 2 x + 2 3 x 2 = 1 3 x − 1 2 x + 2 3 x

2 = 3 5 x − 1 3 x + 2 5 x 2 = 3 5 x − 1 3 x + 2 5 x

1 4 m − 4 5 m + 1 2 m = −1 1 4 m − 4 5 m + 1 2 m = −1

5 6 n − 1 4 n − 1 2 n = −2 5 6 n − 1 4 n − 1 2 n = −2

x + 1 2 = 2 3 x − 1 2 x + 1 2 = 2 3 x − 1 2

x + 3 4 = 1 2 x − 5 4 x + 3 4 = 1 2 x − 5 4

1 3 w + 5 4 = w − 1 4 1 3 w + 5 4 = w − 1 4

3 2 z + 1 3 = z − 2 3 3 2 z + 1 3 = z − 2 3

1 2 x − 1 4 = 1 12 x + 1 6 1 2 x − 1 4 = 1 12 x + 1 6

1 2 a − 1 4 = 1 6 a + 1 12 1 2 a − 1 4 = 1 6 a + 1 12

1 3 b + 1 5 = 2 5 b − 3 5 1 3 b + 1 5 = 2 5 b − 3 5

1 3 x + 2 5 = 1 5 x − 2 5 1 3 x + 2 5 = 1 5 x − 2 5

1 = 1 6 ( 12 x − 6 ) 1 = 1 6 ( 12 x − 6 )

1 = 1 5 ( 15 x − 10 ) 1 = 1 5 ( 15 x − 10 )

1 4 ( p − 7 ) = 1 3 ( p + 5 ) 1 4 ( p − 7 ) = 1 3 ( p + 5 )

1 5 ( q + 3 ) = 1 2 ( q − 3 ) 1 5 ( q + 3 ) = 1 2 ( q − 3 )

1 2 ( x + 4 ) = 3 4 1 2 ( x + 4 ) = 3 4

1 3 ( x + 5 ) = 5 6 1 3 ( x + 5 ) = 5 6

In the following exercises, solve the equation by clearing the decimals.

0.6 y + 3 = 9 0.6 y + 3 = 9

0.4 y − 4 = 2 0.4 y − 4 = 2

3.6 j − 2 = 5.2 3.6 j − 2 = 5.2

2.1 k + 3 = 7.2 2.1 k + 3 = 7.2

0.4 x + 0.6 = 0.5 x − 1.2 0.4 x + 0.6 = 0.5 x − 1.2

0.7 x + 0.4 = 0.6 x + 2.4 0.7 x + 0.4 = 0.6 x + 2.4

0.23 x + 1.47 = 0.37 x − 1.05 0.23 x + 1.47 = 0.37 x − 1.05

0.48 x + 1.56 = 0.58 x − 0.64 0.48 x + 1.56 = 0.58 x − 0.64

0.9 x − 1.25 = 0.75 x + 1.75 0.9 x − 1.25 = 0.75 x + 1.75

1.2 x − 0.91 = 0.8 x + 2.29 1.2 x − 0.91 = 0.8 x + 2.29

0.05 n + 0.10 ( n + 8 ) = 2.15 0.05 n + 0.10 ( n + 8 ) = 2.15

0.05 n + 0.10 ( n + 7 ) = 3.55 0.05 n + 0.10 ( n + 7 ) = 3.55

0.10 d + 0.25 ( d + 5 ) = 4.05 0.10 d + 0.25 ( d + 5 ) = 4.05

0.10 d + 0.25 ( d + 7 ) = 5.25 0.10 d + 0.25 ( d + 7 ) = 5.25

0.05 ( q − 5 ) + 0.25 q = 3.05 0.05 ( q − 5 ) + 0.25 q = 3.05

0.05 ( q − 8 ) + 0.25 q = 4.10 0.05 ( q − 8 ) + 0.25 q = 4.10

Everyday Math

Coins Taylor has $2.00 $2.00 in dimes and pennies. The number of pennies is 2 2 more than the number of dimes. Solve the equation 0.10 d + 0.01 ( d + 2 ) = 2 0.10 d + 0.01 ( d + 2 ) = 2 for d , d , the number of dimes.

Stamps Travis bought $9.45 $9.45 worth of 49-cent 49-cent stamps and 21-cent 21-cent stamps. The number of 21-cent 21-cent stamps was 5 5 less than the number of 49-cent 49-cent stamps. Solve the equation 0.49 s + 0.21 ( s − 5 ) = 9.45 0.49 s + 0.21 ( s − 5 ) = 9.45 for s , s , to find the number of 49-cent 49-cent stamps Travis bought.

Writing Exercises

Explain how to find the least common denominator of 3 8 , 1 6 , and 2 3 . 3 8 , 1 6 , and 2 3 .

If an equation has several fractions, how does multiplying both sides by the LCD make it easier to solve?

If an equation has fractions only on one side, why do you have to multiply both sides of the equation by the LCD?

In the equation 0.35 x + 2.1 = 3.85 , 0.35 x + 2.1 = 3.85 , what is the LCD? How do you know?

ⓐ After completing the exercises, use this checklist to evaluate your mastery of the objectives of this section.

ⓑ Overall, after looking at the checklist, do you think you are well-prepared for the next Chapter? Why or why not?

As an Amazon Associate we earn from qualifying purchases.

This book may not be used in the training of large language models or otherwise be ingested into large language models or generative AI offerings without OpenStax's permission.

Want to cite, share, or modify this book? This book uses the Creative Commons Attribution License and you must attribute OpenStax.

Access for free at https://openstax.org/books/prealgebra-2e/pages/1-introduction

• Authors: Lynn Marecek, MaryAnne Anthony-Smith, Andrea Honeycutt Mathis
• Publisher/website: OpenStax
• Book title: Prealgebra 2e
• Publication date: Mar 11, 2020
• Location: Houston, Texas
• Book URL: https://openstax.org/books/prealgebra-2e/pages/1-introduction
• Section URL: https://openstax.org/books/prealgebra-2e/pages/8-4-solve-equations-with-fraction-or-decimal-coefficients

© Jan 23, 2024 OpenStax. Textbook content produced by OpenStax is licensed under a Creative Commons Attribution License . The OpenStax name, OpenStax logo, OpenStax book covers, OpenStax CNX name, and OpenStax CNX logo are not subject to the Creative Commons license and may not be reproduced without the prior and express written consent of Rice University.

Want to create or adapt books like this? Learn more about how Pressbooks supports open publishing practices.

Chapter 2: Linear Equations

2.4 Fractional Linear Equations

When working with fractions built into linear equations, it is often easiest to remove the fraction in the very first step. This generally means finding the LCD of the fraction and then multiplying every term in the entire equation by the LCD.

Example 2.4.1

Solve for [latex]x[/latex] in the equation [latex]\dfrac{3}{4}x - \dfrac{7}{2} = \dfrac{5}{6}.[/latex]

For this equation, the LCD is 12, so every term in this equation will be multiplied by 12.

[latex]\dfrac{3}{4}x(12) - \dfrac{7}{2}(12) = \dfrac{5}{6}(12)[/latex]

Cancelling out the denominator yields:

[latex]3x(3) - 7(6) = 5(2)[/latex]

Multiplying results in:

[latex]\begin{array}{rrrrr} 9x&-&42&=&10 \\ &+&42&&+42 \\ \hline &&\dfrac{9x}{9}&=&\dfrac{52}{9} \\ \\ &&x&=&\dfrac{52}{9} \end{array}[/latex]

Example 2.4.2

For questions 1 to 18, solve each linear equation.

• [latex]\dfrac{3}{5}\left(1 + p\right) = \dfrac{21}{20}[/latex]
• [latex]-\dfrac{1}{2} = \dfrac{3k}{2} + \dfrac{3}{2}[/latex]
• [latex]0 = -\dfrac{5}{4}\left(x-\dfrac{6}{5}\right)[/latex]
• [latex]\dfrac{3}{2}n - 8 = -\dfrac{29}{12}[/latex]
• [latex]\dfrac{3}{4} - \dfrac{5}{4}m = \dfrac{108}{24}[/latex]
• [latex]\dfrac{11}{4} + \dfrac{3}{4}r = \dfrac{160}{32}[/latex]
• [latex]2b + \dfrac{9}{5} = -\dfrac{11}{5}[/latex]
• [latex]\dfrac{3}{2} - \dfrac{7}{4}v = -\dfrac{9}{8}[/latex]
• [latex]\dfrac{3}{2}\left(\dfrac{7}{3}n+1\right) = \dfrac{3}{2}[/latex]
• [latex]\dfrac{41}{9} = \dfrac{5}{2}\left(x+\dfrac{2}{3}\right) - \dfrac{1}{3}x[/latex]
• [latex]-a - \dfrac{5}{4}\left(-\dfrac{8}{3}a+ 1\right) = -\dfrac{19}{4}[/latex]
• [latex]\dfrac{1}{3}\left(-\dfrac{7}{4}k + 1\right) - \dfrac{10}{3}k = -\dfrac{13}{8}[/latex]
• [latex]\dfrac{55}{6} = -\dfrac{5}{2}\left(\dfrac{3}{2}p-\dfrac{5}{3}\right)[/latex]
• [latex]-\dfrac{1}{2}\left(\dfrac{2}{3}x-\dfrac{3}{4}\right)-\dfrac{7}{2}x=-\dfrac{83}{24}[/latex]
• [latex]-\dfrac{5}{8}=\dfrac{5}{4}\left(r-\dfrac{3}{2}\right)[/latex]
• [latex]\dfrac{1}{12}=\dfrac{4}{3}x+\dfrac{5}{3}\left(x-\dfrac{7}{4}\right)[/latex]
• [latex]-\dfrac{11}{3}+\dfrac{3}{2}b=\dfrac{5}{2}\left(b-\dfrac{5}{3}\right)[/latex]
• [latex]\dfrac{7}{6}-\dfrac{4}{3}n=-\dfrac{3}{2}n+2\left(n+\dfrac{3}{2}\right)[/latex]

Answer Key 2.4

Intermediate Algebra Copyright © 2020 by Terrance Berg is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License , except where otherwise noted.

Share This Book

Want to create or adapt books like this? Learn more about how Pressbooks supports open publishing practices.

3.4 Solve Equations with Fraction or Decimal Coefficients

Learning Objectives

By the end of this section, you will be able to:

Solve equations with fraction coefficients

• Solve equations with decimal coefficients

Solve Equations with Fraction Coefficients

Given equation.

Simplify the left side.

Change the constants to equivalent fractions with the LCD.

This method worked fine, but many students don’t feel very confident when they see all those fractions. So we are going to show an alternate method to solve equations with fractions. This alternate method eliminates the fractions.

We will apply the Multiplication Property of Equality and multiply both sides of an equation by the least common denominator of all the fractions in the equation. The result of this operation will be a new equation, equivalent to the first, but with no fractions. This process is called clearing the equation of fractions . Let’s solve the same equation again, but this time use the method that clears the fractions.

• Find the least common denominator of all the fractions in the equation.

Multiply both sides of the equation by that LCD, 8. This clears the fractions.

Use the Distributive Property.

Simplify — and notice, no more fractions!

• Solve using the General Strategy for Solving Linear Equations.

Notice in (Figure) that once we cleared the equation of fractions, the equation was like those we solved earlier in this chapter. We changed the problem to one we already knew how to solve! We then used the General Strategy for Solving Linear Equations.

HOW TO: Solve Equations with Fraction Coefficients by Clearing the Fractions

• Multiply both sides of the equation by that LCD. This clears the fractions.

We want to clear the fractions by multiplying both sides of the equation by the LCD of all the fractions in the equation.

Multiply both sides of the equation by 12.

Distribute.

Combine like terms.

Divide by 7.

In the next example, we’ll have variables and fractions on both sides of the equation.

Find the LCD of all the fractions in the equation.

Multiply both sides by the LCD.

Simplify — no more fractions!

Subtract 2 from both sides.

Divide by 5.

In (Figure) , we’ll start by using the Distributive Property. This step will clear the fractions right away!

Simplify. Now there are no fractions to clear!

Subtract 1 from both sides.

Divide by 2.

Many times, there will still be fractions, even after distributing.

Multiply by the LCD, 4.

Collect the constants to the right.

Solve Equations with Decimal Coefficients

Multiply, and notice, no more decimals!

Add 50 to get all constants to the right.

Divide both sides by 8.

Look at the decimals and think of the equivalent fractions.

By multiplying by the LCD we will clear the decimals.

Multiply, and now no more decimals.

Divide by 19.

The next example uses an equation that is typical of the ones we will see in the money applications in the next chapter. Notice that we will distribute the decimal first before we clear all decimals in the equation.

Distribute first.

To clear decimals, multiply by 100.

Distribute and collect like terms.

Subtract 15 from both sides.

Divide by 30.

Key Concepts

Practices makes perfect.

In the following exercises, solve the equation by clearing the fractions.

In the following exercises, solve the equation by clearing the decimals.

Everyday Math

Attributions.

This chapter has been adapted from “Solve Equations with Fraction or Decimal Coefficients” in Prealgebra (OpenStax) by Lynn Marecek, MaryAnne Anthony-Smith, and Andrea Honeycutt Mathis, which is under a CC BY 4.0 Licence . Adapted by Izabela Mazur. See the Copyright page for more information.

Intermediate Algebra II Copyright © 2021 by Pooja Gupta is licensed under a Creative Commons Attribution 4.0 International License , except where otherwise noted.

Share This Book

• Mathematicians
• Math Lessons
• Square Roots
• Math Calculators

How to Solve Linear Equations with Fractions – A Step-by-Step Guide

To solve linear equations with fractions , I first clear the fractions by finding the least common denominator (LCD) and multiplying each term of the equation by this number.

This crucial step transforms the equation into a more straightforward format without fractions , which simplifies the process of isolating the variable.

Solving these equations involves the familiar steps of combining like terms and isolating the variable to one side of the equation . The goal is to determine the value of the variable that makes the equation true.

My approach ensures that the process of solving becomes a smooth transition from an equation laden with fractions to one that’s more manageable, akin to standard linear equations.

Keeping the balance between both sides of the equation is paramount as I manipulate it to find a solution. Engaging with this technique not only enhances my algebraic agility but also prepares me for tackling a broader range of mathematical problems.

Stay tuned as I unwrap this process step-by-step, ensuring you’ll confidently handle these equations on your own.

Strategies for Solving Linear Equations With Fractions

When I encounter a linear equation with fractions , I like to start by finding the least common denominator ( LCD ) of all the fractions . This strategy involves multiple steps which I will outline straightforwardly.

First, to clear the equation of fractions , I multiply every term by the LCD . For example, if I have the equation $\frac{2}{3}x + \frac{1}{4} = \frac{3}{8}x + \frac{5}{12}$, I would multiply each term by 24, the LCD of 3, 4, 8, and 12. This simplifies the equation to $16x + 6 = 9x + 10$.

Here’s how I simplify further:

Combine like terms. I bring all variables to one side and constants to the other, creating an equivalent equation . From our equation , it would look like this: $16x – 9x = 10 – 6$, which simplifies to $7x = 4$.

Solve for the variable using inverse operations . In this case, I divide both sides by 7 to isolate x: $x = \frac{4}{7}$.

In some cases, there could be variables on both sides of the equation . My approach here is to first make sure all variables and fractions are cleared before combining like terms.

Throughout this process, I check my work by ensuring each multiplication and division step maintains the balance of the equation . The left-hand side should always be equal to the right-hand side after every operation.

Let me summarize the steps in the following table for easy reference:

It’s essential to keep in mind negative numbers and to apply the multiplication property of negative ones when fractions with negative numerators or denominators appear. This helps to maintain accuracy throughout the solving equations process.

Solving linear equations with fractions doesn’t have to be intimidating. I’ve walked you through a methodical process that can make these problems much more manageable.

Remember to start by finding the least common denominator (LCD) to clear the fractions and simplify the equation into a more familiar form. By multiplying each term by the LCD, our equation looks like any other linear equation without fractions.

Once the equation is clear of fractions, you can proceed with the steps we’re all used to: isolate the variable, combine like terms, and perform operations to solve for the unknown.

It’s crucial to maintain balance by performing the same operations on both sides of the equation. If our initial problem was $\frac{3}{4}x + 2 = \frac{3}{8}x – 4$ , after clearing the fractions and simplifying we would end up with an equation like $6x + 16 = 3x – 32$ , which is straightforward to solve for $x$ .

In my experience, practicing these steps can significantly boost your confidence in handling equations with fractional or decimal coefficients.

Always double-check your work by substituting the solution back into the original equation to ensure it holds. This not only verifies your answer but also reinforces your understanding of the solving process .

By mastering these concepts, you’re not just learning to solve another type of equation; you’re enhancing your overall mathematical skill set, which will be incredibly useful in more advanced math and real-world problem-solving.

Keep practicing, stay curious, and don’t hesitate to revisit previous sections if you need a refresher on the methods used to tackle these linear equations .

• Pre Calculus
• Probability
• Sets & Set Theory
• Trigonometry

If you're seeing this message, it means we're having trouble loading external resources on our website.

If you're behind a web filter, please make sure that the domains *.kastatic.org and *.kasandbox.org are unblocked.

To log in and use all the features of Khan Academy, please enable JavaScript in your browser.

Course: 6th grade   >   Unit 7

• One-step division equations
• One-step multiplication equations
• One-step multiplication & division equations
• One-step multiplication & division equations: fractions & decimals

One-step multiplication equations: fractional coefficients

Want to join the conversation.

• Upvote Button navigates to signup page
• Downvote Button navigates to signup page
• Flag Button navigates to signup page

Video transcript

Module 10: Linear Equations

Solving equations with fraction and decimal coefficients, learning outcomes.

• Solve equations with fraction coefficients
• Solve equations with decimal coefficients

In this section, we will explore how to solve equations with fractions in them.  To start, we will review how equations with fractions can be solved by multiplying by the reciprocal. We will then move on to learn how to use the Multiplication Property of Equality to eliminate the fractions from an equation, making it simpler to solve.

Solving equations with fractions by multiplying by the reciprocal

In our first example, we will solve a one-step equation using the multiplication property of equality. You will see that the variable is part of a fraction in the given equation, and using the multiplication property of equality allows us to remove the variable from the fraction. Remember that fractions imply division, so you can think of this as the variable [latex]k[/latex] is being divided by 10. To “undo” the division, you can use multiplication to isolate [latex]k[/latex]. (Note that there is a negative term in the equation, so it will be important to think about the sign of each term as you work through the problem. Stop after each step you take to make sure all the terms have the correct sign.)

Solve [latex]-\frac{7}{2}=\frac{k}{10}[/latex] for [latex]k[/latex].

We want to isolate the [latex]k[/latex], which is being divided by [latex]10[/latex]. The first thing we should do is multiply both sides by [latex]10[/latex].

[latex]\begin{array}{l}\text{ Multiply the left side by 10: }\\\\\left(10\right)(-\frac{7}{2})=\frac{-10\cdot7}{2} = \frac{-70}{2} = -35\\\\\text{ Now, multiply the right side by 10: }\\\\\,\,\,\frac{k}{10}\left(10\right) = \frac{k\cdot10}{10} = k\\\\\text{ Now, replace your results in the equation: }\\-35=k\end{array}[/latex]

We write the [latex]k[/latex] on the left side as a matter of convention.

[latex]k=-35[/latex]

In our next example, we are given an equation that contains a variable multiplied by a fraction. We will use a reciprocal to isolate the variable.

Solve: [latex]\Large\frac{2}{3}\normalsize x=18[/latex]

Solution: Since the product of a number and its reciprocal is [latex]1[/latex], our strategy will be to isolate [latex]x[/latex] by multiplying by the reciprocal of [latex]\Large\frac{2}{3}[/latex].

Notice that we could have divided both sides of the equation [latex]\Large\frac{2}{3}\normalsize x=18[/latex] by [latex]\Large\frac{2}{3}[/latex] to isolate [latex]x[/latex]. While this would work, multiplying by the reciprocal requires fewer steps.

https://ohm.lumenlearning.com/multiembedq.php?id=141871&theme=oea&iframe_resize_id=mom22

Solving equations with fractions by clearing the denominators

You may feel overwhelmed when you see fractions in an equation, so we are going to show a method to solve equations with fractions where you use the common denominator to eliminate the fractions from an equation. The result of this operation will be a new equation, equivalent to the first, but with no fractions.

Pay attention to the fact that each term in the equation gets multiplied by the least common denominator. That’s what makes it equal to the original!

Solve: [latex]\Large\frac{1}{8}\normalsize x+\Large\frac{1}{2}=\Large\frac{1}{4}[/latex]

In the example above, the least common denominator was [latex]8[/latex]. Now it’s your turn to find an LCD, and clear the fractions before you solve these linear equations.

https://ohm.lumenlearning.com/multiembedq.php?id=71948&theme=oea&iframe_resize_id=mom1

Notice that once we cleared the equation of fractions, the equation was like those we learned how to solve earlier. We changed the problem to one we already knew how to solve!

Solve equations by clearing the Denominators

• Find the least common denominator of all the fractions in the equation.
• Multiply both sides of the equation by that LCD. This clears the fractions.
• Isolate the variable terms on one side, and the constant terms on the other side.
• Simplify both sides.
• Use the multiplication or division property to make the coefficient on the variable equal to [latex]1[/latex].

Here’s an example where you have three variable terms. After you clear fractions with the LCD, you will simplify the three variable terms, then isolate the variable.

Solve: [latex]7=\Large\frac{1}{2}\normalsize x+\Large\frac{3}{4}\normalsize x-\Large\frac{2}{3}\normalsize x[/latex]

Show Solution

Solution: We want to clear the fractions by multiplying both sides of the equation by the LCD of all the fractions in the equation.

Now here’s a similar problem for you to try. Clear the fractions, simplify, then solve.

https://ohm.lumenlearning.com/multiembedq.php?id=71948&theme=oea&iframe_resize_id=mom2

One of the most common mistakes when you clear fractions is forgetting to multiply BOTH sides of the equation by the LCD. If your answer doesn’t check, make sure you have multiplied both sides of the equation by the LCD.

In the next example, we’ll have variables and fractions on both sides of the equation. After you clear the fractions using the LCD, you will see that this equation is similar to ones with variables on both sides that we solved previously. Remember to choose a variable side and a constant side to help you organize your work.

Solve: [latex]x+\Large\frac{1}{3}=\Large\frac{1}{6}\normalsize x-\Large\frac{1}{2}[/latex]

Now you can try solving an equation with fractions that has variables on both sides of the equal sign. The answer may be a fraction.

https://ohm.lumenlearning.com/multiembedq.php?id=142514&theme=oea&iframe_resize_id=mom3

In the following video, we show another example of how to solve an equation that contains fractions and variables on both sides of the equal sign.

In the next example, we start with an equation where the variable term is locked up in parentheses and multiplied by a fraction. You can clear the fraction, or if you use the distributive property, it will eliminate the fraction.  Can you see why?

Solve: [latex]1=\Large\frac{1}{2}\normalsize\left(4x+2\right)[/latex]

Now you can try solving an equation that has the variable term in parentheses that are multiplied by a fraction.

https://ohm.lumenlearning.com/multiembedq.php?id=142542&theme=oea&iframe_resize_id=mom25

In the following video, we show how to solve a multi-step equation with fractions.

If you like to work with fractions, you can just apply your knowledge of operations with fractions and solve.  Regardless of which method you use to solve equations containing variables, you will get the same answer. You can choose the method you find the easiest! Remember to check your answer by substituting your solution into the original equation.

Solving Equations By Clearing Decimals

Some equations have decimals in them. This kind of equation will occur when we solve problems dealing with money and percent. But decimals are really another way to represent fractions. For example, [latex]0.3=\Large\frac{3}{10}[/latex] and [latex]0.17=\Large\frac{17}{100}[/latex]. So, when we have an equation with decimals, we can use the same process we used to clear fractions—multiply both sides of the equation by the least common denominator.

Solve: [latex]0.8x - 5=7[/latex]

Solution: The only decimal in the equation is [latex]0.8[/latex]. Since [latex]0.8=\Large\frac{8}{10}[/latex], the LCD is [latex]10[/latex]. We can multiply both sides by [latex]10[/latex] to clear the decimal.

Solve: [latex]0.06x+0.02=0.25x - 1.5[/latex]

Solution: Look at the decimals and think of the equivalent fractions. [latex]0.06=\Large\frac{6}{100}\normalsize ,0.02=\Large\frac{2}{100}\normalsize ,0.25=\Large\frac{25}{100}\normalsize ,1.5=1\Large\frac{5}{10}[/latex] Notice, the LCD is [latex]100[/latex]. By multiplying by the LCD we will clear the decimals.

Solve [latex]3y+10.5=6.5+2.5y[/latex] by clearing the decimals in the equation first.

Since the smallest decimal place represented in the equation is [latex]0.10[/latex], we want to multiply by [latex]10[/latex] to make  [latex]1.0[/latex] and clear the decimals from the equation.

[latex]\begin{array}{r}3y+10.5=6.5+2.5y\,\,\,\,\,\,\,\,\,\,\,\,\\\\ 10\left(3y+10.5\right)=10\left(6.5+2.5y\right)\end{array}[/latex]

Use the distributive property to expand the expressions on both sides.

[latex]\begin{array}{r}10\left(3y\right)+10\left(10.5\right)=10\left(6.5\right)+10\left(2.5y\right)\end{array}[/latex]

[latex]30y+105=65+25y[/latex]

Move the smaller variable term, [latex]25y[/latex], by subtracting it from both sides.

[latex]\begin{array}{r}30y+105=65+25y\,\,\\ \underline{-25y\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,-25y} \\5y+105=65\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\end{array}[/latex]

Subtract  [latex]105[/latex] from both sides to isolate the term with the variable.

[latex]\begin{array}{r}5y+105=\,\,65\,\,\,\\ \underline{\,\,\,\,\,\,-105\,\,\,-105} \\5y=-40\end{array}[/latex]

Divide both sides by 5 to isolate the [latex]y[/latex].

[latex]\begin{array}{l}\underline{5y}=\underline{-40}\\ 5\,\,\,\,\,\,\,\,\,\,\,\,\,5\\ \,\,\,y=-8\end{array}[/latex]

[latex]y=-8[/latex]

https://ohm.lumenlearning.com/multiembedq.php?id=71955&theme=oea&iframe_resize_id=mom2

In the following video, we present another example of how to solve an equation that contains decimals and variable terms on both sides of the equal sign.

The next example uses an equation that is typical of the ones we will see in the money applications. Note that we will distribute the decimal first, before we clear all decimals in the equation.

Solve: [latex]0.25x+0.05\left(x+3\right)=2.85[/latex]

• Question ID 142514, 142542. Authored by : Lumen Learning. License : CC BY: Attribution . License Terms : IMathAS Community License, CC-BY + GPL
• Solve a Linear Equation with Parentheses and a Fraction 2/3(9x-12)=8+2x. Authored by : James Sousa (Mathispower4u.com) for Lumen Learning. Located at : https://youtu.be/1dmEoG7DkN4 . License : CC BY: Attribution
• Ex 1: Solve an Equation with Fractions with Variable Terms on Both Sides. Authored by : James Sousa (Mathispower4u.com). Located at : https://youtu.be/G5R9jySFMpw . License : CC BY: Attribution
• Question ID 71948. Authored by : Alyson Day. License : CC BY: Attribution . License Terms : IMathAS Community License, CC-BY + GPL
• Prealgebra. Provided by : OpenStax. License : CC BY: Attribution . License Terms : Download for free at http://cnx.org/contents/[email protected]

Solving Systems Of Equations With Fractions Or Decimals

In these lessons, we help Algebra 1 students learn to solve systems of linear equations with fractions.

Related Pages Solving Systems of Equations or Simultaneous Equations using algebra Solving Simultaneous Equations Using Matrices More Lessons On Matrices Algebra Lessons

Solver Title

Generating PDF...

• Pre Algebra Order of Operations Factors & Primes Fractions Long Arithmetic Decimals Exponents & Radicals Ratios & Proportions Percent Modulo Number Line Expanded Form Mean, Median & Mode
• Algebra Equations Inequalities System of Equations System of Inequalities Basic Operations Algebraic Properties Partial Fractions Polynomials Rational Expressions Sequences Power Sums Interval Notation Pi (Product) Notation Induction Logical Sets Word Problems
• Pre Calculus Equations Inequalities Scientific Calculator Scientific Notation Arithmetics Complex Numbers Polar/Cartesian Simultaneous Equations System of Inequalities Polynomials Rationales Functions Arithmetic & Comp. Coordinate Geometry Plane Geometry Solid Geometry Conic Sections Trigonometry
• Calculus Derivatives Derivative Applications Limits Integrals Integral Applications Integral Approximation Series ODE Multivariable Calculus Laplace Transform Taylor/Maclaurin Series Fourier Series Fourier Transform
• Functions Line Equations Functions Arithmetic & Comp. Conic Sections Transformation
• Linear Algebra Matrices Vectors
• Trigonometry Identities Proving Identities Trig Equations Trig Inequalities Evaluate Functions Simplify
• Statistics Mean Geometric Mean Quadratic Mean Average Median Mode Order Minimum Maximum Probability Mid-Range Range Standard Deviation Variance Lower Quartile Upper Quartile Interquartile Range Midhinge Standard Normal Distribution
• Physics Mechanics
• Chemistry Chemical Reactions Chemical Properties
• Finance Simple Interest Compound Interest Present Value Future Value
• Economics Point of Diminishing Return
• Conversions Roman Numerals Radical to Exponent Exponent to Radical To Fraction To Decimal To Mixed Number To Improper Fraction Radians to Degrees Degrees to Radians Hexadecimal Scientific Notation Distance Weight Time Volume
• Pre Algebra
• One-Step Addition
• One-Step Subtraction
• One-Step Multiplication
• One-Step Division
• One-Step Decimals
• Two-Step Integers
• Two-Step Add/Subtract
• Two-Step Multiply/Divide
• Two-Step Fractions
• Two-Step Decimals
• Multi-Step Integers
• Multi-Step with Parentheses
• Multi-Step Rational
• Multi-Step Fractions
• Multi-Step Decimals
• Solve by Factoring
• Completing the Square
• Quadratic Formula
• Biquadratic
• Logarithmic
• Exponential
• Rational Roots
• Floor/Ceiling
• Equation Given Roots
• Newton Raphson
• Substitution
• Elimination
• Cramer's Rule
• Gaussian Elimination
• System of Inequalities
• Perfect Squares
• Difference of Squares
• Difference of Cubes
• Sum of Cubes
• Polynomials
• Distributive Property
• FOIL method
• Perfect Cubes
• Binomial Expansion
• Negative Rule
• Product Rule
• Quotient Rule
• Expand Power Rule
• Fraction Exponent
• Exponent Rules
• Exponential Form
• Logarithmic Form
• Absolute Value
• Rational Number
• Powers of i
• Complex Form
• Partial Fractions
• Is Polynomial
• Leading Coefficient
• Leading Term
• Standard Form
• Complete the Square
• Synthetic Division
• Linear Factors
• Rationalize Denominator
• Rationalize Numerator
• Identify Type
• Convergence
• Interval Notation
• Pi (Product) Notation
• Boolean Algebra
• Truth Table
• Mutual Exclusive
• Cardinality
• Caretesian Product
• Age Problems
• Distance Problems
• Cost Problems
• Investment Problems
• Number Problems
• Percent Problems
• Addition/Subtraction
• Multiplication/Division
• Dice Problems
• Coin Problems
• Card Problems
• Pre Calculus
• Linear Algebra
• Trigonometry
• Conversions

Most Used Actions

Number line.

• \frac{3}{4}x+\frac{5}{6}=5x-\frac{125}{3}
• \sqrt{2}x-\sqrt{3}=\sqrt{5}
• 7y+5-3y+1=2y+2
• \frac{x}{3}+\frac{x}{2}=10
• What is a linear equation?
• A linear equation represents a straight line on a coordinate plane. It can be written in the form: y = mx + b where m is the slope of the line and b is the y-intercept.
• How do you find the linear equation?
• To find the linear equation you need to know the slope and the y-intercept of the line. To find the slope use the formula m = (y2 - y1) / (x2 - x1) where (x1, y1) and (x2, y2) are two points on the line. The y-intercept is the point at which x=0.
• What are the 4 methods of solving linear equations?
• There are four common methods to solve a system of linear equations: Graphing, Substitution, Elimination and Matrix.
• How do you identify a linear equation?
• Here are a few ways to identify a linear equation: Look at the degree of the equation, a linear equation is a first-degree equation. Check if the equation has two variables. Graph the equation.
• What is the most basic linear equation?
• The most basic linear equation is a first-degree equation with one variable, usually written in the form of y = mx + b, where m is the slope of the line and b is the y-intercept.

linear-equation-calculator

• High School Math Solutions – Radical Equation Calculator Radical equations are equations involving radicals of any order. We will show examples of square roots; higher...

Please add a message.

Message received. Thanks for the feedback.