solving word problems involving linear equations in two variables with solution

• school Campus Bookshelves
• menu_book Bookshelves
• perm_media Learning Objects
• login Login
• how_to_reg Request Instructor Account
• hub Instructor Commons

Margin Size

• Download Page (PDF)
• Download Full Book (PDF)
• Periodic Table
• Physics Constants
• Scientific Calculator
• Reference & Cite
• Tools expand_more
• Readability

selected template will load here

This action is not available.

3.3: Applications of Linear Systems with Two Variables

• Last updated
• Save as PDF
• Page ID 6242

\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)

\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)

\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)

( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)

\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)

\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)

\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)

\( \newcommand{\Span}{\mathrm{span}}\)

\( \newcommand{\id}{\mathrm{id}}\)

\( \newcommand{\kernel}{\mathrm{null}\,}\)

\( \newcommand{\range}{\mathrm{range}\,}\)

\( \newcommand{\RealPart}{\mathrm{Re}}\)

\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)

\( \newcommand{\Argument}{\mathrm{Arg}}\)

\( \newcommand{\norm}[1]{\| #1 \|}\)

\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)

\( \newcommand{\vectorA}[1]{\vec{#1}}      % arrow\)

\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}}      % arrow\)

\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)

\( \newcommand{\vectorC}[1]{\textbf{#1}} \)

\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)

\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)

\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)

Learning Objectives

• Set up and solve applications involving relationships between two variables.
• Set up and solve mixture problems.
• Set up and solve uniform motion problems (distance problems).

Problems Involving Relationships between Two Variables

If we translate an application to a mathematical setup using two variables, then we need to form a linear system with two equations. Setting up word problems with two variables often simplifies the entire process, particularly when the relationships between the variables are not so clear.

Example \(\PageIndex{1}\):

The sum of \(4\) times a larger integer and \(5\) times a smaller integer is \(7\). When twice the smaller integer is subtracted from \(3\) times the larger, the result is \(11\). Find the integers.

Begin by assigning variables to the larger and smaller integer.

Let \(x\) represent the larger integer.

Let \(y\) represent the smaller integer.

When using two variables, we need to set up two equations. The first sentence describes a sum and the second sentence describes a difference.

This leads to the following system:

\(\left\{ \begin{array} { l } { 4 x + 5 y = 7 } \\ { 3 x - 2 y = 11 } \end{array} \right.\)

Solve using the elimination method. To eliminate the variable \(y\) multiply the first equation by \(2\) and the second by \(5\).

\(\left\{ \begin{array} { l l } { 4 x + 5y = 7 } & { \stackrel { \times2 } { \Rightarrow } } \\ { 3 x -2y = 11 } & { \stackrel { \Rightarrow } { \times 5 } } \end{array} \right. \left\{ \begin{array} { l } { 8 x + 10 y = 14 } \\ { 15 x -10y = 55 } \end{array} \right.\)

Add the equations in the equivalent system and solve for \(x\).

\(\begin{aligned} 8 x \color{red}{+ 10 y} &\color{black}{=} 14 \\ \pm 15 x \color{red}{- 10 y} & \color{black}{=} 55 \\ \hline\\ 23x & = 99\\ x & = \frac{69}{23}\\x&=3 \end{aligned}\)

Back substitute to find \(y\).

\(\begin{aligned} 4 x + 5 y & = 7 \\ 4 ( \color{OliveGreen}{3} \color{black}{)} + 5 y & = 7 \\ 12 + 5 y & = 7 \\ 5 y & = - 5 \\ y & = - 1 \end{aligned}\)

The largest integer is \(3\) and the smaller integer is \(-1\).

Exercise \(\PageIndex{1}\)

An integer is \(1\) less than twice that of another. If their sum is \(20\), find the integers.

The two integers are \(7\) and \(13\).

www.youtube.com/v/LnzO1_J4X20

Next consider applications involving simple interest and money.

A total of \($12,800\) was invested in two accounts. Part was invested in a CD at a \(3 \frac{1}{8}\)% annual interest rate and part was invested in a money market fund at a \(4 \frac{3}{4}\)% annual interest rate. If the total simple interest for one year was \($465\), then how much was invested in each account?

Begin by identifying two variables.

Let \(x\) represent the amount invested at \(3 \frac{1}{8}\)% \(= 3.125\) % \(= 0.03125\).

Let \(y\) represent the amount invested at \(4 \frac{3}{4}\)% \(= 4.75\)% = \(0.0475\).

The total amount in both accounts can be expressed as

\(x+y=12,800\)

To set up a second equation, use the fact that the total interest was \($465\). Recall that the interest for one year is the interest rate times the principal \((I = prt = pr ⋅ 1 = p)\). Use this to add the interest in both accounts. Be sure r to use the decimal equivalents for the interest rates given as percentages.

\(\begin{aligned} \color{Cerulean} { interest\: from\: the\: C D\: +\: interest\: from\: the\: fund\: =\: total\: interest } \\ 0.03125 x \quad\quad\quad +\quad\quad\:\: \quad 0.0475 y \quad\quad\quad\quad = 465\quad\quad\quad\quad\:\: \end{aligned}\)

These two equations together form the following linear system:

\(\left\{ \begin{array} { c } { x + y = 12,800 } \\ { 0.03125 x + 0.0475 y = 465 } \end{array} \right.\)

Eliminate \(x\) by multiplying the first equation by \(-0.03125\).

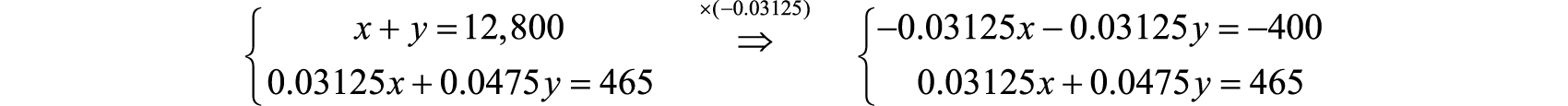
Next, add the resulting equations.

\(\begin{aligned} \color{red}{- 0.03125 x}\color{black}{ -} 0.03125 y &= - 400 \\ \pm\:\: \color{red}{0.03125 x}\color{black}{ +} 0.0475 y &= 465 \\ \hline \\0.01625y &=65 \\ y & = \frac{65}{0.01635} \\ y & = 4,000 \end{aligned}\)

Back substitute to find \(x\).

\(\begin{aligned} x + y & = 12,800 \\ x + 4000 & = 12,800 \\ x & = 8,800 \end{aligned}\)

\($4,000\) was invested at \(4 \frac{3}{4}\)% and \($8,800\) was invested at \(3 \frac{1}{8}\)%.

Example \(\PageIndex{3}\):

A jar consisting of only nickels and dimes contains \(58\) coins. If the total value is \($4.20\), how many of each coin is in the jar?

Let \(n\) represent the number of nickels in the jar.

Let \(d\) represent the number of dimes in the jar.

The total number of coins in the jar can be expressed using the following equation:

Next, use the value of each coin to determine the total value \($4.20\).

\(\begin{aligned} \color{Cerulean} {value\: of\: nickels\: + \: value\: of\: dimes\: =\: total\:value} \\ 0.05 n\quad\quad\: +\quad\:\:\: 0.10 d\quad\quad\quad = 4.20\quad\quad\quad \end{aligned}\)

This leads us to the following linear system:

\(\left\{ \begin{array} { l } { n + d = 58 } \\ { 0.05 n + 0.10 d = 4.20 } \end{array} \right.\)

Here we will solve using the substitution method. In the first equation, we can solve for \(n\).

Substitute \(n = 58 − d\) into the second equation and solve for \(d\).

\(\begin{aligned} 0.05 ( \color{Cerulean}{58 - d}\color{black}{ )} + 0.10 d & = 4.20 \\ 2.9 - 0.05 d + 0.10 d & = 4.20 \\ 2.9 + 0.05 d & = 4.20 \\ 0.05 d & = 1.3 \\ d & = 26 \end{aligned}\)

Now back substitute to find the number of nickels.

\(\begin{aligned} n & = 58 - d \\ & = 58 - 26 \\ & = 32 \end{aligned}\)

There are \(32\) nickels and \(26\) dimes in the jar.

Exercise \(\PageIndex{2}\)

Joey has a jar full of \(40\) coins consisting of only quarters and nickels. If the total value is \($5.00\), how many of each coin does Joey have?

Joey has \(15\) quarters and \(25\) nickels.

www.youtube.com/v/41bxt_tThkA

Mixture Problems

Mixture problems often include a percentage and some total amount. It is important to make a distinction between these two types of quantities. For example, if a problem states that a \(20\)-ounce container is filled with a \(2\)% saline (salt) solution, then this means that the container is filled with a mixture of salt and water as follows:

In other words, we multiply the percentage times the total to get the amount of each part of the mixture.

Example \(\PageIndex{4}\):

A \(1.8\)% saline solution is to be combined and mixed with a \(3.2\)% saline solution to produce \(35\) ounces of a \(2.2\)% saline solution. How much of each is needed?

Let \(x\) represent the amount of \(1.8\)% saline solution needed.

Let \(y\) represent the amount of \(3.2\)% saline solution needed.

The total amount of saline solution needed is \(35\) ounces. This leads to one equation,

The second equation adds up the amount of salt in the correct percentages. The amount of salt is obtained by multiplying the percentage times the amount, where the variables \(x\) and \(y\) represent the amounts of the solutions. The amount of salt in the end solution is \(2.2\)% of the \(35\) ounces, or \(.022(35)\).

\(\begin{aligned} \color {Cerulean} { salt\: in\: 1.8} \%\: \color{Cerulean}{solution } + \color{Cerulean} { salt\: in \:} 3.2 \% \color{Cerulean} { \:solution } = \color{Cerulean} { salt\: in\: the\: end\: solution } \\ 0.018 x \quad\quad\quad+ \quad\:\quad 0.032 y\quad\quad\quad\quad = \quad\quad\quad0.022 ( 35 )\quad\quad\quad \end{aligned}\)

The algebraic setup consists of both equations presented as a system:

\(\left\{ \begin{array} { c } { x + y = 35 } \\ { 0.018 x + 0.032 y = 0.022 ( 35 ) } \end{array} \right.\)

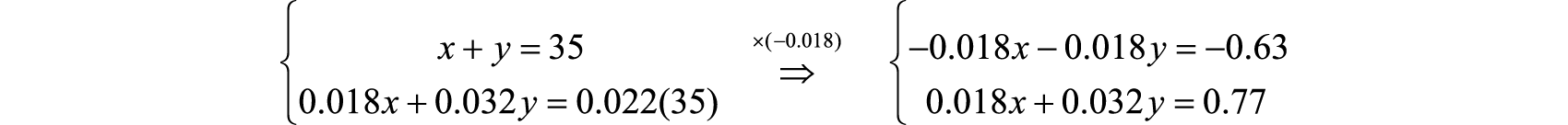
Add the resulting equations together

\(\begin{aligned} - 0.018 x - 0.018 y &= - 0.63 \\ \pm\:\: 0.018 x + 0.032 y &= 0.77 \\ \hline \\0.014y &=0.14\\y&=\frac{0.14}{0.014}\\y&=10 \end{aligned}\)

\(\begin{aligned} x + y & = 35 \\ x + \color{OliveGreen}{10} & \color{Black}{=} 35 \\ x & = 25 \end{aligned}\)

We need \(25\) ounces of the \(1.8\)% saline solution and \(10\) ounces of the \(3.2\)% saline solution.

Example \(\PageIndex{5}\):

An \(80\)% antifreeze concentrate is to be mixed with water to produce a \(48\)-liter mixture containing \(25\)% antifreeze. How much water and antifreeze concentrate is needed?

Let \(x\) represent the amount of \(80\)% antifreeze concentrate needed.

Let \(y\) represent the amount of water needed.

The total amount of the mixture must be \(48\) liters.

The second equation adds up the amount of antifreeze from each solution in the correct percentages. The amount of antifreeze in the end result is \(25\)% of \(48\) liters, or \(0.25(48)\).

\(\begin{aligned} \color {Cerulean} { antifreeze\: in\: 80} \%\: \color{Cerulean}{concentrate } + \color{Cerulean} { antrifreeze\: in \: water} = \color{Cerulean} { antifreeze\: in\: the\: end\: mixture } \\ 0.018 x \quad\quad \quad\quad\quad+ \: \quad\:\quad 0.032 y\quad\quad\quad\quad = \quad\quad\quad\quad0.022 ( 35 )\quad\quad\quad\quad\quad \end{aligned}\)

Now we can form a system of two linear equations and two variables as follows:

\(\left\{ \begin{array} { c } { x + y = 48 } \\ { 0.80 x = 0.25 ( 48 ) } \end{array} \right. \Rightarrow \left\{ \begin{array} { l } { x + y = 48 } \\ { 0.80 x = 12 } \end{array} \right.\)

Use the second equation to find \(x\):

\(\begin{aligned} 0.80 x & = 12 \\ x & = \frac { 12 } { 0.80 } \\ x & = 15 \end{aligned}\)

\(\begin{aligned} x + y & = 48 \\ \color{OliveGreen}{15} + y & = 48 \\ y & = 33 \end{aligned}\)

We need to mix \(33\) liters of water with \(15\) liters of antifreeze concentrate.

Exercise \(\PageIndex{3}\)

A chemist wishes to create \(100\) ml of a solution with \(12\)% acid content. He uses two types of stock solutions, one with \(30\)% acid content and another with \(10\)% acid content. How much of each does he need?

The chemist will need to mix \(10\) ml of the \(30\)% acid solution with \(90\) ml of the \(10\)% acid solution.

www.youtube.com/v/NXbyJNE9mWw

Uniform Motion Problems (Distance Problems)

Recall that the distance traveled is equal to the average rate times the time traveled at that rate, \(D = r ⋅ t\). These uniform motion problems usually have a lot of data, so it helps to first organize that data in a chart and then set up a linear system. In this section, you are encouraged to use two variables.

Example \(\PageIndex{6}\):

An executive traveled a total of \(4\) hours and \(875\) miles by car and by plane. Driving to the airport by car, she averaged \(50\) miles per hour. In the air, the plane averaged \(320\) miles per hour. How long did it take her to drive to the airport?

We are asked to find the time it takes her to drive to the airport; this indicates that time is the unknown quantity.

Let \(x\) represent the time it took to drive to the airport. Let \(y\) represent the time spent in the air.

Fill in the chart with the given information.

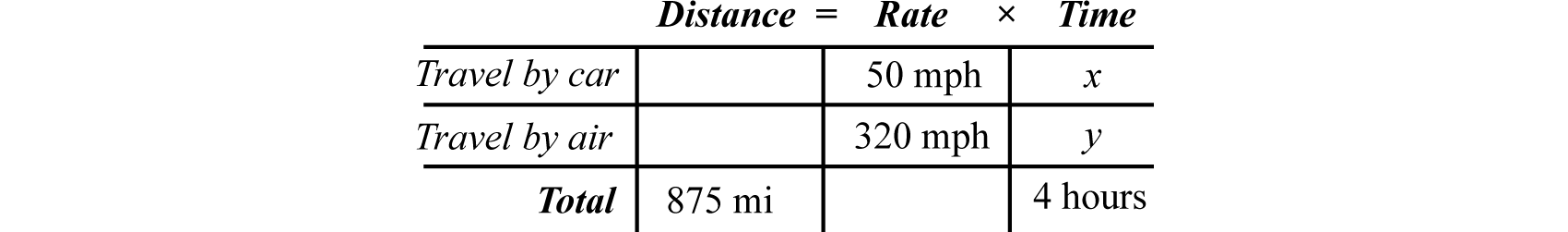
Use the formula \(D = r \cdot t\) to fill in the unknown distances.

\(\begin{array} { l } { \text { Distance traveled in the car: } D = r \cdot t = 50 \cdot x } \\ { \text { Distance traveled in the air: } D = r \cdot t = 320 \cdot y } \end{array}\)

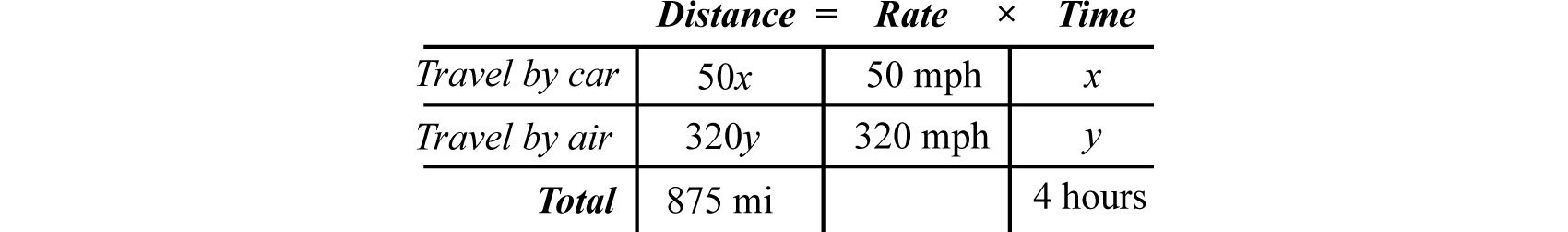
The distance column and the time column of the chart help us to set up the following linear system.

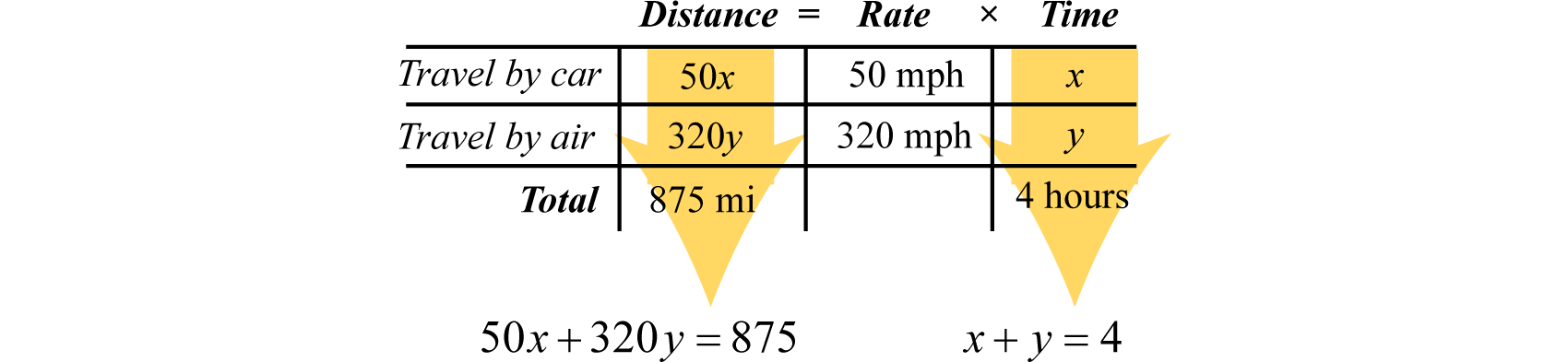
\(\left\{ \begin{array} { c } { x + y = \:4 \:\:\color{Cerulean}{\leftarrow total \:time\:traveled }} \\ { 50 x + 320 y = 875 \color{Cerulean}{\leftarrow total\: distance\: traveled } } \end{array} \right.\)

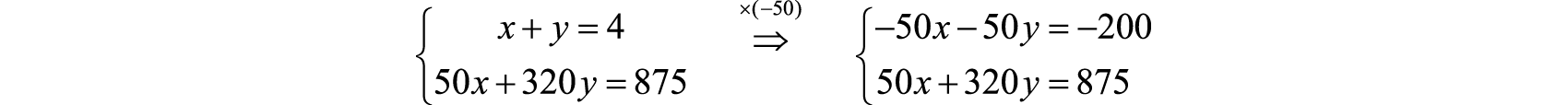
\(\begin{aligned} \color{red}{- 50 x}\color{black}{ -} 50 y& = - 200 \\ \pm\:\:\color{red}{ 50 x}\color{black}{ +} 320 y& = 875 \\ \hline\\270y&=675\\y&=\frac{675}{270}\\y&=\frac{5}{2} \end{aligned}\)

Now back substitute to find the time \(x\) it took to drive to the airport:

\(\begin{aligned} x + y & = 4 \\ x + \color{OliveGreen}{\frac { 5 } { 2 }} & \color{Black}{=} 4 \\ x & = \frac { 8 } { 2 } - \frac { 5 } { 2 } \\ x & = \frac { 3 } { 2 } \end{aligned}\)

It took her \(1 \frac{1}{2}\) hours to drive to the airport.

It is not always the case that time is the unknown quantity. Read the problem carefully and identify what you are asked to find; this defines your variables.

Example \(\PageIndex{7}\):

Flying with the wind, a light aircraft traveled \(240\) miles in \(2\) hours. The aircraft then turned against the wind and traveled another \(135\) miles in \(1 \frac{1}{2}\) hours. Find the speed of the airplane and the speed of the wind.

Begin by identifying variables.

Let \(x\) represent the speed of the airplane.

Let \(w\) represent the speed of the wind.

Use the following chart to organize the data:

With the wind, the airplane’s total speed is \(x + w\). Flying against the wind, the total speed is \(x − w\).

Use the rows of the chart along with the formula \(D = r ⋅ t\) to construct a linear system that models this problem. Take care to group the quantities that represent the rate in parentheses.

\(\left\{ \begin{array} { l } { 240 = ( x + w ) \cdot 2 \color{Cerulean}{\leftarrow distance\: traveled\: with \:the \:wind } } \\ { 135 = ( x - w ) \cdot 1.5 \color{Cerulean}{ \leftarrow distance\: traveled\: against\: the\: wind } } \end{array} \right.\)

If we divide both sides of the first equation by \(2\) and both sides of the second equation by \(1.5\), then we obtain the following equivalent system:

\(\left\{ \begin{array} { l } { 240 = ( x + w ) \cdot 2 } \quad\quad\overset{\div 2}{\Longrightarrow} \\ { 135 = ( x - w ) \cdot 1.5 \:\quad\underset{\div 1.5}{\Longrightarrow} } \end{array} \right. \quad \left\{ \begin{array} { l } { 120 = x + w } \\ { 90 = x - w } \end{array} \right.\)

Here \(w\) is lined up to eliminate.

\(\begin{aligned} x \color{red}{+ w}&\color{black}{ =} 120 \\ \pm x \color{red}{- w}&\color{black}{=} 90 \\ \hline\\2x &=210\\x & = \frac{210}{2} \\x& = 105\end{aligned}\)

Back substitute

\(\begin{aligned} x + w & = 120 \\ \color{OliveGreen}{105}\color{black}{ +} w & = 120 \\ w & = 15 \end{aligned}\)

The speed of the airplane is \(105\) miles per hour and the speed of the wind is \(15\) miles per hour.

Exercise \(\PageIndex{4}\)

A boat traveled \(27\) miles downstream in \(2\) hours. On the return trip, which was against the current, the boat was only able to travel \(21\) miles in \(2\) hours. What were the speeds of the boat and of the current?

The speed of the boat was \(12\) miles per hour and the speed of the current was \(1.5\) miles per hour.

www.youtube.com/v/EvdJQTFSUSs

Key Takeaways

• Use two variables as a means to simplify the algebraic setup of applications where the relationship between unknowns is unclear.
• Carefully read the problem several times. If two variables are used, then remember that you need to set up two linear equations in order to solve the problem.
• Be sure to answer the question in sentence form and include the correct units for the answer.

Exercise \(\PageIndex{5}\)

Set up a linear system and solve.

• The sum of two integers is \(45\). The larger integer is \(3\) less than twice the smaller. Find the two integers.
• The sum of two integers is \(126\). The larger is \(18\) less than \(5\) times the smaller. Find the two integers.
• The sum of two integers is \(41\). When \(3\) times the smaller is subtracted from the larger the result is \(17\). Find the two integers.
• The sum of two integers is \(46\). When the larger is subtracted from twice the smaller the result is \(2\). Find the two integers.
• The difference of two integers is \(11\). When twice the larger is subtracted from \(3\) times the smaller, the result is \(3\). Find the integers.
• The difference of two integers is \(6\). The sum of twice the smaller and the larger is \(72\). Find the integers.
• The sum of \(3\) times a larger integer and \(2\) times a smaller is \(15\). When \(3\) times the smaller integer is subtracted from twice the larger, the result is \(23\). Find the integers.
• The sum of twice a larger integer and \(3\) times a smaller is \(10\). When the \(4\) times the smaller integer is added to the larger, the result is \(0\). Find the integers.
• The difference of twice a smaller integer and \(7\) times a larger is \(4\). When \(5\) times the larger integer is subtracted from \(3\) times the smaller, the result is \(−5\). Find the integers.
• The difference of a smaller integer and twice a larger is \(0\). When \(3\) times the larger integer is subtracted from \(2\) times the smaller, the result is \(−5\). Find the integers.
• The length of a rectangle is \(5\) more than twice its width. If the perimeter measures \(46\) meters, then find the dimensions of the rectangle.
• The width of a rectangle is \(2\) centimeters less than one-half its length. If the perimeter measures \(62\) centimeters, then find the dimensions of the rectangle.
• A partitioned rectangular pen next to a river is constructed with a total \(136\) feet of fencing (see illustration). If the outer fencing measures \(114\) feet, then find the dimensions of the pen.

14. A partitioned rectangular pen is constructed with a total \(168\) feet of fencing (see illustration). If the perimeter measures \(138\) feet, then find the dimensions of the pen.

15. Find \(a\) and \(b\) such that the system \(\left\{ \begin{array} { l } { a x + b y = 8 } \\ { b x + a y = 7 } \end{array} \right.\) has solution \((2,1)\). (Hint: Substitute the given \(x\)- and \(y\)-values and solve the resulting linear system in terms of \(a\) and \(b\).)

16. Find \(a\) and \(b\) such that the system \(\left\{ \begin{array} { l } { a x - b y = 11 } \\ { b x + a y = 13 } \end{array} \right.\) has solution \((3, -1)\).

17. A line passes through two points \((5, −9)\) and \((−3, 7)\). Use these points and \(y = mx + b\) to construct a system of two linear equations in terms of \(m\) and \(b\) and solve it.

18. A line passes through two points \((2, 7)\) and \((\frac{1}{2}, −2)\). Use these points and \(y = mx + b\) to construct a system of two linear equations in terms of \(m\) and \(b\) and solve it.

19. A \($5,200\) principal is invested in two accounts, one earning \(3\)% interest and another earning \(6\)% interest. If the total interest for the year is \($210\), then how much is invested in each account?

20. Harry’s \($2,200\) savings is in two accounts. One account earns \(2\)% annual interest and the other earns \(4\)%. His total interest for the year is \($69\). How much does he have in each account?

21. Janine has two savings accounts totaling \($6,500\). One account earns \(2 \frac{3}{4}\)% annual interest and the other earns \(3 \frac{1}{2}\)%. If her total interest for the year is \($211\), then how much is in each account?

22. Margaret has her total savings of \($24,200\) in two different CD accounts. One CD earns \(4.6\)% interest and another earns \(3.4\)% interest. If her total interest for the year is \($1,007.60\), then how much does she have in each CD account?

23. Last year Mandy earned twice as much interest in her Money Market fund as she did in her regular savings account. The total interest from the two accounts was \($246\). How much interest did she earn in each account?

24. A small business invested \($120,000\) in two accounts. The account earning \(4\)% annual interest yielded twice as much interest as the account earning \(3\)% annual interest. How much was invested in each account?

25. Sally earns \($1,000\) per month plus a commission of \(2\)% of sales. Jane earns \($200\) per month plus \(6\)% of her sales. At what monthly sales figure will both Sally and Jane earn the same amount of pay?

26. The cost of producing specialty book shelves includes an initial set-up fee of \($1,200\) plus an additional \($20\) per unit produced. Each shelf can be sold for \($60\) per unit. Find the number of units that must be produced and sold where the costs equal the revenue generated.

27. Jim was able to purchase a pizza for \($12.35\) with quarters and dimes. If he uses \(71\) coins to buy the pizza, then how many of each did he have?

28. A cash register contains \($5\) bills and \($10\) bills with a total value of \($350\). If there are \(46\) bills total, then how many of each does the register contain?

29. Two families bought tickets for the home basketball game. One family ordered \(2\) adult tickets and \(4\) children’s tickets for a total of \($36.00\). Another family ordered \(3\) adult tickets and \(2\) children’s tickets for a total of \($32.00\). How much did each ticket cost?

30. Two friends found shirts and shorts on sale at a flea market. One bought \(4\) shirts and \(2\) shorts for a total of \($28.00\). The other bought \(3\) shirts and \(3\) shorts for a total of \($30.75\). How much was each shirt and each pair of shorts?

31. A community theater sold \(140\) tickets to the evening musical for a total of \($1,540\). Each adult ticket was sold for \($12\) and each child ticket was sold for \($8\). How many adult tickets were sold?

32. The campus bookstore sells graphing calculators for \($110\) and scientific calculators for \($16\). On the first day of classes \(50\) calculators were sold for a total of \($1,646\). How many of each were sold?

33. A jar consisting of only nickels and quarters contains \(70\) coins. If the total value is \($9.10\), how many of each coin are in the jar?

34. Jill has \($9.20\) worth of dimes and quarters. If there are \(68\) coins in total, how many of each does she have?

1. The integers are \(16\) and \(29\).

3. The integers are \(6\) and \(35\).

5. The integers are \(25\) and \(36\).

7. The integers are \(−3\) and \(7\).

9. The integers are \(−5\) and \(−2\).

11. Length: \(17\) meters; width: \(6\) meters

13. Width: \(22\) feet; length: \(70\) feet

15. \(a = 3, b = 2\)

17. \(m = −2, b = 1\)

19. \($3,400\) at \(3\)% and \($1,800\) at \(6\)%

21. \($2,200\) at \(2 \frac{3}{4}\)% and \($4,300\) at \(3 \frac{1}{2}\)%

23. Savings: \($82\); Money Market: \($164\).

25. \($20,000\)

27. \(35\) quarters and \(36\) dimes

29. Adults \($7.00\) each and children \($5.50\) each.

31. \(105\) adult tickets were sold.

33. The jar contains \(42\) nickels and \(28\) quarters.

Exercise \(\PageIndex{6}\)

• A \(17\)% acid solution is to be mixed with a \(9\)% acid solution to produce \(8\) gallons of a \(10\)% acid solution. How much of each is needed?
• A nurse wishes to obtain \(28\) ounces of a \(1.5\)% saline solution. How much of a \(1\)% saline solution must she mix with a \(4.5\)% saline solution to achieve the desired mixture?
• A customer ordered \(4\) pounds of a mixed peanut product containing \(12\)% cashews. The inventory consists of only two mixes containing \(10\)% and \(26\)% cashews. How much of each type must be mixed to fill the order?
• One alcohol solution contains \(10\)% alcohol and another contains \(25\)% alcohol. How much of each should be mixed together to obtain \(2\) gallons of a \(13.75\)% alcohol solution?
• How much cleaning fluid concentrate, with \(60\)% alcohol content, must be mixed with water to obtain a \(24\)-ounce mixture with \(15\)% alcohol content?
• How many pounds of pure peanuts must be combined with a \(20\)% peanut mix to produce \(2\) pounds of a \(50\)% peanut mix?
• A \(50\)% fruit juice concentrate can be purchased wholesale. Best taste is achieved when water is mixed with the concentrate in such a way as to obtain a \(15\)% fruit juice mixture. How much water and concentrate is needed to make a \(60\)-ounce fruit juice drink?
• Pure sugar is to be mixed with a fruit salad containing \(10\)% sugar to produce \(65\) ounces of a salad containing \(18\)% sugar. How much pure sugar is required?
• A custom aluminum alloy is created by mixing \(150\) grams of a \(15\)% aluminum alloy and \(350\) grams of a \(55\)% aluminum alloy. What percentage of aluminum is in the resulting mixture?
• A research assistant mixed \(500\) milliliters of a solution that contained a \(12\)% acid with \(300\) milliliters of water. What percentage of acid is in the resulting solution?

1. \(7\) gallons of the \(9\)% acid solution and \(1\) gallon of the \(17\)% acid solution

3. \(3.5\) pounds of the \(10\)% cashew mix and \(0.5\) pounds of the \(26\)% cashew mix

5. \(6\) ounces of cleaning fluid concentrate

7. \(18\) ounces of fruit juice concentrate and \(42\) ounces of water

Exercise \(\PageIndex{7}\)

• The two legs of a \(432\)-mile trip took \(8\) hours. The average speed for the first leg of the trip was \(52\) miles per hour and the average speed for the second leg of the trip was \(60\) miles per hour. How long did each leg of the trip take?
• Jerry took two buses on the \(265\)-mile trip from Los Angeles to Las Vegas. The first bus averaged \(55\) miles per hour and the second bus was able to average \(50\) miles per hour. If the total trip took \(5\) hours, then how long was spent in each bus?
• An executive was able to average \(48\) miles per hour to the airport in her car and then board an airplane that averaged \(210\) miles per hour. The \(549\)-mile business trip took \(3\) hours. How long did it take her to drive to the airport?
• Joe spends \(1\) hour each morning exercising by jogging and then cycling for a total of \(15\) miles. He is able to average \(6\) miles per hour jogging and \(18\) miles per hour cycling. How long does he spend jogging each morning?
• Swimming with the current Jack can swim \(2.5\) miles in \(\frac{1}{2}\) hour. Swimming back, against the same current, he can only swim \(2\) miles in the same amount of time. How fast is the current?
• A light aircraft flying with the wind can travel \(180\) miles in \(1 \frac{1}{2}\) hours. The aircraft can fly the same distance against the wind in \(2\) hours. Find the speed of the wind.
• A light airplane flying with the wind can travel \(600\) miles in \(4\) hours. On the return trip, against the wind, it will take \(5\) hours. What are the speeds of the airplane and of the wind?
• A boat can travel \(15\) miles with the current downstream in \(1 \frac{1}{4}\) hours. Returning upstream against the current, the boat can only travel \(8 \frac{3}{4}\) miles in the same amount of time. Find the speed of the current.
• Mary jogged the trail from her car to the cabin at the rate of \(6\) miles per hour. She then walked back to her car at a rate of \(4\) miles per hour. If the entire trip took \(1\) hour, then how long did it take her to walk back to her car?
• Two trains leave the station traveling in opposite directions. One train is \(8\) miles per hour faster than the other and in \(2 \frac{1}{2}\) hours they are \(230\) miles apart. Determine the average speed of each train.
• Two trains leave the station traveling in opposite directions. One train is \(12\) miles per hour faster than the other and in \(3\) hours they are \(300\) miles apart. Determine the average speed of each train.
• A jogger can sustain an average running rate of \(8\) miles per hour to his destination and \(6\) miles an hour on the return trip. Find the total distance the jogger ran if the total time running was \(1 \frac{3}{4}\) hour.

1. The first leg of the trip took \(6\) hours and the second leg took \(2\) hours.

3. It took her \(\frac{1}{2}\) hour to drive to the airport.

5. \(0.5\) miles per hour.

7. Airplane: \(135\) miles per hour; wind: \(15\) miles per hour

9. \(\frac{3}{5}\) hour

11. One train averaged \(44\) miles per hour and the other averaged \(56\) miles per hour.

Exercise \(\PageIndex{8}\)

• Compose a number or money problem of your own and share it on the discussion board.
• Compose a mixture problem of your own and share it on the discussion board.
• Compose a uniform motion problem of your own and share it on the discussion board.

1. Answer may vary

3. Answer may vary

Word Problems on Linear Equations

Worked-out word problems on linear equations with solutions explained step-by-step in different types of examples.

There are several problems which involve relations among known and unknown numbers and can be put in the form of equations. The equations are generally stated in words and it is for this reason we refer to these problems as word problems. With the help of equations in one variable, we have already practiced equations to solve some real life problems.

Steps involved in solving a linear equation word problem: ● Read the problem carefully and note what is given and what is required and what is given. ● Denote the unknown by the variables as x, y, ……. ● Translate the problem to the language of mathematics or mathematical statements. ● Form the linear equation in one variable using the conditions given in the problems. ● Solve the equation for the unknown. ● Verify to be sure whether the answer satisfies the conditions of the problem.

Step-by-step application of linear equations to solve practical word problems:

1. The sum of two numbers is 25. One of the numbers exceeds the other by 9. Find the numbers. 

Solution: Then the other number = x + 9 Let the number be x.  Sum of two numbers = 25 According to question, x + x + 9 = 25 ⇒ 2x + 9 = 25 ⇒ 2x = 25 - 9 (transposing 9 to the R.H.S changes to -9)  ⇒ 2x = 16 ⇒ 2x/2 = 16/2 (divide by 2 on both the sides)  ⇒ x = 8 Therefore, x + 9 = 8 + 9 = 17 Therefore, the two numbers are 8 and 17.

2.The difference between the two numbers is 48. The ratio of the two numbers is 7:3. What are the two numbers?  Solution:   Let the common ratio be x.  Let the common ratio be x.  Their difference = 48 According to the question,  7x - 3x = 48  ⇒ 4x = 48  ⇒ x = 48/4  ⇒ x = 12 Therefore, 7x = 7 × 12 = 84           3x = 3 × 12 = 36  Therefore, the two numbers are 84 and 36.

3. The length of a rectangle is twice its breadth. If the perimeter is 72 metre, find the length and breadth of the rectangle.  Solution: Let the breadth of the rectangle be x,  Then the length of the rectangle = 2x Perimeter of the rectangle = 72 Therefore, according to the question 2(x + 2x) = 72 ⇒ 2 × 3x = 72 ⇒ 6x = 72  ⇒ x = 72/6 ⇒ x = 12 We know, length of the rectangle = 2x                       = 2 × 12 = 24 Therefore, length of the rectangle is 24 m and breadth of the rectangle is 12 m.

4. Aaron is 5 years younger than Ron. Four years later, Ron will be twice as old as Aaron. Find their present ages. 

Solution: Let Ron’s present age be x.  Then Aaron’s present age = x - 5 After 4 years Ron’s age = x + 4, Aaron’s age x - 5 + 4.  According to the question;  Ron will be twice as old as Aaron.  Therefore, x + 4 = 2(x - 5 + 4)  ⇒ x + 4 = 2(x - 1)  ⇒ x + 4 = 2x - 2 ⇒ x + 4 = 2x - 2 ⇒ x - 2x = -2 - 4 ⇒ -x = -6 ⇒ x = 6 Therefore, Aaron’s present age = x - 5 = 6 - 5 = 1 Therefore, present age of Ron = 6 years and present age of Aaron = 1 year.

5. A number is divided into two parts, such that one part is 10 more than the other. If the two parts are in the ratio 5 : 3, find the number and the two parts.  Solution: Let one part of the number be x Then the other part of the number = x + 10 The ratio of the two numbers is 5 : 3 Therefore, (x + 10)/x = 5/3 ⇒ 3(x + 10) = 5x  ⇒ 3x + 30 = 5x ⇒ 30 = 5x - 3x ⇒ 30 = 2x  ⇒ x = 30/2  ⇒ x = 15 Therefore, x + 10 = 15 + 10 = 25 Therefore, the number = 25 + 15 = 40  The two parts are 15 and 25. 

More solved examples with detailed explanation on the word problems on linear equations.

6. Robert’s father is 4 times as old as Robert. After 5 years, father will be three times as old as Robert. Find their present ages.  Solution: Let Robert’s age be x years.  Then Robert’s father’s age = 4x After 5 years, Robert’s age = x + 5 Father’s age = 4x + 5 According to the question,  4x + 5 = 3(x + 5)  ⇒ 4x + 5 = 3x + 15  ⇒ 4x - 3x = 15 - 5  ⇒ x = 10 ⇒ 4x = 4 × 10 = 40  Robert’s present age is 10 years and that of his father’s age = 40 years.  

7. The sum of two consecutive multiples of 5 is 55. Find these multiples.  Solution: Let the first multiple of 5 be x.  Then the other multiple of 5 will be x + 5 and their sum = 55 Therefore, x + x + 5 = 55 ⇒ 2x + 5 = 55 ⇒ 2x = 55 - 5 ⇒ 2x = 50 ⇒ x = 50/2  ⇒ x = 25  Therefore, the multiples of 5, i.e., x + 5 = 25 + 5 = 30 Therefore, the two consecutive multiples of 5 whose sum is 55 are 25 and 30.  

8. The difference in the measures of two complementary angles is 12°. Find the measure of the angles.  Solution: Let the angle be x.  Complement of x = 90 - x Given their difference = 12° Therefore, (90 - x) - x = 12° ⇒ 90 - 2x = 12 ⇒ -2x = 12 - 90 ⇒ -2x = -78 ⇒ 2x/2 = 78/2 ⇒ x = 39 Therefore, 90 - x = 90 - 39 = 51  Therefore, the two complementary angles are 39° and 51°

9. The cost of two tables and three chairs is $705. If the table costs $40 more than the chair, find the cost of the table and the chair.  Solution: The table cost $ 40 more than the chair.  Let us assume the cost of the chair to be x.  Then the cost of the table = $ 40 + x The cost of 3 chairs = 3 × x = 3x and the cost of 2 tables 2(40 + x)  Total cost of 2 tables and 3 chairs = $705 Therefore, 2(40 + x) + 3x = 705 80 + 2x + 3x = 705 80 + 5x = 705 5x = 705 - 80 5x = 625/5 x = 125 and 40 + x = 40 + 125 = 165 Therefore, the cost of each chair is $125 and that of each table is $165. 

10. If 3/5 ᵗʰ of a number is 4 more than 1/2 the number, then what is the number?  Solution: Let the number be x, then 3/5 ᵗʰ of the number = 3x/5 Also, 1/2 of the number = x/2  According to the question,  3/5 ᵗʰ of the number is 4 more than 1/2 of the number.  ⇒ 3x/5 - x/2 = 4 ⇒ (6x - 5x)/10 = 4 ⇒ x/10 = 4 ⇒ x = 40 The required number is 40.  

Try to follow the methods of solving word problems on linear equations and then observe the detailed instruction on the application of equations to solve the problems.

●   Equations

What is an Equation?

What is a Linear Equation?

How to Solve Linear Equations?

Solving Linear Equations

Problems on Linear Equations in One Variable

Word Problems on Linear Equations in One Variable

Practice Test on Linear Equations

Practice Test on Word Problems on Linear Equations

●   Equations - Worksheets

Worksheet on Linear Equations

Worksheet on Word Problems on Linear Equation

7th Grade Math Problems   8th Grade Math Practice   From Word Problems on Linear Equations to HOME PAGE

Didn't find what you were looking for? Or want to know more information about Math Only Math . Use this Google Search to find what you need.

New! Comments

• Preschool Activities
• Kindergarten Math
• 1st Grade Math
• 2nd Grade Math
• 3rd Grade Math
• 4th Grade Math
• 5th Grade Math
• 6th Grade Math
• 7th Grade Math
• 8th Grade Math
• 9th Grade Math
• 10th Grade Math
• 11 & 12 Grade Math
• Concepts of Sets
• Probability
• Boolean Algebra
• Math Coloring Pages
• Multiplication Table
• Cool Maths Games
• Math Flash Cards
• Online Math Quiz
• Math Puzzles
• Binary System
• Math Dictionary
• Conversion Chart
• Homework Sheets
• Math Problem Ans
• Free Math Answers
• Printable Math Sheet
• Funny Math Answers
• Employment Test
• Math Patterns
• Link Partners
• Privacy Policy

Recent Articles

Numbers | Notation | Numeration | Numeral | Estimation | Examples

May 12, 24 06:28 PM

Face Value and Place Value|Difference Between Place Value & Face Value

May 12, 24 06:23 PM

Patterns in Numbers | Patterns in Maths |Math Patterns|Series Patterns

May 12, 24 06:09 PM

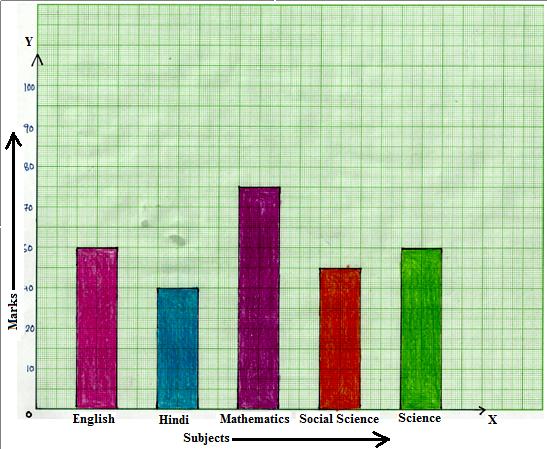
Worksheet on Bar Graphs | Bar Graphs or Column Graphs | Graphing Bar

May 12, 24 04:59 PM

Worksheet on Pictographs | Picture Graph Worksheets | Pictograph Works

May 12, 24 04:31 PM

© and ™ math-only-math.com. All Rights Reserved. 2010 - 2024.

Word Problems Linear Equations

Andymath.com features free videos, notes, and practice problems with answers! Printable pages make math easy. Are you ready to be a mathmagician?

\(\textbf{1)}\) Joe and Steve are saving money. Joe starts with $105 and saves $5 per week. Steve starts with $5 and saves $15 per week. After how many weeks do they have the same amount of money? Show Equations \(y= 5x+105,\,\,\,y=15x+5\) Show Answer 10 weeks ($155)

\(\textbf{2)}\) mike and sarah collect rocks. together they collected 50 rocks. mike collected 10 more rocks than sarah. how many rocks did each of them collect show equations \(m+s=50,\,\,\,m=s+10\) show answer mike collected 30 rocks, sarah collected 20 rocks., \(\textbf{3)}\) in a classroom the ratio of boys to girls is 2:3. there are 25 students in the class. how many are girls show equations \(b+g=50,\,\,\,3b=2g\) show answer 15 girls (10 boys), \(\textbf{4)}\) kyle makes sandals at home. the sandal making tools cost $100 and he spends $10 on materials for each sandal. he sells each sandal for $30. how many sandals does he have to sell to break even show equations \(c=10x+100,\,\,\,r=30x\) show answer 5 sandals ($150), \(\textbf{5)}\) molly is throwing a beach party. she still needs to buy beach towels and beach balls. towels are $3 each and beachballs are $4 each. she bought 10 items in total and it cost $34. how many beach balls did she get show equations show answer 4 beachballs (6 towels), \(\textbf{6)}\) anna volunteers at a pet shelter. they have cats and dogs. there are 36 pets in total at the shelter, and the ratio of dogs to cats is 4:5. how many cats are at the shelter show equations \(c+d=40,\,\,\,5d=4c\) show answer 20 cats (16 dogs), \(\textbf{7)}\) a store sells oranges and apples. oranges cost $1.00 each and apples cost $2.00 each. in the first sale of the day, 15 fruits were sold in total, and the price was $25. how many of each type of frust was sold show equations \(o+a=15,\,\,\,1o+2a=25\) show answer 10 apples and 5 oranges, \(\textbf{8)}\) the ratio of red marbles to green marbles is 2:7. there are 36 marbles in total. how many are red show equations \(r+g=36,\,\,\,7r=2g\) show answer 8 red marbles (28 green marbles), \(\textbf{9)}\) a tennis club charges $100 to join the club and $10 for every hour using the courts. write an equation to express the cost \(c\) in terms of \(h\) hours playing tennis. show equation the equation is \(c=10h+100\), \(\textbf{10)}\) emma and liam are saving money. emma starts with $80 and saves $10 per week. liam starts with $120 and saves $6 per week. after how many weeks will they have the same amount of money show equations \(e = 10x + 80,\,\,\,l = 6x + 120\) show answer 10 weeks ($180 each), \(\textbf{11)}\) mark and lisa collect stamps. together they collected 200 stamps. mark collected 40 more stamps than lisa. how many stamps did each of them collect show equations \(m + l = 200,\,\,\,m = l + 40\) show answer mark collected 120 stamps, lisa collected 80 stamps., \(\textbf{12)}\) in a classroom, the ratio of boys to girls is 3:5. there are 40 students in the class. how many are boys show equations \(b + g = 40,\,\,\,5b = 3g\) show answer 15 boys (25 girls), \(\textbf{13)}\) lisa is selling handmade jewelry. the materials cost $60, and she sells each piece for $20. how many pieces does she have to sell to break even show equations \(c=60,\,\,\,r=20x\) show answer 3 pieces, \(\textbf{14)}\) tom is buying books and notebooks for school. books cost $15 each, and notebooks cost $3 each. he bought 12 items in total, and it cost $120. how many notebooks did he buy show equations \(b + n = 12,\,\,\,15b+3n=120\) show answer 5 notebooks (7 books), \(\textbf{15)}\) emily volunteers at an animal shelter. they have rabbits and guinea pigs. there are 36 animals in total at the shelter, and the ratio of guinea pigs to rabbits is 4:5. how many guinea pigs are at the shelter show equations \(r + g = 36,\,\,\,5g=4r\) show answer 16 guinea pigs (20 rabbits), \(\textbf{16)}\) mike and sarah are going to a theme park. mike’s ticket costs $40, and sarah’s ticket costs $30. they also bought $20 worth of food. how much did they spend in total show equations \(m + s + f = t,\,\,\,m=40,\,\,\,s=30,\,\,\,f=20\) show answer they spent $90 in total., \(\textbf{17)}\) the ratio of red marbles to blue marbles is 2:3. there are 50 marbles in total. how many are blue show equations \(r + b = 50,\,\,\,3r=2b\) show answer 30 blue marbles (20 red marbles), \(\textbf{18)}\) a pizza restaurant charges $12 for a large pizza and $8 for a small pizza. if a customer buys 5 pizzas in total, and it costs $52, how many large pizzas did they buy show equations \(l + s = 5,\,\,\,12l+8s=52\) show answer they bought 3 large pizzas (2 small pizzas)., \(\textbf{19)}\) the area of a rectangle is 48 square meters. if the length is 8 meters, what is the width of the rectangle show equations \(a=l\times w,\,\,\,l=8,\,\,\,a=48\) show answer the width is 6 meters., \(\textbf{20)}\) two numbers have a sum of 50. one number is 10 more than the other. what are the two numbers show equations \(x+y=50,\,\,\,x=y+10\) show answer the numbers are 30 and 20., \(\textbf{21)}\) a store sells jeans for $40 each and t-shirts for $20 each. in the first sale of the day, they sold 8 items in total, and the price was $260. how many of each type of item was sold show equations \(j+t=8,\,\,\,40j+20t=260\) show answer 5 jeans and 3 t-shirts were sold., \(\textbf{22)}\) the ratio of apples to carrots is 3:4. there are 28 fruits in total. how many are apples show equations \(\)a+c=28,\,\,\,4a=3c show answer there are 12 apples and 16 carrots., \(\textbf{23)}\) a phone plan costs $30 per month, and there is an additional charge of $0.10 per minute for calls. write an equation to express the cost \(c\) in terms of \(m\) minutes. show equation the equation is \(\)c=30+0.10m, \(\textbf{24)}\) a triangle has a base of 8 inches and a height of 6 inches. calculate its area. show equations \(a=0.5\times b\times h,\,\,\,b=8,\,\,\,h=6\) show answer the area is 24 square inches., \(\textbf{25)}\) a store sells shirts for $25 each and pants for $45 each. in the first sale of the day, 4 items were sold, and the price was $180. how many of each type of item was sold show equations \(t+p=4,\,\,\,25t+45p=180\) show answer 0 shirts and 4 pants were sold., \(\textbf{26)}\) a garden has a length of 12 feet and a width of 10 feet. calculate its area. show equations \(a=l\times w,\,\,\,l=12,\,\,\,w=10\) show answer the area is 120 square feet., \(\textbf{27)}\) the sum of two consecutive odd numbers is 56. what are the two numbers show equations \(x+y=56,\,\,\,x=y+2\) show answer the numbers are 27 and 29., \(\textbf{28)}\) a toy store sells action figures for $15 each and toy cars for $5 each. in the first sale of the day, 10 items were sold, and the price was $110. how many of each type of item was sold show equations \(a+c=10,\,\,\,15a+5c=110\) show answer 6 action figures and 4 toy cars were sold., \(\textbf{29)}\) a bakery sells pie for $2 each and cookies for $1 each. in the first sale of the day, 14 items were sold, and the price was $25. how many of each type of item was sold show equations \(p+c=14,\,\,\,2p+c=25\) show answer 11 pies and 3 cookies were sold., \(\textbf{for 30-33}\) two car rental companies charge the following values for x miles. car rental a: \(y=3x+150 \,\,\) car rental b: \(y=4x+100\), \(\textbf{30)}\) which rental company has a higher initial fee show answer company a has a higher initial fee, \(\textbf{31)}\) which rental company has a higher mileage fee show answer company b has a higher mileage fee, \(\textbf{32)}\) for how many driven miles is the cost of the two companies the same show answer the companies cost the same if you drive 50 miles., \(\textbf{33)}\) what does the \(3\) mean in the equation for company a show answer for company a, the cost increases by $3 per mile driven., \(\textbf{34)}\) what does the \(100\) mean in the equation for company b show answer for company b, the initial cost (0 miles driven) is $100., \(\textbf{for 35-39}\) andy is going to go for a drive. the formula below tells how many gallons of gas he has in his car after m miles. \(g=12-\frac{m}{18}\), \(\textbf{35)}\) what does the \(12\) in the equation represent show answer andy has \(12\) gallons in his car when he starts his drive., \(\textbf{36)}\) what does the \(18\) in the equation represent show answer it takes \(18\) miles to use up \(1\) gallon of gas., \(\textbf{37)}\) how many miles until he runs out of gas show answer the answer is \(216\) miles, \(\textbf{38)}\) how many gallons of gas does he have after 90 miles show answer the answer is \(7\) gallons, \(\textbf{39)}\) when he has \(3\) gallons remaining, how far has he driven show answer the answer is \(162\) miles, \(\textbf{for 40-42}\) joe sells paintings. each month he makes no commission on the first $5,000 he sells but then makes a 10% commission on the rest., \(\textbf{40)}\) find the equation of how much money x joe needs to sell to earn y dollars per month. show answer the answer is \(y=.1(x-5,000)\), \(\textbf{41)}\) how much does joe need to sell to earn $10,000 in a month. show answer the answer is \($105,000\), \(\textbf{42)}\) how much does joe earn if he sells $45,000 in a month show answer the answer is \($4,000\), see related pages\(\), \(\bullet\text{ word problems- linear equations}\) \(\,\,\,\,\,\,\,\,\), \(\bullet\text{ word problems- averages}\) \(\,\,\,\,\,\,\,\,\), \(\bullet\text{ word problems- consecutive integers}\) \(\,\,\,\,\,\,\,\,\), \(\bullet\text{ word problems- distance, rate and time}\) \(\,\,\,\,\,\,\,\,\), \(\bullet\text{ word problems- break even}\) \(\,\,\,\,\,\,\,\,\), \(\bullet\text{ word problems- ratios}\) \(\,\,\,\,\,\,\,\,\), \(\bullet\text{ word problems- age}\) \(\,\,\,\,\,\,\,\,\), \(\bullet\text{ word problems- mixtures and concentration}\) \(\,\,\,\,\,\,\,\,\), linear equations are a type of equation that has a linear relationship between two variables, and they can often be used to solve word problems. in order to solve a word problem involving a linear equation, you will need to identify the variables in the problem and determine the relationship between them. this usually involves setting up an equation (or equations) using the given information and then solving for the unknown variables . linear equations are commonly used in real-life situations to model and analyze relationships between different quantities. for example, you might use a linear equation to model the relationship between the cost of a product and the number of units sold, or the relationship between the distance traveled and the time it takes to travel that distance. linear equations are typically covered in a high school algebra class. these types of problems can be challenging for students who are new to algebra, but they are an important foundation for more advanced math concepts. one common mistake that students make when solving word problems involving linear equations is failing to set up the problem correctly. it’s important to carefully read the problem and identify all of the relevant information, as well as any given equations or formulas that you might need to use. other related topics involving linear equations include graphing and solving systems. understanding linear equations is also useful for applications in fields such as economics, engineering, and physics., about andymath.com, andymath.com is a free math website with the mission of helping students, teachers and tutors find helpful notes, useful sample problems with answers including step by step solutions, and other related materials to supplement classroom learning. if you have any requests for additional content, please contact andy at [email protected] . he will promptly add the content. topics cover elementary math , middle school , algebra , geometry , algebra 2/pre-calculus/trig , calculus and probability/statistics . in the future, i hope to add physics and linear algebra content. visit me on youtube , tiktok , instagram and facebook . andymath content has a unique approach to presenting mathematics. the clear explanations, strong visuals mixed with dry humor regularly get millions of views. we are open to collaborations of all types, please contact andy at [email protected] for all enquiries. to offer financial support, visit my patreon page. let’s help students understand the math way of thinking thank you for visiting. how exciting.

Systems of Linear Equations and Word Problems

Note that we saw how to solve linear inequalities here in the Coordinate System and Graphing Lines section . Note also that we solve Algebra Word Problems without Systems here , and we solve systems using matrices in the Matrices and Solving Systems with Matrices  section here.

Introduction to Systems

“Systems of equations” just means that we are dealing with more than one equation and variable. So far, we’ve basically just played around with the equation for a line, which is $ y=mx+b$. Let’s say we have the following situation:

Now, you can always do “guess and check” to see what would work, but you might as well use algebra! It’s much better to learn the algebra way, because even though this problem is fairly simple to solve, the algebra way will let you solve any algebra problem – even the really complicated ones.

The first trick in problems like this is to figure out what we want to know. This will help us decide what variables (unknowns) to use. What we want to know is how many pairs of jeans we want to buy (let’s say “$ j$”) and how many dresses we want to buy (let’s say “$ d$”). Always write down what your variables will be:

Let $ j=$ the number of jeans you will buy Let $ d=$ the number of dresses you’ll buy

Like we did before, let’s translate word-for-word from math to English:

Now we have the 2 equations as shown below. Notice that the $ j$ variable is just like the $ x$ variable and the $ d$ variable is just like the $ y$. It’s easier to put in $ j$   and $ d$ so we can remember what they stand for when we get the answers.

This is what we call a system, since we have to solve for more than one variable – we have to solve for 2 here. The cool thing is to solve for 2   variables , you typically need 2   equations , to solve for 3   variables , you need 3   equations , and so on. That’s easy to remember, right?

We need to get an answer that works in both equations ; this is what we’re doing when we’re solving; this is called solving simultaneous systems , or solving system simultaneously . There are several ways to solve systems; we’ll talk about graphing first.

Solving Systems by Graphing

Remember that when you graph a line, you see all the different coordinates (or $ x/y$ combinations) that make the equation work. In systems, you have to make both equations work, so the intersection of the two lines shows the point that fits both equations (assuming the lines do in fact intersect; we’ll talk about that later).  The points of intersections satisfy both equations simultaneously. 

Put these equations into the $ y=mx+b$ ($ d=mj+b$) format, by solving for the $ d$ (which is like the $ y$):

$ \displaystyle j+d=6;\text{ }\,\text{ }\text{solve for }d:\text{ }d=-j+6\text{ }$

$ \displaystyle 25j+50d=200;\text{ }\,\,\text{solve for }d:\text{ }d=\frac{{200-25j}}{{50}}=-\frac{1}{2}j+4$

Now graph both lines:

Note that with non-linear equations, there will most likely be more than one intersection; an example of how to get more than one solution via the Graphing Calculator can be found in the  Exponents and Radicals in Algebra section. Also, t here are some examples of systems of inequality  here in the Coordinate System and Graphing Lines section .

Solving Systems with Substitution

Substitution is the favorite way to solve for many students! It involves exactly what it says: substituting one variable in another equation so that you only have one variable in that equation. Here is the same problem:

You’re going to the mall with your friends and you have $200 to spend from your recent birthday money. You discover a store that has all jeans for $25 and all dresses for $50 .  You really, really want to take home 6 items of clothing because you “need” that many new things. How many pairs of jeans and how many dresses you can buy so you use the whole $200 (tax not included)?

Below are our two equations, and let’s solve for “$ d$” in terms of “$ j$” in the first equation. Then, let’s substitute what we got for “$ d$” into the next equation. Even though it doesn’t matter which equation you start with, remember to always pick the “easiest” equation first (one that we can easily solve for a variable) to get a variable by itself.

We could buy 4 pairs of jeans and 2 dresses . Note that we could have also solved for “$ j$” first; it really doesn’t matter. You’ll want to pick the variable that’s most easily solved for. Let’s try another substitution problem that’s a little bit different:

Solving Systems with Linear Combination or Elimination

Probably the most useful way to solve systems is using linear combination, or linear elimination. The reason it’s most useful is that usually in real life we don’t have one variable in terms of another (in other words, a “$ y=$” situation).

The main purpose of the linear combination method is to add or subtract the equations so that one variable is eliminated. We can add, subtract, or multiply both sides of equations by the same numbers – let’s use real numbers as shown below. We are using the Additive Property of Equality , Subtraction Property of Equality , Multiplicative Property of Equality , and/or Division Property of Equality that we saw here in the Types of Numbers and Algebraic Properties section :

If we have a set of 2 equations with 2 unknowns, for example, we can manipulate them by adding, multiplying or subtracting (we usually prefer adding) so that we get one equation with one variable. Let’s use our previous problem:

We could buy 4 pairs of jeans and 2 dresses .

Here’s another example:

Types of equations

In the example above, we found one unique solution to the set of equations. Sometimes, however, for a set of equations, there are no solutions (when lines are parallel) or an infinite number of solutions or infinitely many solutions (when the two lines are actually the same line, and one is just a “multiple” of the other).

When there is at least one solution , the equations are consistent equations , since they have a solution. When there is only one solution, the system is called independent , since they cross at only one point. When equations have infinite solutions, they are the same equation, are consistent , and are called dependent or coincident (think of one just sitting on top of the other).

When equations have no solutions , they are called inconsistent equations , since we can never get a solution . 

Here are graphs of inconsistent and dependent equations that were created on a graphing calculator:

Systems with Three Equations

Let’s get a little more complicated with systems; in real life, we rarely just have two unknowns to solve for.

Let’s say at the same store, they also had pairs of shoes for $20 and we managed to get $60 more to spend! Now we have a new problem. To spend the even $260 , how many pairs of jeans, dresses, and pairs of shoes should we get if want, for example, exactly 10 total items (Remember that jeans cost $25 each and dresses cost $50 each).

Let’s let $ j=$ the number of pair of jeans, $ d=$ the number of dresses, and $ s=$ the number of pairs of shoes we should buy. So far, we’ll have the following equations:

$ \displaystyle \begin{array}{c}j+d+s=10\text{ }\\25j+\text{ }50d+\,20s=260\end{array}$

We’ll need another equation, since for three variables, we need three equations (otherwise, we theoretically might have infinite ways to solve the problem). In this type of problem, you would also need something like this: We want twice as many pairs of jeans as pairs of shoes . Now, since we have the same number of equations as variables , we can potentially get one solution for the system of equations. Here are the three equations:

We’ll learn later how to put these in our calculator to easily solve using matrices (see the  Matrices and Solving Systems with Matrices section). For now, we can use two equations at a time to eliminate a variable (using substitution and/or elimination), and keep doing this until we’ve solved for all variables. These can get really difficult to solve, but remember that in “real life”, there are computers to do all this work!

Remember again, that if we ever get to a point where we end up with something like this, it means there are an infinite number of solutions : $ 4=4$  (variables are gone and a number equals another number and they are the same). And if we up with something like this, it means there are no solutions : $ 5=2$ (variables are gone and two numbers are left and they don’t equal each other).

Let’s solve our system:      $ \displaystyle \begin{array}{c}j+d+s=10\text{ }\\25j+\text{ }50d+20s=260\\j=2s\end{array}$ :

We could buy 6 pairs of jeans, 1 dress, and 3 pairs of shoes .

Here’s one more example of a three-variable system of equations, where we’ll only use linear elimination:

$ \displaystyle \begin{align}5x-6y-\,7z\,&=\,7\\6x-4y+10z&=\,-34\\2x+4y-\,3z\,&=\,29\end{align}$

I know – this is really difficult stuff! But if you do it step-by-step and keep using the equations you need with the right variables, you can do it. Think of it like a puzzle – you may not know exactly where you’re going, but do what you can in baby steps, and you’ll get there (sort of like life sometimes, right?!). And we’ll learn much easier ways to do these types of problems.

Algebra Word Problems with Systems

Let’s do more word problems; you’ll notice that many of these are the same type that we did earlier in the Algebra Word Problems  section , but now we can use more than one variable. This will actually make the problems easier! Again, when doing these word problems:

• If you’re wondering what the variables (or unknowns) should be when working on a word problem, look at what the problem is asking. These are usually (but not always) what your variables are!
• If you’re not sure how to set up the equations, use regular numbers (simple ones!) and see what you’re doing. Then put the variables back in!

Here are some problems:

Investment Word Problem

We also could have set up this problem with a table:

Mixture Word Problems

Here’s a mixture word problem . With mixture problems, remember if the problem calls for a pure solution or concentrate , use 100% (if the percentage is that solution) or 0% (if the percentage is another solution).

Let’s do the math (use substitution )!

$ \displaystyle \begin{array}{c}x\,\,+\,\,y=10\\.01x+.035y=10(.02)\end{array}$          $ \displaystyle \begin{array}{c}\,y=10-x\\.01x+.035(10-x)=.2\\.01x\,+\,.35\,\,-\,.035x=.2\\\,-.025x=-.15;\,\,\,\,\,x=6\\\,y=10-6=4\end{array}$

We would need 6 liters of the 1% milk, and 4 liters of the 3.5% milk.

Here’s another mixture problem:

$ \displaystyle \begin{array}{c}x+y=50\\8x+4y=50\left( {6.4} \right)\end{array}$                   $ \displaystyle \begin{array}{c}y=50-x\\8x+4\left( {50-x} \right)=320\\8x+200-4x=320\\4x=120\,;\,\,\,\,x=30\\y=50-30=20\\8x+4y=50(6.4)\end{array}$

We would need 30 pounds of the $8 coffee bean, and 20 pounds of the $4 coffee bean. See how similar this problem is to the one where we use percentages?

Distance Word Problem:

Here’s a distance word problem using systems ; distance problems have to do with an object’s speed, time, and distance. Note that, as well as the distance word problem here in the Algebra Word Problems section , there’s an example of a Parametric Distance Problem here in the Parametric Equations section .

Which Plumber Problem

Many word problems you’ll have to solve have to do with an initial charge or setup charge, and a charge or rate per time period. In these cases, the initial charge will be the $ \boldsymbol {y}$ -intercept , and the  rate will be the slope . Here is an example:

Geometry Word Problem:

Many times, we’ll have a geometry problem as an algebra word problem; these might involve perimeter, area, or sometimes angle measurements (so don’t forget these things!). Let’s do one involving angle measurements.

See – these are getting easier! Here’s one that’s a little tricky though:

Work Problem : 

Let’s do a “ work problem ” that is typically seen when studying Rational Equations (fraction with variables in them) and can be found here in the Rational Functions, E quations and Inequalities  section .

Note that there’s also a simpler version of this problem here in the Direct, Inverse, Joint and Combined Variation section .

Three Variable Word Problem:

Let’s do one more with three equations and three unknowns:

The “Candy” Problem

Sometimes we get lucky and can solve a system of equations where we have more unknowns (variables) then equations. (Actually, I think it’s not so much luck, but having good problem writers!) Here’s one like that:

There are more Systems Word Problems in the  Matrices and Solving Systems with Matrices section , Linear Programming section , and Right Triangle Trigonometry section .

Understand these problems, and practice, practice, practice!

For Practice : Use the Mathway  widget below to try a  Systems of Equations  problem. Click on Submit (the blue arrow to the right of the problem) and click on Solve by Substitution or Solve by Addition/Elimination  to see the answer .

You can also type in your own problem, or click on the three dots in the upper right hand corner and click on “Examples” to drill down by topic.

If you click on Tap to view steps , or Click Here , you can register at Mathway for a free trial , and then upgrade to a paid subscription at any time (to get any type of math problem solved!).

On to Algebraic Functions, including Domain and Range   – you’re ready! 

7.1 Systems of Linear Equations: Two Variables

Learning objectives.

In this section, you will:

• Solve systems of equations by graphing.
• Solve systems of equations by substitution.
• Solve systems of equations by addition.
• Identify inconsistent systems of equations containing two variables.
• Express the solution of a system of dependent equations containing two variables.

A skateboard manufacturer introduces a new line of boards. The manufacturer tracks its costs, which is the amount it spends to produce the boards, and its revenue, which is the amount it earns through sales of its boards. How can the company determine if it is making a profit with its new line? How many skateboards must be produced and sold before a profit is possible? In this section, we will consider linear equations with two variables to answer these and similar questions.

Introduction to Systems of Equations

In order to investigate situations such as that of the skateboard manufacturer, we need to recognize that we are dealing with more than one variable and likely more than one equation. A system of linear equations consists of two or more linear equations made up of two or more variables such that all equations in the system are considered simultaneously. To find the unique solution to a system of linear equations, we must find a numerical value for each variable in the system that will satisfy all equations in the system at the same time. Some linear systems may not have a solution and others may have an infinite number of solutions. In order for a linear system to have a unique solution, there must be at least as many equations as there are variables. Even so, this does not guarantee a unique solution.

In this section, we will look at systems of linear equations in two variables, which consist of two equations that contain two different variables. For example, consider the following system of linear equations in two variables.

The solution to a system of linear equations in two variables is any ordered pair that satisfies each equation independently. In this example, the ordered pair (4, 7) is the solution to the system of linear equations. We can verify the solution by substituting the values into each equation to see if the ordered pair satisfies both equations. Shortly we will investigate methods of finding such a solution if it exists.

In addition to considering the number of equations and variables, we can categorize systems of linear equations by the number of solutions. A consistent system of equations has at least one solution. A consistent system is considered to be an independent system if it has a single solution, such as the example we just explored. The two lines have different slopes and intersect at one point in the plane. A consistent system is considered to be a dependent system if the equations have the same slope and the same y -intercepts. In other words, the lines coincide so the equations represent the same line. Every point on the line represents a coordinate pair that satisfies the system. Thus, there are an infinite number of solutions.

Another type of system of linear equations is an inconsistent system , which is one in which the equations represent two parallel lines. The lines have the same slope and different y- intercepts. There are no points common to both lines; hence, there is no solution to the system.

Types of Linear Systems

There are three types of systems of linear equations in two variables, and three types of solutions.

• An independent system has exactly one solution pair ( x , y ) . ( x , y ) . The point where the two lines intersect is the only solution.
• An inconsistent system has no solution. Notice that the two lines are parallel and will never intersect.
• A dependent system has infinitely many solutions. The lines are coincident. They are the same line, so every coordinate pair on the line is a solution to both equations.

Figure 2 compares graphical representations of each type of system.

Given a system of linear equations and an ordered pair, determine whether the ordered pair is a solution.

• Substitute the ordered pair into each equation in the system.
• Determine whether true statements result from the substitution in both equations; if so, the ordered pair is a solution.

Determining Whether an Ordered Pair Is a Solution to a System of Equations

Determine whether the ordered pair ( 5 , 1 ) ( 5 , 1 ) is a solution to the given system of equations.

Substitute the ordered pair ( 5 , 1 ) ( 5 , 1 ) into both equations.

The ordered pair ( 5 , 1 ) ( 5 , 1 ) satisfies both equations, so it is the solution to the system.

We can see the solution clearly by plotting the graph of each equation. Since the solution is an ordered pair that satisfies both equations, it is a point on both of the lines and thus the point of intersection of the two lines. See Figure 3 .

Determine whether the ordered pair ( 8 , 5 ) ( 8 , 5 ) is a solution to the following system.

Solving Systems of Equations by Graphing

There are multiple methods of solving systems of linear equations. For a system of linear equations in two variables, we can determine both the type of system and the solution by graphing the system of equations on the same set of axes.

Solving a System of Equations in Two Variables by Graphing

Solve the following system of equations by graphing. Identify the type of system.

Solve the first equation for y . y .

Solve the second equation for y . y .

Graph both equations on the same set of axes as in Figure 4 .

The lines appear to intersect at the point ( −3, −2 ) . ( −3, −2 ) . We can check to make sure that this is the solution to the system by substituting the ordered pair into both equations.

The solution to the system is the ordered pair ( −3, −2 ) , ( −3, −2 ) , so the system is independent.

Solve the following system of equations by graphing.

Can graphing be used if the system is inconsistent or dependent?

Yes, in both cases we can still graph the system to determine the type of system and solution. If the two lines are parallel, the system has no solution and is inconsistent. If the two lines are identical, the system has infinite solutions and is a dependent system.

Solving Systems of Equations by Substitution

Solving a linear system in two variables by graphing works well when the solution consists of integer values, but if our solution contains decimals or fractions, it is not the most precise method. We will consider two more methods of solving a system of linear equations that are more precise than graphing. One such method is solving a system of equations by the substitution method , in which we solve one of the equations for one variable and then substitute the result into the second equation to solve for the second variable. Recall that we can solve for only one variable at a time, which is the reason the substitution method is both valuable and practical.

Given a system of two equations in two variables, solve using the substitution method.

• Solve one of the two equations for one of the variables in terms of the other.
• Substitute the expression for this variable into the second equation, then solve for the remaining variable.
• Substitute that solution into either of the original equations to find the value of the first variable. If possible, write the solution as an ordered pair.
• Check the solution in both equations.

Solving a System of Equations in Two Variables by Substitution

Solve the following system of equations by substitution.

First, we will solve the first equation for y . y .

Now we can substitute the expression x −5 x −5 for y y in the second equation.

Now, we substitute x = 8 x = 8 into the first equation and solve for y . y .

Our solution is ( 8 , 3 ) . ( 8 , 3 ) .

Check the solution by substituting ( 8 , 3 ) ( 8 , 3 ) into both equations.

Can the substitution method be used to solve any linear system in two variables?

Yes, but the method works best if one of the equations contains a coefficient of 1 or –1 so that we do not have to deal with fractions.

Solving Systems of Equations in Two Variables by the Addition Method

A third method of solving systems of linear equations is the addition method . In this method, we add two terms with the same variable, but opposite coefficients, so that the sum is zero. Of course, not all systems are set up with the two terms of one variable having opposite coefficients. Often we must adjust one or both of the equations by multiplication so that one variable will be eliminated by addition.

Given a system of equations, solve using the addition method.

• Write both equations with x - and y -variables on the left side of the equal sign and constants on the right.
• Write one equation above the other, lining up corresponding variables. If one of the variables in the top equation has the opposite coefficient of the same variable in the bottom equation, add the equations together, eliminating one variable. If not, use multiplication by a nonzero number so that one of the variables in the top equation has the opposite coefficient of the same variable in the bottom equation, then add the equations to eliminate the variable.
• Solve the resulting equation for the remaining variable.
• Substitute that value into one of the original equations and solve for the second variable.
• Check the solution by substituting the values into the other equation.

Solving a System by the Addition Method

Solve the given system of equations by addition.

Both equations are already set equal to a constant. Notice that the coefficient of x x in the second equation, –1, is the opposite of the coefficient of x x in the first equation, 1. We can add the two equations to eliminate x x without needing to multiply by a constant.

Now that we have eliminated x , x , we can solve the resulting equation for y . y .

Then, we substitute this value for y y into one of the original equations and solve for x . x .

The solution to this system is ( − 7 3 , 2 3 ) . ( − 7 3 , 2 3 ) .

Check the solution in the first equation.

We gain an important perspective on systems of equations by looking at the graphical representation. See Figure 5 to find that the equations intersect at the solution. We do not need to ask whether there may be a second solution because observing the graph confirms that the system has exactly one solution.

Using the Addition Method When Multiplication of One Equation Is Required

Solve the given system of equations by the addition method .

Adding these equations as presented will not eliminate a variable. However, we see that the first equation has 3 x 3 x in it and the second equation has x . x . So if we multiply the second equation by −3 , −3 , the x -terms will add to zero.

Now, let’s add them.

For the last step, we substitute y = −4 y = −4 into one of the original equations and solve for x . x .

Our solution is the ordered pair ( 3 , −4 ) . ( 3 , −4 ) . See Figure 6 . Check the solution in the original second equation.

Solve the system of equations by addition.

Using the Addition Method When Multiplication of Both Equations Is Required

Solve the given system of equations in two variables by addition.

One equation has 2 x 2 x and the other has 5 x . 5 x . The least common multiple is 10 x 10 x so we will have to multiply both equations by a constant in order to eliminate one variable. Let’s eliminate x x by multiplying the first equation by −5 −5 and the second equation by 2. 2.

Then, we add the two equations together.

Substitute y = −4 y = −4 into the original first equation.

The solution is ( −2 , −4 ) . ( −2 , −4 ) . Check it in the other equation.

See Figure 7 .

Using the Addition Method in Systems of Equations Containing Fractions

First clear each equation of fractions by multiplying both sides of the equation by the least common denominator.

Now multiply the second equation by −1 −1 so that we can eliminate the x -variable.

Add the two equations to eliminate the x -variable and solve the resulting equation.

Substitute y = 7 y = 7 into the first equation.

The solution is ( 11 2 , 7 ) . ( 11 2 , 7 ) . Check it in the other equation.

Identifying Inconsistent Systems of Equations Containing Two Variables

Now that we have several methods for solving systems of equations, we can use the methods to identify inconsistent systems. Recall that an inconsistent system consists of parallel lines that have the same slope but different y y -intercepts. They will never intersect. When searching for a solution to an inconsistent system, we will come up with a false statement, such as 12 = 0. 12 = 0.

Solving an Inconsistent System of Equations

Solve the following system of equations.

We can approach this problem in two ways. Because one equation is already solved for x , x , the most obvious step is to use substitution.

Clearly, this statement is a contradiction because 9 ≠ 13. 9 ≠ 13. Therefore, the system has no solution.

The second approach would be to first manipulate the equations so that they are both in slope-intercept form. We manipulate the first equation as follows.

We then convert the second equation expressed to slope-intercept form.

Comparing the equations, we see that they have the same slope but different y -intercepts. Therefore, the lines are parallel and do not intersect.

Writing the equations in slope-intercept form confirms that the system is inconsistent because all lines will intersect eventually unless they are parallel. Parallel lines will never intersect; thus, the two lines have no points in common. The graphs of the equations in this example are shown in Figure 8 .

Solve the following system of equations in two variables.

Expressing the Solution of a System of Dependent Equations Containing Two Variables

Recall that a dependent system of equations in two variables is a system in which the two equations represent the same line. Dependent systems have an infinite number of solutions because all of the points on one line are also on the other line. After using substitution or addition, the resulting equation will be an identity, such as 0 = 0. 0 = 0.

Finding a Solution to a Dependent System of Linear Equations

Find a solution to the system of equations using the addition method .

With the addition method, we want to eliminate one of the variables by adding the equations. In this case, let’s focus on eliminating x . x . If we multiply both sides of the first equation by −3 , −3 , then we will be able to eliminate the x x -variable.

Now add the equations.

We can see that there will be an infinite number of solutions that satisfy both equations.

If we rewrote both equations in the slope-intercept form, we might know what the solution would look like before adding. Let’s look at what happens when we convert the system to slope-intercept form.

See Figure 9 . Notice the results are the same. The general solution to the system is ( x , − 1 3 x + 2 3 ) . ( x , − 1 3 x + 2 3 ) .

Using Systems of Equations to Investigate Profits

Using what we have learned about systems of equations, we can return to the skateboard manufacturing problem at the beginning of the section. The skateboard manufacturer’s revenue function is the function used to calculate the amount of money that comes into the business. It can be represented by the equation R = x p , R = x p , where x = x = quantity and p = p = price. The revenue function is shown in orange in Figure 10 .

The cost function is the function used to calculate the costs of doing business. It includes fixed costs, such as rent and salaries, and variable costs, such as utilities. The cost function is shown in blue in Figure 10 . The x x -axis represents quantity in hundreds of units. The y -axis represents either cost or revenue in hundreds of dollars.

The point at which the two lines intersect is called the break-even point . We can see from the graph that if 700 units are produced, the cost is $3,300 and the revenue is also $3,300. In other words, the company breaks even if they produce and sell 700 units. They neither make money nor lose money.

The shaded region to the right of the break-even point represents quantities for which the company makes a profit. The shaded region to the left represents quantities for which the company suffers a loss. The profit function is the revenue function minus the cost function, written as P ( x ) = R ( x ) − C ( x ) . P ( x ) = R ( x ) − C ( x ) . Clearly, knowing the quantity for which the cost equals the revenue is of great importance to businesses.

Finding the Break-Even Point and the Profit Function Using Substitution

Given the cost function C ( x ) = 0.85 x + 35,000 C ( x ) = 0.85 x + 35,000 and the revenue function R ( x ) = 1.55 x , R ( x ) = 1.55 x , find the break-even point and the profit function.

Write the system of equations using y y to replace function notation.

Substitute the expression 0.85 x + 35,000 0.85 x + 35,000 from the first equation into the second equation and solve for x . x .

Then, we substitute x = 50,000 x = 50,000 into either the cost function or the revenue function.

The break-even point is ( 50,000 , 77,500 ) . ( 50,000 , 77,500 ) .

The profit function is found using the formula P ( x ) = R ( x ) − C ( x ) . P ( x ) = R ( x ) − C ( x ) .

The profit function is P ( x ) = 0.7 x −35,000. P ( x ) = 0.7 x −35,000.

The cost to produce 50,000 units is $77,500, and the revenue from the sales of 50,000 units is also $77,500. To make a profit, the business must produce and sell more than 50,000 units. See Figure 11 .

We see from the graph in Figure 12 that the profit function has a negative value until x = 50,000 , x = 50,000 , when the graph crosses the x -axis. Then, the graph emerges into positive y -values and continues on this path as the profit function is a straight line. This illustrates that the break-even point for businesses occurs when the profit function is 0. The area to the left of the break-even point represents operating at a loss.

Writing and Solving a System of Equations in Two Variables

The cost of a ticket to the circus is $ 25.00 $ 25.00 for children and $ 50.00 $ 50.00 for adults. On a certain day, attendance at the circus is 2,000 2,000 and the total gate revenue is $ 70,000. $ 70,000. How many children and how many adults bought tickets?

Let c = the number of children and a = the number of adults in attendance.

The total number of people is 2,000. 2,000. We can use this to write an equation for the number of people at the circus that day.

The revenue from all children can be found by multiplying $ 25.00 $ 25.00 by the number of children, 25 c . 25 c . The revenue from all adults can be found by multiplying $ 50.00 $ 50.00 by the number of adults, 50 a . 50 a . The total revenue is $ 70,000. $ 70,000. We can use this to write an equation for the revenue.

We now have a system of linear equations in two variables.

In the first equation, the coefficient of both variables is 1. We can quickly solve the first equation for either c c or a . a . We will solve for a . a .

Substitute the expression 2,000 − c 2,000 − c in the second equation for a a and solve for c . c .

Substitute c = 1,200 c = 1,200 into the first equation to solve for a . a .

We find that 1,200 1,200 children and 800 800 adults bought tickets to the circus that day.

Meal tickets at the circus cost $ 4.00 $ 4.00 for children and $ 12.00 $ 12.00 for adults. If 1,650 1,650 meal tickets were bought for a total of $ 14,200 , $ 14,200 , how many children and how many adults bought meal tickets?

Access these online resources for additional instruction and practice with systems of linear equations.

• Solving Systems of Equations Using Substitution
• Solving Systems of Equations Using Elimination
• Applications of Systems of Equations

7.1 Section Exercises

Can a system of linear equations have exactly two solutions? Explain why or why not.

If you are performing a break-even analysis for a business and their cost and revenue equations are dependent, explain what this means for the company’s profit margins.

If you are solving a break-even analysis and get a negative break-even point, explain what this signifies for the company?

If you are solving a break-even analysis and there is no break-even point, explain what this means for the company. How should they ensure there is a break-even point?

Given a system of equations, explain at least two different methods of solving that system.

For the following exercises, determine whether the given ordered pair is a solution to the system of equations.

5 x − y = 4 x + 6 y = 2 5 x − y = 4 x + 6 y = 2 and ( 4 , 0 ) ( 4 , 0 )

−3 x − 5 y = 13 − x + 4 y = 10 −3 x − 5 y = 13 − x + 4 y = 10 and ( −6 , 1 ) ( −6 , 1 )

3 x + 7 y = 1 2 x + 4 y = 0 3 x + 7 y = 1 2 x + 4 y = 0 and ( 2 , 3 ) ( 2 , 3 )

−2 x + 5 y = 7 2 x + 9 y = 7 −2 x + 5 y = 7 2 x + 9 y = 7 and ( −1 , 1 ) ( −1 , 1 )

x + 8 y = 43 3 x −2 y = −1 x + 8 y = 43 3 x −2 y = −1 and ( 3 , 5 ) ( 3 , 5 )

For the following exercises, solve each system by substitution.

x + 3 y = 5 2 x + 3 y = 4 x + 3 y = 5 2 x + 3 y = 4

3 x −2 y = 18 5 x + 10 y = −10 3 x −2 y = 18 5 x + 10 y = −10

4 x + 2 y = −10 3 x + 9 y = 0 4 x + 2 y = −10 3 x + 9 y = 0

2 x + 4 y = −3.8 9 x −5 y = 1.3 2 x + 4 y = −3.8 9 x −5 y = 1.3

− 2 x + 3 y = 1.2 − 3 x − 6 y = 1.8 − 2 x + 3 y = 1.2 − 3 x − 6 y = 1.8

x −0.2 y = 1 −10 x + 2 y = 5 x −0.2 y = 1 −10 x + 2 y = 5

3 x + 5 y = 9 30 x + 50 y = −90 3 x + 5 y = 9 30 x + 50 y = −90

−3 x + y = 2 12 x −4 y = −8 −3 x + y = 2 12 x −4 y = −8

1 2 x + 1 3 y = 16 1 6 x + 1 4 y = 9 1 2 x + 1 3 y = 16 1 6 x + 1 4 y = 9

− 1 4 x + 3 2 y = 11 − 1 8 x + 1 3 y = 3 − 1 4 x + 3 2 y = 11 − 1 8 x + 1 3 y = 3

For the following exercises, solve each system by addition.

−2 x + 5 y = −42 7 x + 2 y = 30 −2 x + 5 y = −42 7 x + 2 y = 30

6 x −5 y = −34 2 x + 6 y = 4 6 x −5 y = −34 2 x + 6 y = 4

5 x − y = −2.6 −4 x −6 y = 1.4 5 x − y = −2.6 −4 x −6 y = 1.4

7 x −2 y = 3 4 x + 5 y = 3.25 7 x −2 y = 3 4 x + 5 y = 3.25

−x + 2 y = −1 5 x −10 y = 6 −x + 2 y = −1 5 x −10 y = 6

7 x + 6 y = 2 −28 x −24 y = −8 7 x + 6 y = 2 −28 x −24 y = −8

5 6 x + 1 4 y = 0 1 8 x − 1 2 y = − 43 120 5 6 x + 1 4 y = 0 1 8 x − 1 2 y = − 43 120

1 3 x + 1 9 y = 2 9 − 1 2 x + 4 5 y = − 1 3 1 3 x + 1 9 y = 2 9 − 1 2 x + 4 5 y = − 1 3

−0.2 x + 0.4 y = 0.6 x −2 y = −3 −0.2 x + 0.4 y = 0.6 x −2 y = −3

−0.1 x + 0.2 y = 0.6 5 x −10 y = 1 −0.1 x + 0.2 y = 0.6 5 x −10 y = 1

For the following exercises, solve each system by any method.

5 x + 9 y = 16 x + 2 y = 4 5 x + 9 y = 16 x + 2 y = 4

6 x −8 y = −0.6 3 x + 2 y = 0.9 6 x −8 y = −0.6 3 x + 2 y = 0.9

5 x −2 y = 2.25 7 x −4 y = 3 5 x −2 y = 2.25 7 x −4 y = 3

x − 5 12 y = − 55 12 −6 x + 5 2 y = 55 2 x − 5 12 y = − 55 12 −6 x + 5 2 y = 55 2

7 x −4 y = 7 6 2 x + 4 y = 1 3 7 x −4 y = 7 6 2 x + 4 y = 1 3

3 x + 6 y = 11 2 x + 4 y = 9 3 x + 6 y = 11 2 x + 4 y = 9

7 3 x − 1 6 y = 2 − 21 6 x + 3 12 y = −3 7 3 x − 1 6 y = 2 − 21 6 x + 3 12 y = −3

1 2 x + 1 3 y = 1 3 3 2 x + 1 4 y = − 1 8 1 2 x + 1 3 y = 1 3 3 2 x + 1 4 y = − 1 8

2.2 x + 1.3 y = −0.1 4.2 x + 4.2 y = 2.1 2.2 x + 1.3 y = −0.1 4.2 x + 4.2 y = 2.1

0.1 x + 0.2 y = 2 0.35 x −0.3 y = 0 0.1 x + 0.2 y = 2 0.35 x −0.3 y = 0

For the following exercises, graph the system of equations and state whether the system is consistent, inconsistent, or dependent and whether the system has one solution, no solution, or infinite solutions.

3 x − y = 0.6 x −2 y = 1.3 3 x − y = 0.6 x −2 y = 1.3

− x + 2 y = 4 2 x −4 y = 1 − x + 2 y = 4 2 x −4 y = 1

x + 2 y = 7 2 x + 6 y = 12 x + 2 y = 7 2 x + 6 y = 12

3 x −5 y = 7 x −2 y = 3 3 x −5 y = 7 x −2 y = 3

3 x −2 y = 5 −9 x + 6 y = −15 3 x −2 y = 5 −9 x + 6 y = −15

For the following exercises, use the intersect function on a graphing device to solve each system. Round all answers to the nearest hundredth.

0.1 x + 0.2 y = 0.3 −0.3 x + 0.5 y = 1 0.1 x + 0.2 y = 0.3 −0.3 x + 0.5 y = 1

−0.01 x + 0.12 y = 0.62 0.15 x + 0.20 y = 0.52 −0.01 x + 0.12 y = 0.62 0.15 x + 0.20 y = 0.52

0.5 x + 0.3 y = 4 0.25 x −0.9 y = 0.46 0.5 x + 0.3 y = 4 0.25 x −0.9 y = 0.46

0.15 x + 0.27 y = 0.39 −0.34 x + 0.56 y = 1.8 0.15 x + 0.27 y = 0.39 −0.34 x + 0.56 y = 1.8

−0.71 x + 0.92 y = 0.13 0.83 x + 0.05 y = 2.1 −0.71 x + 0.92 y = 0.13 0.83 x + 0.05 y = 2.1

For the following exercises, solve each system in terms of A , B , C , D , E , A , B , C , D , E , and F F where A – F A – F are nonzero numbers. Note that A ≠ B A ≠ B and A E ≠ B D . A E ≠ B D .

x + y = A x − y = B x + y = A x − y = B

x + A y = 1 x + B y = 1 x + A y = 1 x + B y = 1

A x + y = 0 B x + y = 1 A x + y = 0 B x + y = 1

A x + B y = C x + y = 1 A x + B y = C x + y = 1

A x + B y = C D x + E y = F A x + B y = C D x + E y = F

Real-World Applications

For the following exercises, solve for the desired quantity.

A stuffed animal business has a total cost of production C = 12 x + 30 C = 12 x + 30 and a revenue function R = 20 x . R = 20 x . Find the break-even point.

An Ethiopian restaurant has a cost of production C ( x ) = 11 x + 120 C ( x ) = 11 x + 120 and a revenue function R ( x ) = 5 x . R ( x ) = 5 x . When does the company start to turn a profit?

A cell phone factory has a cost of production C ( x ) = 150 x + 10 , 000 C ( x ) = 150 x + 10 , 000 and a revenue function R ( x ) = 200 x . R ( x ) = 200 x . What is the break-even point?

A musician charges C ( x ) = 64 x + 20,000 C ( x ) = 64 x + 20,000 where x x is the total number of attendees at the concert. The venue charges $80 per ticket. After how many people buy tickets does the venue break even, and what is the value of the total tickets sold at that point?

A guitar factory has a cost of production C ( x ) = 75 x + 50,000. C ( x ) = 75 x + 50,000. If the company needs to break even after 150 units sold, at what price should they sell each guitar? Round up to the nearest dollar, and write the revenue function.

For the following exercises, use a system of linear equations with two variables and two equations to solve.

Find two numbers whose sum is 28 and difference is 13.

A number is 9 more than another number. Twice the sum of the two numbers is 10. Find the two numbers.

The startup cost for a restaurant is $120,000, and each meal costs $10 for the restaurant to make. If each meal is then sold for $15, after how many meals does the restaurant break even?

A moving company charges a flat rate of $150, and an additional $5 for each box. If a taxi service would charge $20 for each box, how many boxes would you need for it to be cheaper to use the moving company, and what would be the total cost?

A total of 1,595 first- and second-year college students gathered at a pep rally. The number of first-years exceeded the number of second-years by 15. How many students from each year group were in attendance?

276 students enrolled in an introductory chemistry class. By the end of the semester, 5 times the number of students passed as failed. Find the number of students who passed, and the number of students who failed.

There were 130 faculty at a conference. If there were 18 more women than men attending, how many of each gender attended the conference?

A jeep and a pickup truck enter a highway running east-west at the same exit heading in opposite directions. The jeep entered the highway 30 minutes before the pickup did, and traveled 7 mph slower than the pickup. After 2 hours from the time the pickup entered the highway, the cars were 306.5 miles apart. Find the speed of each car, assuming they were driven on cruise control and retained the same speed.

If a scientist mixed 10% saline solution with 60% saline solution to get 25 gallons of 40% saline solution, how many gallons of 10% and 60% solutions were mixed?

An investor earned triple the profits of what they earned last year. If they made $500,000.48 total for both years, how much did the investor earn in profits each year?

An investor invested 1.1 million dollars into two land investments. On the first investment, Swan Peak, her return was a 110% increase on the money she invested. On the second investment, Riverside Community, she earned 50% over what she invested. If she earned $1 million in profits, how much did she invest in each of the land deals?

If an investor invests a total of $25,000 into two bonds, one that pays 3% simple interest, and the other that pays 2 7 8 % 2 7 8 % interest, and the investor earns $737.50 annual interest, how much was invested in each account?

If an investor invests $23,000 into two bonds, one that pays 4% in simple interest, and the other paying 2% simple interest, and the investor earns $710.00 annual interest, how much was invested in each account?

Blu-rays cost $5.96 more than regular DVDs at All Bets Are Off Electronics. How much would 6 Blu-rays and 2 DVDs cost if 5 Blu-rays and 2 DVDs cost $127.73?

A store clerk sold 60 pairs of sneakers. The high-tops sold for $98.99 and the low-tops sold for $129.99. If the receipts for the two types of sales totaled $6,404.40, how many of each type of sneaker were sold?

A concert manager counted 350 ticket receipts the day after a concert. The price for a student ticket was $12.50, and the price for an adult ticket was $16.00. The register confirms that $5,075 was taken in. How many student tickets and adult tickets were sold?

Admission into an amusement park for 4 children and 2 adults is $116.90. For 6 children and 3 adults, the admission is $175.35. Assuming a different price for children and adults, what is the price of the child’s ticket and the price of the adult ticket?

As an Amazon Associate we earn from qualifying purchases.

This book may not be used in the training of large language models or otherwise be ingested into large language models or generative AI offerings without OpenStax's permission.

Want to cite, share, or modify this book? This book uses the Creative Commons Attribution License and you must attribute OpenStax.

Access for free at https://openstax.org/books/college-algebra-2e/pages/1-introduction-to-prerequisites

• Authors: Jay Abramson
• Publisher/website: OpenStax
• Book title: College Algebra 2e
• Publication date: Dec 21, 2021
• Location: Houston, Texas
• Book URL: https://openstax.org/books/college-algebra-2e/pages/1-introduction-to-prerequisites
• Section URL: https://openstax.org/books/college-algebra-2e/pages/7-1-systems-of-linear-equations-two-variables

© Jan 9, 2024 OpenStax. Textbook content produced by OpenStax is licensed under a Creative Commons Attribution License . The OpenStax name, OpenStax logo, OpenStax book covers, OpenStax CNX name, and OpenStax CNX logo are not subject to the Creative Commons license and may not be reproduced without the prior and express written consent of Rice University.

Linear Equations in Two Variables

A linear equation in two variables is an equation in which two variables have the exponent 1. A system of equations with two variables has a unique solution, no solutions, or infinitely many solutions. A linear system of equations may have 'n' number of variables. An important thing to keep in mind while solving linear equations with n number of variables is that there must be n equations to solve and determine the value of variables.

Linear equations in two variables are the algebraic equations which are of the form (or can be converted to the form) y = mx + b, where m is the slope and b is the y-intercept . They are the equations of the first order. For example, y = 2x + 3 and 2y = 4x + 9 are two-variable linear equations.

What are Linear Equations in Two Variables?

The linear equations in two variables are the equations in which each of the two variables is of the highest order ( exponent ) of 1 and may have one, none, or infinitely many solutions. The standard form of a two-variable linear equation is ax + by + c = 0 where x and y are the two variables. The solutions can also be written in ordered pairs like (x, y). The graphical representation of the pairs of linear equations in two variables includes two straight lines which could be:

• intersecting lines
• parallel lines or
• coincident lines .

Forms of Linear Equations in Two Variables

A linear equation in two variables can be in different forms like standard form , intercept form and point-slope form . For example, the same equation 2x + 3y=9 can be represented in each of the forms like 2x + 3y - 9=0 (standard form), y = (-2/3)x + 3 ( slope-intercept form ), and y - 5/3 = -2/3(x + (-2)) (point-slope form). Look at the image given below showing all these three forms of representing linear equations in two variables with examples.

The system of equations means the collection of equations and they are also referred to as simultaneous linear equations . We will learn how to solve pair of linear equations in two variables using different methods.

Solving Pairs of Linear Equations in Two Variables

There are five methods to solve pairs of linear equations in two variables as shown below:

• Graphical Method
• Substitution Method
• Cross Multiplication Method
• Elimination Method

Determinant Method

Graphical method for solving linear equations in two variables.

The steps to solve linear equations in two variables graphically are given below:

• Step 1 : To solve a system of two equations in two variables graphically , we graph each equation. To know how, click here or follow steps 2 and 3 below.
• Step 2 : To graph an equation manually, first convert it to the form y = mx+b by solving the equation for y.
• Step 3 : Start putting the values of x as 0, 1, 2, and so on and find the corresponding values of y, or vice-versa.
• Step 4 : Identify the point where both lines meet.
• Step 5 : The point of intersection is the solution of the given system.

Example: Find the solution of the following system of equations graphically.

Solution: We will graph them and see whether they intersect at a point. As you can see below, both lines meet at (1, 2). Thus, the solution of the given system of linear equations is x=1 and y=2.

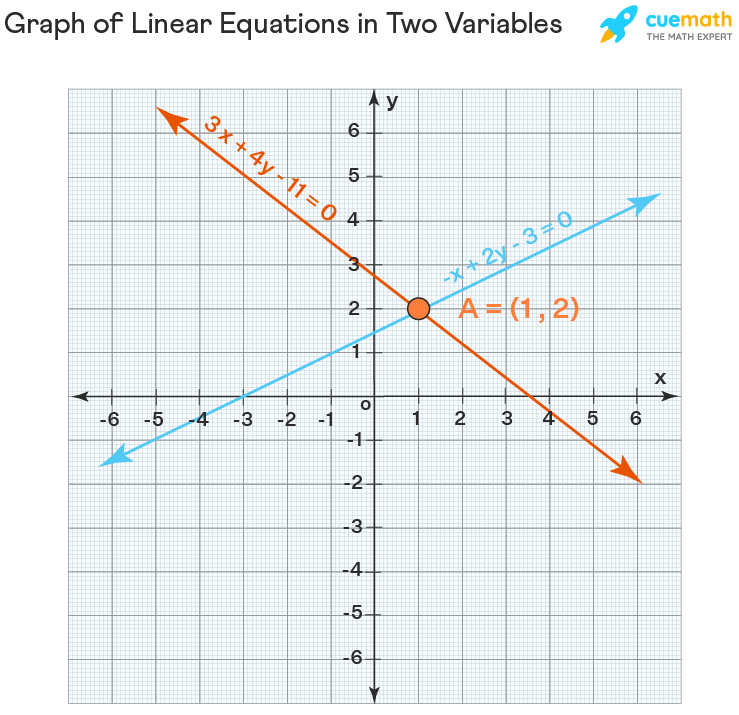
But both lines may not intersect always. Sometimes they may be parallel. In that case, the pairs of linear equations in two variables have no solution. In some other cases, both lines coincide with each other. In that case, each point on that line is a solution of the given system and hence the given system has an infinite number of solutions.

Consistent and Inconsistent System of Linear Equations:

• If the system has a solution, then it is said to be consistent;
• otherwise, it is said to be inconsistent.

Independent and Dependent System of Linear Equations:

• If the system has a unique solution, then it is independent.
• If it has an infinite number of solutions, then it is dependent. It means that one variable depends on the other.

Consider a system of two linear equations: a 1 x + b 1 y + c 1 = 0 and a 2 x + b 2 y + c 2 = 0. Here we can understand when a linear system with two variables is consistent/inconsistent and independent/dependent.

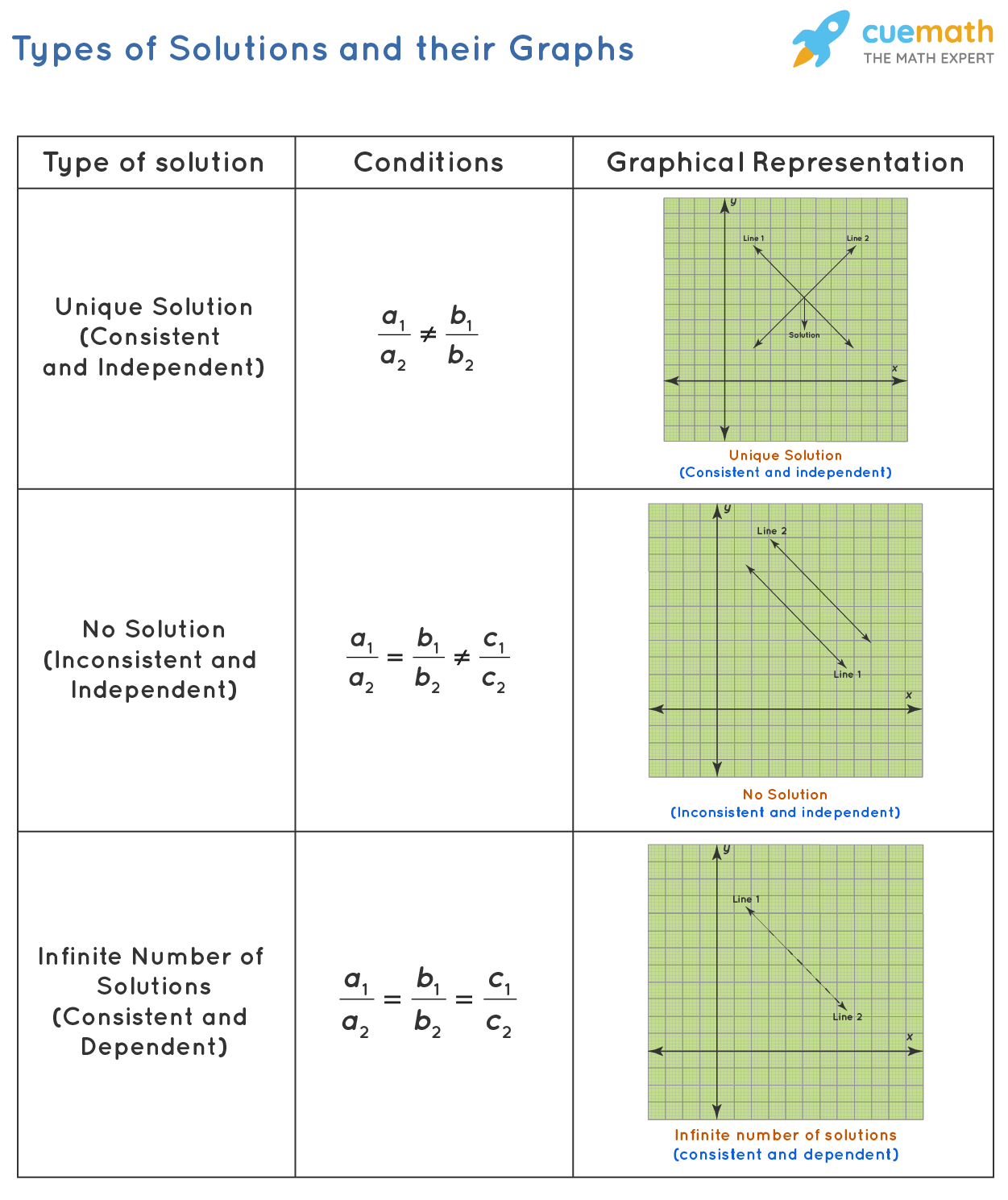
Method of Substitution

To solve a system of two linear equations in two variables using the substitution method , we have to use the steps given below:

• Step 1: Solve one of the equations for one variable.
• Step 2: Substitute this in the other equation to get an equation in terms of a single variable.
• Step 3: Solve it for the variable.
• Step 4: Substitute it in any of the equations to get the value of another variable.

Example: Solve the following system of equations using the substitution method. x+2y-7=0 2x-5y+13=0

Solution: Let us solve the equation, x+2y-7=0 for y: x+2y-7=0 ⇒2y=7-x ⇒ y=(7-x)/2

Substitute this in the equation, 2x-5y+13=0:

2x-5y+13=0 ⇒ 2x-5((7-x)/2)+13=0 ⇒ 2x-(35/2)+(5x/2)+13=0 ⇒ 2x + (5x/2) = 35/2 - 13 ⇒ 9x/2 = 9/2 ⇒ x=1

Substitute x=1 this in the equation y=(7-x)/2:

y=(7-1)/2 = 3

Therefore, the solution of the given system is x=1 and y=3.

Consider a system of linear equations: a 1 x + b 1 y + c 1 = 0 and a 2 x + b 2 y + c 2 = 0.

To solve this using the cross multiplication method , we first write the coefficients of each of x and y and constants as follows:

Here, the arrows indicate that those coefficients have to be multiplied. Now we write the following equation by cross-multiplying and subtracting the products. \(\dfrac{x}{b_{1} c_{2}-b_{2} c_{1}}=\dfrac{y}{c_{1} a_{2}-c_{2} a_{1}}=\dfrac{1}{a_{1} b_{2}-a_{2} b_{1}}\)

From this equation, we get two equations:

\(\begin{align} \dfrac{x}{b_{1} c_{2}-b_{2} c_{1}}&=\dfrac{1}{a_{1} b_{2}-a_{2} b_{1}} \\[0.2cm] \dfrac{y}{c_{1} a_{2}-c_{2} a_{1}}&=\dfrac{1}{a_{1} b_{2}-a_{2} b_{1}} \end{align}\)

Solving each of these for x and y, the solution of the given system is:

\(\begin{align} x&=\frac{b_{1} c_{2}-b_{2} c_{1}}{a_{1} b_{2}-a_{2} b_{1}}\\[0.2cm] y&=\frac{c_{1} a_{2}-c_{2} a_{1}}{a_{1} b_{2}-a_{2} b_{1}} \end{align}\)

Method of Elimination

To solve a system of linear equations in two variables using the elimination method , we will use the steps given below:

• Step 1: Arrange the equations in the standard form: ax+by+c=0 or ax+by=c.
• Step 2: Check if adding or subtracting the equations would result in the cancellation of a variable.
• Step 3: If not, multiply one or both equations by either the coefficient of x or y such that their addition or subtraction would result in the cancellation of any one of the variables.
• Step 4: Solve the resulting single variable equation.
• Step 5: Substitute it in any of the given equations to get the value of another variable.

Example: Solve the following system of equations using the elimination method. 2x+3y-11=0 3x+2y-9=0

Adding or subtracting these two equations would not result in the cancellation of any variable. Let us aim at the cancellation of x. The coefficients of x in both equations are 2 and 3. Their LCM is 6. We will make the coefficients of x in both equations 6 and -6 such that the x terms get canceled when we add the equations.

3 × (2x+3y-11=0) ⇒ 6x+9y-33=0 -2 × (3x+2y-9=0) ⇒ -6x-4y+18=0

Now we will add these two equations: 6x+9y-33=0 -6x-4y+18=0

On adding both the above equations we get, ⇒ 5y-15=0 ⇒ 5y=15 ⇒ y=3

Substitute this in one of the given two equations and solve the resultant variable for x. 2x+3y-11=0 ⇒ 2x+3(3)-11=0 ⇒ 2x+9-11=0 ⇒ 2x=2 ⇒ x=1

Therefore, the solution of the given system of equations is x=1 and y=3.

The determinant of a 2 × 2 matrix is obtained by cross-multiplying elements starting from the top left corner and subtracting the products.

Consider a system of linear equations in two variables: a 1 x + b 1 y = c 1 and a 2 x + b 2 y = c 2 . To solve them using the determinants method (which is also known as Crammer's Rule ), follow the steps given below:

• Step 1: We first find the determinant formed by the coefficients of x and y and label it Δ. Δ = \(\left|\begin{array}{ll}a_1 & b_1 \\a_2 & b_2\end{array}\right| = a_1 b_2 - a_2b_1\)
• Step 2: Then we find the determinant Δ x which is obtained by replacing the first column of Δ with constants. Δ x = \(\left|\begin{array}{ll}c_1 & b_1 \\c_2 & b_2\end{array}\right| = c_1 b_2 - c_2b_1\)
• Step 3: We then find the determinant Δ y which is obtained by replacing the second column of Δ with constants. Δ y = \(\left|\begin{array}{ll}a_1 & c_1 \\a_2 & c_2\end{array}\right| = a_1 c_2 - a_2c_1\)

Now, the solution of the given system of linear equations is obtained by the formulas:

• x = Δ x / Δ
• y = Δ y / Δ

Important Points on Linear Equations with Two Variables:

• A linear equation in two variables is of the form ax + by + c = 0, where x and y are variables; and a, b, and c are real numbers.
• A pair of linear equations are of the form a 1 x + b 1 y + c 1 = 0 and a 2 x + b 2 y + c 2 = 0 and its solution is a pair of values (x, y) that satisfy both equations.
• To solve linear equations in two variables, we must have at least two equations.
• A linear equation in two variables has infinitely many solutions.

Tricks and Tips:

While solving the equations using either the substitution method or the elimination method:

• If we get an equation that is true (i.e., something like 0 = 0, -1 = -1, etc), then it means that the system has an infinite number of solutions.
• If we get an equation that is false (i.e., something like 0 = 2, 3 = -1, etc), then it means that the system has no solution.

☛Related Topics:

• Solving Linear Equations Calculator
• Equation Calculator
• System of Equations Calculator
• Linear Graph Calculator

Linear Equations in Two Variables Examples

Example 1: The sum of the digits of a two-digit number is 8. When the digits are reversed, the number is increased by 18. Find the number.

Solution: Let us assume that x and y are the tens digit and the ones digit of the required number. Then the number is 10x+y.

And the number when the digits are reversed is 10y+x.

The question says, "The sum of the digits of a two-digit number is 8".

So from this, we get a linear equation in two variables: x+y=8.

Also, when the digits are reversed, the number is increased by 18.

So, the equation is 10y+x =10x+y+18

⇒ 10(8-x)+x =10x+(8-x)+18 (by substituting the value of y) ⇒ 80-10x+x =10x+8-x+18 ⇒ 80-9x=9x+26 ⇒ 18x = 54 ⇒ x=3

Substituting x=3 in y=8-x, we get, ⇒ y = 8-3 = 5 ⇒ 10x+y=10(3)+5 =35 Answer: The required number is 35.

Example 2: Jake's piggy bank has 11 coins (only quarters or dimes) that have a total value of $1.85. How many dimes and quarters does the piggy bank has?

Solution: Let us assume that the number of dimes be x and the number of quarters be y in the piggy bank. Let us form linear equations in two variables based on the given information.

Since there are 11 coins in total, x+y=11 ⇒ y=11-x. We know that, 1 dime = 10 cents and 1 quarter = 25 cents. The total value of the money in the piggy bank is $1.85 (185 cents).

Thus we get the equation 10x + 25y = 185 ⇒ 10x + 25(11-x) = 185 (as y = 11-x) ⇒ 10x + 275 - 25x =185 ⇒ -15x +275 =185 ⇒ -15x=-90 ⇒ x = 6

Substitute this value of x in x+y=11. ⇒ y=11-6=5

Answer: Therefore, the number of dimes is 6 and the number of quarters is 5.

Example 3: In a river, a boat can travel 30 miles upstream in 2 hours. The same boat can travel 51 miles downstream in 3 hours. Find,

• What is the speed of the boat in still water?
• What is the speed of the current?

Solution: Let us assume that:

• the speed of the boat in still water = x miles per hour
• the speed of current = y miles per hour.

During upstream, the current pulls back the boat's speed and the speed of the boat upstream = (x-y). During downstream, the current's speed adds to the boat's speed and the speed of the boat downstream = (x+y).

Using the last two columns of the table, we can form a pair of linear equations in two variables: x-y=15 x+y=17

Adding both equations we get: 2x = 32 ⇒ x=16

Substitute x=16 in x+y=17 16+y= 17 y=1

Answer: Therefore, the speed of the boat is 16 miles per hour and the speed of the current is 1 mile per hour.

go to slide go to slide go to slide

Book a Free Trial Class

Practice Questions on Linear Equations in Two Variables

go to slide go to slide

FAQs on Linear Equations in Two Variables

What is meant by linear equation in two variables.

A linear equation is an equation with degree 1. A linear equation in two variables is a type of linear equation in which there are 2 variables present. For example, 2x - y = 45, x+y =35, a-b = 45 etc.

How do you Identify Linear Equations in Two Variables?

We can identify a linear equation in two variables if it can be expressed in the form ax+by+ c = 0, consisting of two variables x and y and the highest degree of the given equation is 1.

Can You Solve a Pair of Linear Equations in Two Variables?

Yes, we can solve pair of linear equations in two variables using different methods and ensure there are two equations present in the given system of equations so as to obtain the values of variables. If there is one solution it means that the given lines are intersecting, if there is no solution possible, then it means that the given equations are of parallel lines. If there are infinitely many solutions possible, it means that the given equations are forming coincidental lines.

How to Graphically Represent a Pair of Linear Equations in Two Variables?

We can represent linear equations in two variables graphically using the steps given below:

• Step 1: A system of two equations in two variables can be solved graphically by graphing each equation by converting it to the form y=mx+b by solving the equation for y.
• Step 2: The points where both lines meet are identified.
• Step 3: The point of intersection is the solution of the given pair of linear equations in two variables.

How Does One Solve the System of Linear Equations in Two Variables?

We have different methods to solve the system of linear equations:

• Determinant or Matrix Method

How Many Solutions Does a Linear Equation with Two Variables Have?

Suppose we have a 1 x + b 1 y + c 1 = 0 and a 2 x + b 2 y + c 2 = 0. The solutions of a linear equation with two variables are:

• One and unique if a 1 /a 2 ≠ b 1 /b 2
• None if a 1 /a 2 = b 1 /b 2 ≠ c 1 /c 2
• Infinitely many if a 1 /a 2 = b 1 /b 2 = c 1 /c 2

How is a Linear Inequality in Two Variables like a Linear Equation in Two Variables?

A linear inequality in two variables and a linear equation in two variables have the following things in common:

• The degree of a linear equation and linear inequality is always 1.
• Both of them can be solved graphically.
• The way to solve a linear inequality is the same as linear equations except that it is separated by an inequality symbol. But note that the inequality rules should be taken care of.

Linear Equations Word Problems Part 2 Lesson

• Demonstrate an understanding of how to solve a Linear Equation in One Variable
• Demonstrate an understanding of the six-step process for solving word problems
• Demonstrate an understanding of how to check the solution for a word problem
• Learn how to set up and solve mixture word problems
• Learn how to set up and solve motion word problems

How to Solve Word Problems with Linear Equations

Six-step method for applications of linear equations in one variable.

• Write down the main objective of the problem
• If more than one unknown exists, we express the other unknowns in terms of this variable
• Write out an equation that describes the given situation
• Solve the equation
• State the answer using a nice clear sentence
• We need to make sure the answer is reasonable. In other words, if asked how many miles were driven to the store, the answer shouldn't be -300 as we can't drive a negative amount of miles.

Mixture Word Problems

Motion word problems, skills check:.

Solve each word problem.

Mary created a metal containing 70% platinum by combining 1 pound of pure platinum with 3 pounds of another metal. What percent of the other metal was platinum?

Please choose the best answer.

A cargo plane flew to New Castle and back. The trip there took 5.6 hours and the trip back took 7.2 hours. It averaged 73 mph faster on the trip there than on the return trip. What was the cargo plane's average speed on the outbound trip?

Betty left the movie theater and drove south at an average speed of 29 miles per hour. David left 2.2 hours later and drove in the same direction but with an average speed of 51 miles per hour. Find the number of hours Betty drove before David caught up.

Congrats, Your Score is 100 %

Better Luck Next Time, Your Score is %

Ready for more? Watch the Step by Step Video Lesson   |   Take the Practice Test

• + ACCUPLACER Mathematics
• + ACT Mathematics
• + AFOQT Mathematics
• + ALEKS Tests
• + ASVAB Mathematics
• + ATI TEAS Math Tests
• + Common Core Math
• + DAT Math Tests
• + FSA Tests
• + FTCE Math
• + GED Mathematics
• + Georgia Milestones Assessment
• + GRE Quantitative Reasoning
• + HiSET Math Exam
• + HSPT Math
• + ISEE Mathematics
• + PARCC Tests
• + Praxis Math
• + PSAT Math Tests
• + PSSA Tests
• + SAT Math Tests
• + SBAC Tests
• + SIFT Math
• + SSAT Math Tests
• + STAAR Tests
• + TABE Tests
• + TASC Math
• + TSI Mathematics
• + ACT Math Worksheets
• + Accuplacer Math Worksheets
• + AFOQT Math Worksheets
• + ALEKS Math Worksheets
• + ASVAB Math Worksheets
• + ATI TEAS 6 Math Worksheets
• + FTCE General Math Worksheets
• + GED Math Worksheets
• + 3rd Grade Mathematics Worksheets
• + 4th Grade Mathematics Worksheets
• + 5th Grade Mathematics Worksheets
• + 6th Grade Math Worksheets
• + 7th Grade Mathematics Worksheets
• + 8th Grade Mathematics Worksheets
• + 9th Grade Math Worksheets
• + HiSET Math Worksheets
• + HSPT Math Worksheets
• + ISEE Middle-Level Math Worksheets
• + PERT Math Worksheets
• + Praxis Math Worksheets
• + PSAT Math Worksheets
• + SAT Math Worksheets
• + SIFT Math Worksheets
• + SSAT Middle Level Math Worksheets
• + 7th Grade STAAR Math Worksheets
• + 8th Grade STAAR Math Worksheets
• + THEA Math Worksheets
• + TABE Math Worksheets
• + TASC Math Worksheets
• + TSI Math Worksheets
• + AFOQT Math Course
• + ALEKS Math Course
• + ASVAB Math Course
• + ATI TEAS 6 Math Course
• + CHSPE Math Course
• + FTCE General Knowledge Course
• + GED Math Course
• + HiSET Math Course
• + HSPT Math Course
• + ISEE Upper Level Math Course
• + SHSAT Math Course
• + SSAT Upper-Level Math Course
• + PERT Math Course
• + Praxis Core Math Course
• + SIFT Math Course
• + 8th Grade STAAR Math Course
• + TABE Math Course
• + TASC Math Course
• + TSI Math Course
• + Number Properties Puzzles
• + Algebra Puzzles
• + Geometry Puzzles
• + Intelligent Math Puzzles
• + Ratio, Proportion & Percentages Puzzles
• + Other Math Puzzles

How to Solve Word Problems by Finding Two-Variable Equations?

In this step-by-step guide, you will learn how to solve word problems by finding two-variable equations.

A step-by-step guide to solving word problems by finding two-variable equations

Here is a step-by-step guide on how to solve word problems by finding two-variable equations:

• Read the problem carefully: Begin by reading the problem carefully to understand what is being asked. Identify the relevant variables and their relationships to each other.
• Choose variables to represent the problem: Choose variables to represent the quantities involved in the problem. It’s common to use x and y as variables in two-variable equations, but other variables can also be used.
• Identify the known quantities: Identify the quantities in the problem that are known, such as given values or constraints.
• Identify the unknown quantities: Identify the quantities in the problem that are unknown, which are typically the values you are trying to solve for.
• Write a two-variable equation: Write a two-variable equation that relates the known and unknown quantities. This equation should represent the problem in a way that can be solved algebraically.
• Solve the equation: Use algebraic techniques to solve the equation for the unknown quantity. This may involve rearranging the equation, factoring, or using formulas.
• Check your answer: Once you have solved for the unknown quantity, check your answer by plugging it back into the original equation and verifying that it makes sense in the context of the problem.
• Interpret the solution: Finally, interpret the solution in the context of the problem. Make sure the answer makes sense and is reasonable given the known constraints and quantities.

Solving Word Problems by Finding Two-Variable Equations – Example 1

Solve the relationships in the word problem. There are \(8\) cookies in a pack. Let \(p\) represent the number of packs and \(c\) represent the number of cookies. Find the number of cookies when \(p=2\).

Look for relationships between the number of packs and cookies. Find c by multiplying the number of packs by cookies. So, \(8×2=16\) \(c=16\)

Solving Word Problems by Finding Two-Variable Equations – Example 2

Solve the relationships in the word problem. David rides the taxi for \(10\) minutes every day. \(d\) represents the number of days and \(m\) represents the total number of minutes David spends in the taxi. After two weeks how much time will he spend in the taxi?

Look for relationships between the number of days and minutes. He spends 10 minutes every day. To know the total time after two weeks (14 days), multiply time by days. So, \(10×14=140\) \(t=140\)

by: Effortless Math Team about 1 year ago (category: Articles )

Effortless Math Team

Related to this article, more math articles.

• Interwoven Variables: The World of Implicit Relations
• 6th Grade RICAS Math Worksheets: FREE & Printable
• Top Tablets for Online Math Teaching
• How to Prepare for the ISEE Lower Level Math Test?
• How to Unlock the Secrets: “SIFT Math for Beginners” Solution Manual
• How to Find Maxima and Minima of a Function?
• Convert Between Decimals and Mixed Numbers
• Decimals Demystified: From Standard Form to Expanded Form with Fractions
• 8th Grade IAR Math Worksheets: FREE & Printable
• How to Solve One-step and Two-step Linear Equations Word Problems

What people say about "How to Solve Word Problems by Finding Two-Variable Equations? - Effortless Math: We Help Students Learn to LOVE Mathematics"?

No one replied yet.

Leave a Reply Cancel reply

You must be logged in to post a comment.

Mastering Grade 6 Math Word Problems The Ultimate Guide to Tackling 6th Grade Math Word Problems

Mastering grade 5 math word problems the ultimate guide to tackling 5th grade math word problems, mastering grade 7 math word problems the ultimate guide to tackling 7th grade math word problems, mastering grade 2 math word problems the ultimate guide to tackling 2nd grade math word problems, mastering grade 8 math word problems the ultimate guide to tackling 8th grade math word problems, mastering grade 4 math word problems the ultimate guide to tackling 4th grade math word problems, mastering grade 3 math word problems the ultimate guide to tackling 3rd grade math word problems.

• ATI TEAS 6 Math
• ISEE Upper Level Math
• SSAT Upper-Level Math
• Praxis Core Math
• 8th Grade STAAR Math

Limited time only!

Save Over 45 %

It was $89.99 now it is $49.99

Login and use all of our services.

Effortless Math services are waiting for you. login faster!

Register Fast!

Password will be generated automatically and sent to your email.

After registration you can change your password if you want.

• Math Worksheets
• Math Courses
• Math Topics
• Math Puzzles
• Math eBooks
• GED Math Books
• HiSET Math Books
• ACT Math Books
• ISEE Math Books
• ACCUPLACER Books
• Premium Membership
• Youtube Videos

Effortless Math provides unofficial test prep products for a variety of tests and exams. All trademarks are property of their respective trademark owners.

• Bulk Orders
• Refund Policy

WORD PROBLEMS INVOLVING LINEAR EQUATIONS IN TWO VARIABLES

Problem 1 :

Raman’s age is three times he sum of the ages of his two sons. After 5 years his age will be twice the sum of the ages of his two sons. Find the age of Raman

Let x be Raman's age and y be the sum of ages of his two sons.

x  =  3y  -----(1)

After 5 years :

Raman's age  =  x + 5

The sum of the ages of his two sons  =  y + 5 + 5  

  =  y + 10

x + 5  =  2(y + 10)

x + 5  =  2y + 20

x - 2y  =  20 - 5

x - 2y  =  15 --------(2)

By applying the value of x in (2), we get

3y - 2y  =  15

y  =  15

x  =  3(15)

x  =  45

So, Raman is 45 years old.

Problem 2 :

The middle digit of a number between 100 and 1000 is zero and the sum of the other digit is 13. If the digits are reversed, the number so formed exceeds the original number by 495. Find the number

The required number will be in the form X 0 Y

Middle digit  =  0

x + y  =  13 -------(1)

Y 0 X  =  X0Y + 495

100y + x  =  100x + 1y + 495

x - 100x + 100y - y  =  495

 -99x + 99y  =  495

-x + y  =  5 -------(2)

x  =  y - 5

By applying the value of x in (1), we get

y - 5  + y  =  13

2y  =  13+5

2y  =  18

y  =  9

When y  =  9,

x  =  9 - 5

x  =  4

So, the required number is 409.

Kindly mail your feedback to   [email protected]

We always appreciate your feedback.

© All rights reserved. onlinemath4all.com

• Sat Math Practice
• SAT Math Worksheets
• PEMDAS Rule
• BODMAS rule
• GEMDAS Order of Operations
• Math Calculators
• Transformations of Functions
• Order of rotational symmetry
• Lines of symmetry
• Compound Angles
• Quantitative Aptitude Tricks
• Trigonometric ratio table
• Word Problems
• Times Table Shortcuts
• 10th CBSE solution
• PSAT Math Preparation
• Privacy Policy
• Laws of Exponents

Recent Articles

Mean Value Theorem

May 14, 24 02:48 AM

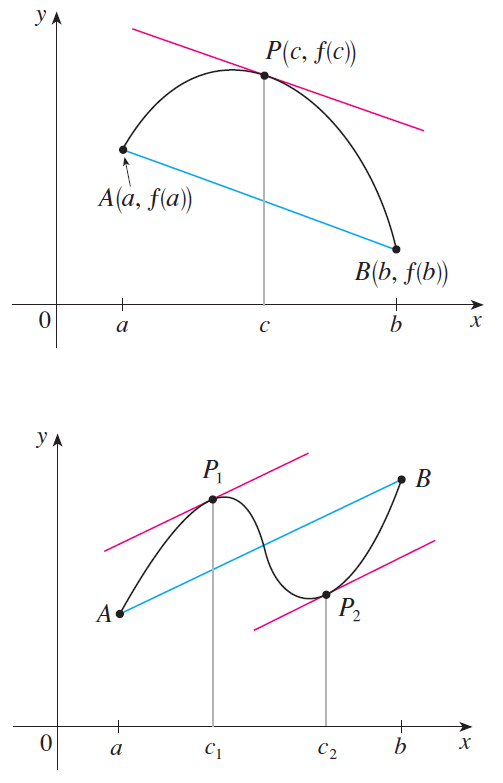
How to Find the Vertex of a Parabola

May 12, 24 10:03 PM

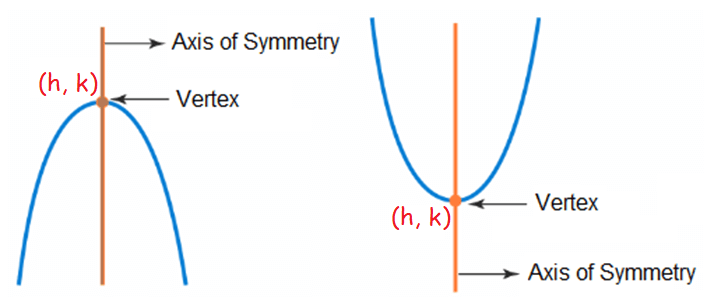
Converting Between Polar and Rectangular Coordinates

May 12, 24 01:14 PM

If you're seeing this message, it means we're having trouble loading external resources on our website.

If you're behind a web filter, please make sure that the domains *.kastatic.org and *.kasandbox.org are unblocked.

To log in and use all the features of Khan Academy, please enable JavaScript in your browser.

Course: Algebra 1   >   Unit 7

• Writing two-variable inequalities word problem
• Solving two-variable inequalities word problem
• Interpreting two-variable inequalities word problem

Two-variable inequalities word problems

• Modeling with systems of inequalities
• Writing systems of inequalities word problem
• Solving systems of inequalities word problem
• Graphs of systems of inequalities word problem
• Systems of inequalities word problems
• Graphs of two-variable inequalities word problem
• Inequalities (systems & graphs): FAQ
• Creativity break: What can we do to expand our creative skills?

SOLVING LINEAR INEQUALITIES WORD PROBLEMS IN TWO VARIABLES

A statement involving the symbols ‘>’, ‘<’, ‘ ≥’, ‘≤’ is called an inequality. 

By understanding the real situation, we have to use two variables to represent each quantities

Problem 1 :

Katie has $50 in a savings account at the beginning of the summer. She wants to have at least $20 in the account by the end of the summer. She withdraws $2 each week for food, clothes, and movie tickets. Write an inequality that expresses Katie’s situation and display it on the graph below. For how many weeks can Katie withdraw money?

Let x be the number of weeks 

50 - 2x  ≥  20

2x  ≤  30

x  ≤  15 weeks

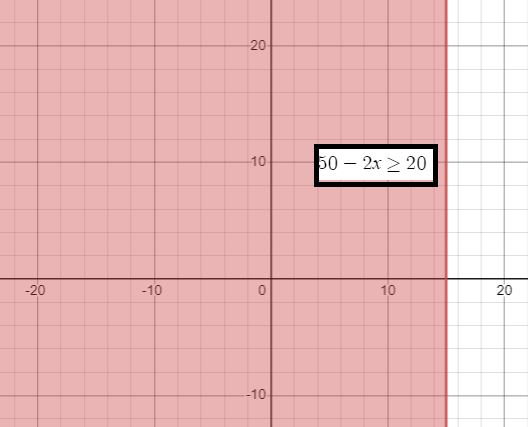
Problem 2 :

Skate Land charges a $50 flat fee for a birthday party rental and $4 for each person. Joann has no more than $100 to budget for her party. Write an inequality that models her situation and display it on the graph below. How many people can attend Joann's party.

Assume x people can attend the party.

y = 50 + 4x

50 + 4x ≤ 100

So, 12 people can attend Joan’s party.

Problem 3 :

Sarah is selling bracelets and earrings to make money for summer vacation. The bracelets cost $2 and the earrings cost $3. She needs to make at least $60. Sarah knows she will sell more than 10 bracelets. Write inequalities to represent the income from jewelry sold and number of bracelets sold. Find two possible solutions.

Let x be the number of bracelets sold.

Let y be the number of earrings sold.

2x + 3y ≥ 60

If x = 11, then 2(11) + 3y  ≥ 60

3y  ≥ 60 - 22

3y  ≥ 38

Since y is the number of earrings, x = 11 is not possible.

• Variables and Expressions
• Variables and Expressions Worksheet
• Place Value of Number
• Formation of Large Numbers
• Laws of Exponents
• Angle Bisector Theorem
• Pre Algebra
• SAT Practice Topic Wise
• Geometry Topics
• SMO Past papers
• Parallel Lines and Angles
• Properties of Quadrilaterals
• Circles and Theorems
• Transformations of 2D Shapes
• Quadratic Equations and Functions
• Composition of Functions
• Polynomials
• Fractions Decimals and Percentage
• Customary Unit and Metric Unit
• Honors Geometry
• 8th grade worksheets
• Linear Equations
• Precalculus Worksheets
• 7th Grade Math Worksheets
• Vocabulary of Triangles and Special right triangles
• 6th Grade Math Topics
• STAAR Math Practice
• Math 1 EOC Review Worksheets
• 11 Plus Math Papers
• CA Foundation Papers

Recent Articles

Factoring Exponential Expression Using Algebraic Identities Worksheet

Mar 14, 24 10:44 PM

Positive and Negative Numbers Connecting in Real Life Worksheet

Mar 14, 24 10:12 AM

Solver Title

Generating PDF...

• Pre Algebra Order of Operations Factors & Primes Fractions Long Arithmetic Decimals Exponents & Radicals Ratios & Proportions Percent Modulo Number Line Expanded Form Mean, Median & Mode
• Algebra Equations Inequalities System of Equations System of Inequalities Basic Operations Algebraic Properties Partial Fractions Polynomials Rational Expressions Sequences Power Sums Interval Notation Pi (Product) Notation Induction Logical Sets Word Problems
• Pre Calculus Equations Inequalities Scientific Calculator Scientific Notation Arithmetics Complex Numbers Polar/Cartesian Simultaneous Equations System of Inequalities Polynomials Rationales Functions Arithmetic & Comp. Coordinate Geometry Plane Geometry Solid Geometry Conic Sections Trigonometry
• Calculus Derivatives Derivative Applications Limits Integrals Integral Applications Integral Approximation Series ODE Multivariable Calculus Laplace Transform Taylor/Maclaurin Series Fourier Series Fourier Transform
• Functions Line Equations Functions Arithmetic & Comp. Conic Sections Transformation
• Linear Algebra Matrices Vectors
• Trigonometry Identities Proving Identities Trig Equations Trig Inequalities Evaluate Functions Simplify
• Statistics Mean Geometric Mean Quadratic Mean Average Median Mode Order Minimum Maximum Probability Mid-Range Range Standard Deviation Variance Lower Quartile Upper Quartile Interquartile Range Midhinge Standard Normal Distribution
• Physics Mechanics
• Chemistry Chemical Reactions Chemical Properties
• Finance Simple Interest Compound Interest Present Value Future Value
• Economics Point of Diminishing Return
• Conversions Roman Numerals Radical to Exponent Exponent to Radical To Fraction To Decimal To Mixed Number To Improper Fraction Radians to Degrees Degrees to Radians Hexadecimal Scientific Notation Distance Weight Time Volume
• Pre Algebra
• One-Step Addition
• One-Step Subtraction
• One-Step Multiplication
• One-Step Division
• One-Step Decimals
• Two-Step Integers
• Two-Step Add/Subtract
• Two-Step Multiply/Divide
• Two-Step Fractions
• Two-Step Decimals
• Multi-Step Integers
• Multi-Step with Parentheses
• Multi-Step Rational
• Multi-Step Fractions
• Multi-Step Decimals
• Solve by Factoring
• Completing the Square
• Quadratic Formula
• Biquadratic
• Logarithmic
• Exponential
• Rational Roots
• Floor/Ceiling
• Equation Given Roots
• Newton Raphson
• Substitution
• Elimination
• Cramer's Rule
• Gaussian Elimination
• System of Inequalities
• Perfect Squares
• Difference of Squares
• Difference of Cubes
• Sum of Cubes
• Polynomials
• Distributive Property
• FOIL method
• Perfect Cubes
• Binomial Expansion
• Negative Rule
• Product Rule
• Quotient Rule
• Expand Power Rule
• Fraction Exponent
• Exponent Rules
• Exponential Form
• Logarithmic Form
• Absolute Value
• Rational Number
• Powers of i
• Complex Form
• Partial Fractions
• Is Polynomial
• Leading Coefficient
• Leading Term
• Standard Form
• Complete the Square
• Synthetic Division
• Linear Factors
• Rationalize Denominator
• Rationalize Numerator
• Identify Type
• Convergence
• Interval Notation
• Pi (Product) Notation
• Boolean Algebra
• Truth Table
• Mutual Exclusive
• Cardinality
• Caretesian Product
• Age Problems
• Distance Problems
• Cost Problems
• Investment Problems
• Number Problems
• Percent Problems
• Addition/Subtraction
• Multiplication/Division
• Dice Problems
• Coin Problems
• Card Problems
• Pre Calculus
• Linear Algebra
• Trigonometry
• Conversions

Most Used Actions

Number line.

• \mathrm{Lauren's\:age\:is\:half\:of\:Joe's\:age.\:Emma\:is\:four\:years\:older\:than\:Joe.\:The\:sum\:of\:Lauren,\:Emma,\:and\:Joe's\:age\:is\:54.\:How\:old\:is\:Joe?}
• \mathrm{Kira\:went\:for\:a\:drive\:in\:her\:new\:car.\:She\:drove\:for\:142.5\:miles\:at\:a\:speed\:of\:57\:mph.\:For\:how\:many\:hours\:did\:she\:drive?}
• \mathrm{The\:sum\:of\:two\:numbers\:is\:249\:.\:Twice\:the\:larger\:number\:plus\:three\:times\:the\:smaller\:number\:is\:591\:.\:Find\:the\:numbers.}
• \mathrm{If\:2\:tacos\:and\:3\:drinks\:cost\:12\:and\:3\:tacos\:and\:2\:drinks\:cost\:13\:how\:much\:does\:a\:taco\:cost?}
• \mathrm{You\:deposit\:3000\:in\:an\:account\:earning\:2\%\:interest\:compounded\:monthly.\:How\:much\:will\:you\:have\:in\:the\:account\:in\:15\:years?}
• How do you solve word problems?
• To solve word problems start by reading the problem carefully and understanding what it's asking. Try underlining or highlighting key information, such as numbers and key words that indicate what operation is needed to perform. Translate the problem into mathematical expressions or equations, and use the information and equations generated to solve for the answer.
• How do you identify word problems in math?
• Word problems in math can be identified by the use of language that describes a situation or scenario. Word problems often use words and phrases which indicate that performing calculations is needed to find a solution. Additionally, word problems will often include specific information such as numbers, measurements, and units that needed to be used to solve the problem.
• Is there a calculator that can solve word problems?
• Symbolab is the best calculator for solving a wide range of word problems, including age problems, distance problems, cost problems, investments problems, number problems, and percent problems.
• What is an age problem?
• An age problem is a type of word problem in math that involves calculating the age of one or more people at a specific point in time. These problems often use phrases such as 'x years ago,' 'in y years,' or 'y years later,' which indicate that the problem is related to time and age.

word-problems-calculator

• High School Math Solutions – Systems of Equations Calculator, Elimination A system of equations is a collection of two or more equations with the same set of variables. In this blog post,...

Please add a message.

Message received. Thanks for the feedback.

Two-Variable Word Problems

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.